All ISEE Upper Level Quantitative Resources
Example Questions
Example Question #1 : How To Find The Radius Of A Sphere
The volume of a sphere is one cubic yard. Give its radius in inches.
Possible Answers:
Correct answer:
Explanation:
The volume 

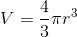
To find the radius in yards, we set 

![r = \sqrt[3]{ \frac{3}{4\pi} } = \sqrt[3]{ \frac{3\cdot 2 \pi^{2}}{4\pi \cdot 2 \pi^{2}} }= \sqrt[3]{ \frac{6 \pi^{2}}{8 \pi^{3}} } = \frac{ \sqrt[3]{6 \pi^{2}} } {2\pi}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/203839/gif.latex)
Since the problem requests the radius in inches, multiply by 36:
All ISEE Upper Level Quantitative Resources
Popular Subjects
English Tutors in Phoenix, Spanish Tutors in Dallas Fort Worth, Reading Tutors in Houston, GMAT Tutors in New York City, Computer Science Tutors in Washington DC, MCAT Tutors in San Diego, Chemistry Tutors in Miami, GRE Tutors in San Diego, Calculus Tutors in Atlanta, English Tutors in San Francisco-Bay Area
Popular Courses & Classes
GMAT Courses & Classes in San Diego, GMAT Courses & Classes in Atlanta, ACT Courses & Classes in New York City, SAT Courses & Classes in San Francisco-Bay Area, GMAT Courses & Classes in Denver, MCAT Courses & Classes in San Diego, SSAT Courses & Classes in San Diego, ISEE Courses & Classes in Los Angeles, ACT Courses & Classes in Miami, ACT Courses & Classes in Atlanta
Popular Test Prep
GRE Test Prep in Philadelphia, GRE Test Prep in Denver, LSAT Test Prep in Philadelphia, ACT Test Prep in New York City, SSAT Test Prep in San Francisco-Bay Area, ISEE Test Prep in San Francisco-Bay Area, SAT Test Prep in New York City, ISEE Test Prep in Los Angeles, GMAT Test Prep in San Francisco-Bay Area, SAT Test Prep in Miami


![\frac{ 18\sqrt[3]{6 \pi^{2}} } {\pi} \textrm{ in}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/203814/gif.latex)

![\frac{ 6\sqrt[3]{6 \pi^{2}} } {\pi} \textrm{ in}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/203816/gif.latex)
![\frac{ \sqrt[3]{6 \pi^{2}} } {2\pi}\textrm{ in}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/203815/gif.latex)


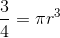


![36 \times \frac{ \sqrt[3]{6 \pi^{2}} } {2\pi} = \frac{ 18\sqrt[3]{6 \pi^{2}} } {\pi}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/203840/gif.latex)



