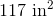Call Now to Set Up Tutoring:

(888) 888-0446

MAP 6th Grade Math : Geometry

Study concepts, example questions & explanations for MAP 6th Grade Math

Create An Account

All MAP 6th Grade Math Resources

5 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Geometry

What is the area of the right triangle in the following figure?

Possible Answers:

$58.5\textup{ in}^2$

$117\textup{ in}^2$

$80\textup{ in}^2$

$65.5\textup{ in}^2$

Correct answer:

$58.5\textup{ in}^2$

Explanation:

There are several different ways to solve for the area of a right triangle. In this lesson, we will transform the right triangle into a rectangle, use the the simpler formula for area of a rectangle to solve for the new figure's area, and divide this area in half in order to solve for the area of the original figure.

First, let's transform the triangle into a rectangle:

1 1

Second, let's remember that the formula for area of a rectangle is as follows:

$A=l\times w$

Substitute in our side lengths.

$A=13\times 9$

A=117

Last, notice that our triangle is exactly half the size of the rectangle that we made. This means that in order to solve for the area of the triangle we will need to take half of the area of the rectangle, or divide it by .

$117\div 2= 58.5\textup{ in}^2$

Thus, the area formula for a right triangle is as follows:

$A=\frac{1}{2}(l\times w)$ or $A=\frac{l\times w}{2}$

Report an Error

Example Question #2 : Geometry

What is the volume of the rectangular prism in the following figure?

Possible Answers:

$144\textup{ cm}^3$

$146.5\textup{ cm}^3$

$140\textup{ cm}^3$

$138.5\textup{ cm}^3$

Correct answer:

$140\textup{ cm}^3$

Explanation:

The formula used to find volume of a rectangular prism is as follows:

$A=l\times w\times h$

Substitute our side lengths:

$A=3\frac{1}{2}\times4\times10$

$A=140\textup{ cm}^3$

Remember, volume is always written with cubic units because volume is how many cubic units can fit inside of a figure.

Report an Error

Example Question #3 : Geometry

Hydraulic fracturing is a process used by gas companies to rupture and collects pockets of gas trapped within pockets of shale rock. A particular shale fracking site is $\frac{1}{6}\textup{ miles}$ in length and occupies an area of $\frac{5}{8}\textup{ miles}^2$ . How wide is this particular site?

Possible Answers:

$4\tfrac{1}{4}\textup{ miles}$

$3\tfrac{1}{3}\textup{ miles}$

$3\tfrac{3}{4}\textup{ miles}$

$3\tfrac{5}{7}\textup{ miles}$

Correct answer:

$3\tfrac{3}{4}\textup{ miles}$

Explanation:

In order to solve this question, we need to first recall how to find the area of a rectangle.

$\text{Area}=\text{Length} \times \text{Width}$

Substitute in the given values in the equation and solve for $\text{Width}$ .

$\frac{5}{8}\ miles^2=\frac{1}{6}\ miles\times Width$

Divide both sides by $\frac{1}{6}\ miles$

$\frac{\frac{5}{8}\ miles^2}{\frac{1}{6}\ miles}=\frac{\frac{1}{6}\ miles \times Width}{\frac{1}{6}\ miles}$

Dividing by a fraction is the same as multiplying by its inverse or reciprocal.

Find the reciprocal of $\frac{1}{6}\ miles$

$\frac{1}{6}\ miles\rightarrow \frac{6}{1}\ miles$

Simplify and rewrite.

$Width=\frac{6}{1} \times \frac{5}{8}$

Multiply and solve.

$Width=\frac{30}{8}$

Reduce.

$Width=3\tfrac{3}{4}\ miles$

The width of the fracking site is $3\tfrac{3}{4}\ miles$

Report an Error

View Tutors

Patricia
Certified Tutor

SUNY New Paltz, Bachelors, French. SUNY New Paltz, Masters, Secondary Education: French.

View Tutors

Anjali
Certified Tutor

Delhi University, Bachelor of Science, Physics. Kumuan University, Master of Science, Physics.

View Tutors

Pankaj
Certified Tutor

University of Delhi, Electrical Engineer, Electrical Engineering. Columbia University in the City of New York, Master of Arts...

All MAP 6th Grade Math Resources

5 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

ISEE Tutors in San Francisco-Bay Area, Reading Tutors in Los Angeles, Chemistry Tutors in Miami, Calculus Tutors in Washington DC, SSAT Tutors in Boston, Chemistry Tutors in San Francisco-Bay Area, Reading Tutors in Seattle, Spanish Tutors in San Diego, Reading Tutors in Chicago, Algebra Tutors in Chicago

Popular Courses & Classes

SAT Courses & Classes in Atlanta, ISEE Courses & Classes in Seattle, LSAT Courses & Classes in Boston, GRE Courses & Classes in Atlanta, GMAT Courses & Classes in Seattle, GMAT Courses & Classes in Denver, ISEE Courses & Classes in Dallas Fort Worth, GRE Courses & Classes in San Francisco-Bay Area, SSAT Courses & Classes in Washington DC, LSAT Courses & Classes in Seattle

Popular Test Prep

SSAT Test Prep in Phoenix, SSAT Test Prep in Atlanta, ISEE Test Prep in Los Angeles, GRE Test Prep in Philadelphia, ISEE Test Prep in Atlanta, ACT Test Prep in Miami, GMAT Test Prep in Seattle, ACT Test Prep in Washington DC, SSAT Test Prep in San Francisco-Bay Area, GRE Test Prep in Atlanta

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

MAP 6th Grade Math : Geometry

Study concepts, example questions & explanations for MAP 6th Grade Math

All MAP 6th Grade Math Resources

Example Questions

Example Question #1 : Geometry

Example Question #2 : Geometry

Example Question #3 : Geometry

All MAP 6th Grade Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: