Call Now to Set Up Tutoring:

(888) 888-0446

Precalculus : Find the Period of a Sine or Cosine Function

Study concepts, example questions & explanations for Precalculus

Create An Account

All Precalculus Resources

12 Diagnostic Tests 380 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Find The Period Of A Sine Or Cosine Function

Given $F(t)=sin\left(\frac{x}{2}-4\right)$ , what is the period for the function?

Possible Answers:

$\pi$

$2\pi$

$\frac{\pi}{2}$

$4\pi$

Correct answer:

$4\pi$

Explanation:

The formula for the period of a sine/cosine function is $\frac{2\pi}{|B|}$ .

With the standard form being:

Asin(Bx-C)+D

Since $B=\frac{1}{2}$ , the formula becomes $\frac{2\pi}{\frac{1}{2}}$ .

Simplified, the period is $4\pi$ .

Report an Error

Example Question #1 : Find The Period Of A Sine Or Cosine Function

What is the period of this graph?

3cos etc with pi and two pi

Possible Answers:

$\frac{2}{3}\pi$

$\pi$

$\frac{3}{2}\pi$

$2\pi$

Correct answer:

$2\pi$

Explanation:

One wave of the graph goes exactly from 0 to $2\pi$ before repeating itself. This means that the period is $2\pi$ .

Report an Error

Example Question #1 : Find The Period Of A Sine Or Cosine Function

Please choose the best answer from the following choices.

Find the period of the following function in radians:

y=cos(x)

Possible Answers:

$\pi$

$2\pi$

$\pi/2$

180

Correct answer:

$2\pi$

Explanation:

If you look at a graph, you can see that the period (length of one wave) is $2\pi$ . Without the graph, you can divide $2\pi$ with the frequency, which in this case, is 1.

Report an Error

Example Question #1 : Find The Period Of A Sine Or Cosine Function

Please choose the best answer from the following choices.

Find the period of the following function.

y=5sin(2x)

Possible Answers:

$2\pi$

$\pi$

$\pi/2$

Correct answer:

$\pi$

Explanation:

The period is defined as the length of one wave of the function. In this case, one full wave is 180 degrees or $\pi$ radians. You can figure this out without looking at a graph by dividing $2\pi$ with the frequency, which in this case, is 2.

Report an Error

Example Question #1 : Find The Period Of A Sine Or Cosine Function

What is the period of this sine graph?

Trig period 1

Possible Answers:

$\frac{3}{2}\pi$

$3\pi$

$\frac{2}{3}\pi$

$\pi^2$

Correct answer:

$\frac{2}{3}\pi$

Explanation:

The graph has 3 waves between 0 and $2\pi$ , meaning that the length of each of the waves is $2\pi$ divided by 3, or $\frac{2}{3}\pi$ .

Report an Error

Example Question #1 : Find The Period Of A Sine Or Cosine Function

Write the equation for a cosine graph with a minimum at $\left(\frac{ 3 \pi }{ 8 } , -5\right)$ and a maximum at $\left(\frac{ 11 \pi }{ 8 } , -1 \right)$ .

Possible Answers:

$y = 2 \cos (x - \frac {3 \pi }{ 8 }) - 3$

$y = 2 \cos (x + \frac{ 5 \pi }{ 8 }) - 3$

$y = 3 \cos (x - \frac{ 5 \pi }{ 8 }) - 2$

$y = -5 \cos (x + \frac{ 3 \pi }{8 })$

$y = 3 \cos (x - \frac{ 11 \pi }{8 }) - 2$

Correct answer:

$y = 2 \cos (x + \frac{ 5 \pi }{ 8 }) - 3$

Explanation:

The equation for this graph will be in the form $y = A \cos (f (x - h)) + k$ where A is the amplitude, f is the frequency, h is the horizontal shift, and k is the vertical shift.

To write this equation, it is helpful to sketch a graph:

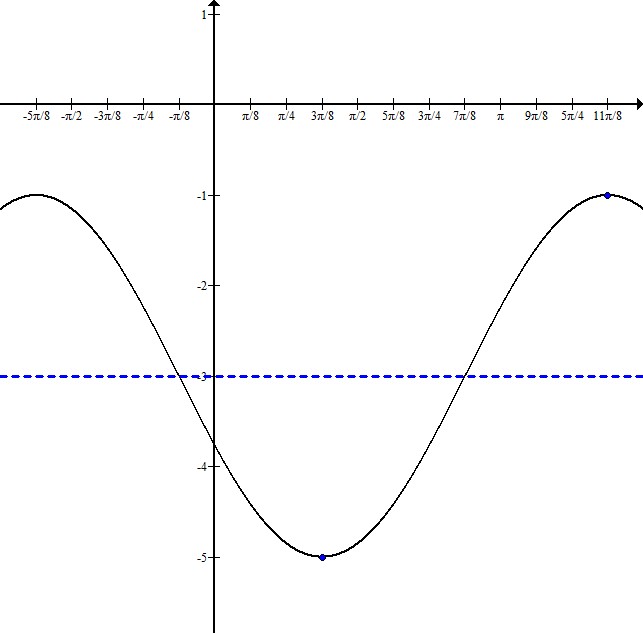
Trig graph 4

From sketching the maximum and the minimum, we can see that the graph is centered at y = -3 and has an amplitude of 2.

The distance between the maximum and the minimum is half the wavelength. Here, it is $\frac{ 11 \pi }{ 8 } - \frac{ 3 \pi }{ 8 } = \frac{ 8 \pi }{ 8 } = \pi$ . That means that the full wavelength is $2 \pi$ , so the frequency is 1.

The minimum occurs in the middle of the graph, so to figure out where it starts, subtract $\pi$ from the minimum's x-coordinate:

$\frac{ 3 \pi }{ 8 } - \pi = \frac{ 3 \pi }{8 } - \frac{ 8 \pi }{ 8 } = \frac{ -5 \pi }{8 }$

This graph's equation is

$y = 2 \cos (x + \frac{ 5 \pi }{8 }) -3$ .

Report an Error

Example Question #1 : Find The Period Of A Sine Or Cosine Function

Give the period and frequency for the equation $y = 4 \sin (6x - \pi ) + 2$ .

Possible Answers:

Period: $\pi$ , Frequency:

Period: $12 \pi$ , Frequency:

Period: $6 \pi$ , Frequency:

Period: $\frac{ \pi }{ 2 }$ , Frequency:

Period: $\frac{ \pi }{3}$ , Frequency:

Correct answer:

Period: $\frac{ \pi }{3}$ , Frequency:

Explanation:

Our equation is in the form $y = A \cdot \sin ( fx - h ) + k$

where A is the amplitude, f is the frequency, h is the horizontal shift, and k is the vertical shift.

We can look at the equation and see that the frequency, , is .

The period is $\frac{ 2 \pi }{f }$ , so in this case $\frac{ 2 \pi }{6} = \frac{ \pi }{3}$ .

Report an Error

Example Question #1 : Find The Period Of A Sine Or Cosine Function

What is the period of the graph $y = -2 \cos (\frac { \pi }{8 } x + 1 )$ ?

Possible Answers:

$\frac{ \pi }{ 8 }$

$\frac{ 8}{\pi }$

$\frac{ \pi }{4}$

Correct answer:

Explanation:

The equation for this function is in the form $y = A \cos (fx - h) + k$

where A is the amplitude, f is the frequency, h is the horizontal shift, and k is the vertical shift.

By looking at the equation, we can see that the frequency, , is $\frac{ \pi }{ 8 }$ .

The period is $\frac{ 2 \pi }{f }$ , so in this case $\frac{ 2 \pi }{ \frac{ \pi }{ 8 }} = 2 \pi \div \frac{ \pi }{ 8 } = \frac{ 2 \pi }{1} \cdot \frac{ 8}{\pi } = 16$ .

Report an Error

Example Question #9 : Find The Period Of A Sine Or Cosine Function

What could be the function for the following graph?

4sin

Possible Answers:

$y=-2\sin(x)+4$

$y=4\cos(x)+2$

$y=\sin(x)-2$

$y=4\sin(x)+2$

Correct answer:

$y=4\sin(x)+2$

Explanation:

What could be the function for the following graph?

4sin

Begin by realizing we are dealing with a periodic function, so sine and cosine are your best bet.

Next, note that the range of the function is $-2\leq y \leq 6$ and that the function goes through the point (0,2) .

From this information, we can find the amplitude:

$A=\frac{6--2}{2}=\frac{8}{2}=4$

So our function must have a out in front.

Also, from the point (0,2) , we can deduce that the function has a vertical translation of positive two.

The only remaining obstacle, is whether the function is sine or cosine. Recall that sine passes through (0,0) , while cosine passes through (0,1) . this means that our function must be a sine function, because in order to be a cosien graph, we would need a horizontal translation as well.

Thus, our answer is:

$y=4\sin(x)+2$

Report an Error

View Pre-Calculus Tutors

James
Certified Tutor

St. Mary of the Plains College, Bachelors, Mathematics. Baker University, Masters, Business Administration and Management.

View Pre-Calculus Tutors

Satweka
Certified Tutor

The University of Texas at Dallas, Master of Science, Biomedical Engineering.

View Pre-Calculus Tutors

Pankaj
Certified Tutor

University of Delhi, Electrical Engineer, Electrical Engineering. Columbia University in the City of New York, Master of Arts...

All Precalculus Resources

12 Diagnostic Tests 380 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Statistics Tutors in Denver, SSAT Tutors in San Francisco-Bay Area, SAT Tutors in Philadelphia, Computer Science Tutors in Phoenix, Chemistry Tutors in Chicago, Biology Tutors in San Diego, SSAT Tutors in Los Angeles, Physics Tutors in Denver, Physics Tutors in New York City, ACT Tutors in Atlanta

Popular Courses & Classes

LSAT Courses & Classes in Philadelphia, SAT Courses & Classes in Denver, ISEE Courses & Classes in Boston, SSAT Courses & Classes in Denver, SAT Courses & Classes in Philadelphia, GRE Courses & Classes in Phoenix, ISEE Courses & Classes in New York City, Spanish Courses & Classes in Chicago, SAT Courses & Classes in Chicago, ACT Courses & Classes in Seattle

Popular Test Prep

ISEE Test Prep in Atlanta, LSAT Test Prep in New York City, MCAT Test Prep in Houston, GRE Test Prep in Phoenix, SAT Test Prep in New York City, SAT Test Prep in Dallas Fort Worth, SSAT Test Prep in Philadelphia, LSAT Test Prep in San Francisco-Bay Area, GMAT Test Prep in Miami, ACT Test Prep in Seattle

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Precalculus : Find the Period of a Sine or Cosine Function

Study concepts, example questions & explanations for Precalculus

All Precalculus Resources

Example Questions

Example Question #1 : Find The Period Of A Sine Or Cosine Function

Example Question #1 : Find The Period Of A Sine Or Cosine Function

Example Question #1 : Find The Period Of A Sine Or Cosine Function

Example Question #1 : Find The Period Of A Sine Or Cosine Function

Example Question #1 : Find The Period Of A Sine Or Cosine Function

Example Question #1 : Find The Period Of A Sine Or Cosine Function

Example Question #1 : Find The Period Of A Sine Or Cosine Function

Example Question #1 : Find The Period Of A Sine Or Cosine Function

Example Question #9 : Find The Period Of A Sine Or Cosine Function

All Precalculus Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: