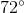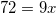ACT Math : Trapezoids
Study concepts, example questions & explanations for ACT Math
All ACT Math Resources
Example Questions
Example Question #51 : Quadrilaterals
If a trapezoid has a height of 14, base lengths of 10 and 12, and side lengths of 13, what is its perimeter?
Use the formula for perimeter of a trapezoid:
Where 
In this case:
Example Question #1 : How To Find An Angle In A Trapezoid
Find the measure of angle 
The sum of the angles in any quadrilateral is 360°, and the properties of an isosceles trapezoid dictate that the sets of angles adjoined by parallel lines (in this case, the bottom set and top set of angles) are equal. Subtracting 2(72°) from 360° gives the sum of the two top angles, and dividing the resulting 216° by 2 yields the measurement of x, which is 108°.
Example Question #2 : How To Find An Angle In A Trapezoid
Trapezoid 
As a rule, adjacent (non-paired) angles in a trapezoid are supplementary. Thus, we know that if 
Example Question #2 : How To Find An Angle In A Trapezoid
In the isosceles trapezoid above,
In degrees, what is the measure of 
To find the measure of angle DAC, we must know that the interior angles of all triangles sum up to 180 degrees. Also, as this is an isosceles trapezoid, 
Thus, 
Thus, 
Now that we know two angles out of the three in the triangle on the left, we can subtract them from 180 degrees to find 
Example Question #1 : How To Find An Angle In A Trapezoid
Given the following isosceles triangle:
In degrees, find the measure of the sum of 
All quadrilaterals' interior angles sum to 360°. In isosceles trapezoids, the two top angles are equal to each other.
Similarly, the two bottom angles are equal to each other as well.
Therefore, to find the sum of the two bottom angles, we subtract the measures of the top two angles from 360:
Example Question #1 : How To Find If Trapezoids Are Similar
Trapezoid A and Trapezoid B are similar. The bases of Trapezoid A are 
Because the two trapezoids are similar, the ratio of their bases must be the same. Therefore, we must set up a cross-multiplication to solve for the missing base:
Therefore, the length of the longer base of Trapezoid B is 
Example Question #1 : Trapezoids
What is the area of this regular trapezoid?
45
32
20
26
32
To solve this question, you must divide the trapezoid into a rectangle and two right triangles. Using the Pythagorean Theorem, you would calculate the height of the triangle which is 4. The dimensions of the rectangle are 5 and 4, hence the area will be 20. The base of the triangle is 3 and the height of the triangle is 4. The area of one triangle is 6. Hence the total area will be 20+6+6=32. If you forget to split the shape into a rectangle and TWO triangles, or if you add the dimensions of the trapezoid, you could arrive at 26 as your answer.
Example Question #1 : How To Find The Area Of A Trapezoid
What is the area of the trapezoid above if a = 2, b = 6, and h = 4?
32
8
24
16
64
16
Area of a Trapezoid = ½(a+b)*h
= ½ (2+6) * 4
= ½ (8) * 4
= 4 * 4 = 16
Example Question #2 : How To Find The Area Of A Trapezoid
Find the area of a trapezoid if the height is 
Write the formula to find the area of a trapezoid.
Substitute the givens and evaluate the area.
Example Question #1 : How To Find The Area Of A Trapezoid
Trapezoid 
The formula for the area of a trapezoid is:
We have here the height and one of the bases, plus the area, and we are being asked to find the length of base 
Thus,
All ACT Math Resources
