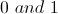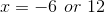All Algebra II Resources
Example Questions
Example Question #1 : Graphing Circle Functions
Find the 
To find the 


Because the left side of the equation is squared, it will always give us a positive answer. Thus if we want to take the root of both sides, we must account for this by setting up two scenarios, one where the value inside of the parentheses is positive and one where it is negative. This gives us the equations:

We can then solve these two equations to obtain 
Example Question #4 : Circle Functions
Find the 
To find the 


Because the left side of the equation is squared, it will always give us a positive answer. Thus if we want to take the root of both sides, we must account for this by setting up two scenarios, one where the value inside of the parentheses is positive and one where it is negative. This gives us the equations:

We can then solve these two equations to obtain
Example Question #51 : Quadratic Functions
What is the greatest possible value of the 
This equation describes a circle of radius 





Example Question #52 : Quadratic Functions
Find the 
Let
Therefore the equation becomes,
Solve for x.
Example Question #5 : Circle Functions
Find the 
Let
Therefore, the equation becomes:
Solve for y.
Example Question #3 : Circle Functions
A circle centered at 

What is the equation of the circle?
The equation for a circle centered at the point (h, k) with radius r units is

Setting 



Example Question #7 : Circle Functions
Convert the following angle to radians
To convert degrees to radians, multiply degrees by:
Therefore
Example Question #4 : Circle Functions
Find the negative coterminal of 160.
Coterminal angles are angles that are the same but written differently. Circles have 360 degrees, so an angle that goes above this threshold has completed one revolution. For example, a 450 degree angle would be in the same position as a 90 degree angle.
To find a positive coterminal angle, add 360 degrees to the initial value.
To find the negative coterminal angle, simply subtract 360. The only exception to this rule would be if the initial value were greater than 360. In this case, subtract 360 until the value is negative, making it a negative coterminal. Therefore,
160-360 = -200
Do this a second time and we get -560. These are examples of negative coterminal angles.
Example Question #9 : Circle Functions
Which of the following equations represent a circle?
The circle is represented by the formula:
Although some of the equations might not in this form, we can see by the variables that the equation 
Multiply two on both sides of the equation and we will have:
This is an equation of a circle. The other equations represent other conic shapes.
The answer is:
Example Question #451 : Functions And Graphs
Determine the equation of a circle that has radius 
None of these
Definition of the formula of a circle:
Where:





Plugging in values:
Certified Tutor
All Algebra II Resources