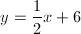(4,2) and (15,-4)

All that we really need to ascertain is the slope of our line.  So long as a given answer has this slope, it will not matter what its y-intercept is (given the openness of our question).  To find the slope, use the formula: m = rise / run = (y1 - y2) / (x1 - x2):

(2 - (-4)) / (4 - 15) = (2 + 4) / -11 = -6/11

Given this slope, our answer is: y = -6/11x + 57.4

By using the complementary and supplementary rules of geometry (due to lines m and n being parallel), as well as the fact that the sum of all angles within a triangle is 180, we can carry through the operations through stepwise subtraction of 180. 

x = 125 → angle directly below also = 125. Since a line is 180 degrees, 180 – 125 = 55. Since right triangle, 90 + 55 = 145 → rightmost angle of triangle 180 – 145 = 35 which is equal to the reflected angle. Use supplementary rule again for 180 – 35 = 145 = y.

Once can also recognize that both a straight line and triangle must sum up to 180 degrees to skip the last step.

To begin, solve the given equation for .  This will give you the slope-intercept form of the line.

Divide everything by :

Therefore, the slope of the line is .

Now, for a point , the point-slope form of a line is:

, where  is the slope

For our point, this is:

Distribute and solve for :

To begin, it is necessary to find the slope of the line running through the two points.  (A parallel line will have the same slope.   Recall that the slope is:

Or, for two points  and :

For our points this is:

Now, to solve for this problem, the easiest way is to solve each equation for the form .  When you do this, the slope () will be very easy to calculate.  The only option that reduces to the correct slope is 

Notice what happens when you solve for :

This shows that the slope of this line is .

Parallel lines have the same slope. Solve for the slope in the first line by converting the equation to slope-intercept form.

3x + 4y = 12

4y = –3x + 12

y = –(3/4)x + 3

slope = –3/4

We know that the second line will also have a slope of –3/4, and we are given the point (1,2). We can set up an equation in slope-intercept form and use these values to solve for the y-intercept.

y = mx + b

2 = –3/4(1) + b

2 = –3/4 + b

b = 2 + 3/4 = 2.75

Plug the y-intercept back into the equation to get our final answer.

y = –(3/4)x + 2.75

To solve, we will need to find the slope of the line. We know that it is parallel to the line given by the equation, meaning that the two lines will have equal slopes. Find the slope of the given line by converting the equation to slope-intercept form.

The slope of the line will be . In slope intercept-form, we know that the line will be . Now we can use the given point to find the y-intercept.

The final equation for the line will be .

Start by converting the original equation to slop-intercept form.

The slope of this line is . A parallel line will have the same slope. Now that we know the slope of our new line, we can use slope-intercept form and the given point to solve for the y-intercept.

Plug the y-intercept into the slope-intercept equation to get the final answer.

The line parallel to must have a slope of , giving us the equation . To solve for b, we can substitute the values for y and x.

Therefore, the equation of the line is .

Converting the given line to slope-intercept form we get the following equation:

For parallel lines, the slopes must be equal, so the slope of the new line must also be . We can plug the new slope and the given point into the slope-intercept form to solve for the y-intercept of the new line.

Use the y-intercept in the slope-intercept equation to find the final answer.

Find the slope of the given line:  (slope intercept form)

 therefore the slope is 

Parallel lines have the same slope, so now we need to find the equation of a line with slope  and going through point  by substituting values into the point-slope formula.

So, 

Thus, the new equation is