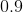GRE Math : Basic Squaring / Square Roots

Study concepts, example questions & explanations for GRE Math

Create An Account

All GRE Math Resources

13 Diagnostic Tests 452 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : How To Square A Decimal

Which quantity is greater? When 0<x<1

Quantity A

x^2

Quantity B

Possible Answers:

Quantity B is greater.

The relationship cannot be determined from the information given.

Quantity A is greater.

The two quantities are equal.

Correct answer:

Quantity B is greater.

Explanation:

X must equal a number between 0 and 1.

If you square any decimal number in this range you will get an answer less than 1. Try (0.999)^2 = 0.998.

Therefore Quantity B is always larger.

Report an Error

Example Question #1 : How To Square A Decimal

Which is greater, when $\small \small \small 0<x<1?$

Quantity A

$\small x^2$

Quantity B

$\small \frac{x}{2}$

Possible Answers:

Quantity B is greater

The two quantities are equal

Quantity A is greater

The relationship cannot be determined from the information given

Correct answer:

The relationship cannot be determined from the information given

Explanation:

When $\small 0<x<1$ , $\small x$ must be a decimal. Therefore it will decrease when it is squared. To find this answer we can substitute two values for $\small x$ .

$\small x = 0.9$ and $\small x = 0.1$

When $\small x = 0.9$

$\small \small 0.9^2 = 0.81 > \frac{0.9}{2} = 0.45$

But when $\small x = 0.1$

$\small 0.1^2 = 0.01 < \frac{0.1}{2} = 0.05$

Therefore we cannot tell which is greater based on the information given.

Report an Error

Example Question #22 : Decimals

Which is greater, when $\small \small 0<x<1?$

Quantity A

$\small \small x$

Quantity B

$\small 2x^2$

Possible Answers:

The relationship cannot be determined from the information given

Quantity A is greater

Quantity B is greater

The two quantities are equal

Correct answer:

The relationship cannot be determined from the information given

Explanation:

When $\small 0<x<1$ , $\small x$ must be a decimal. Therefore it will decrease when it is squared. To find this answer we can substitute two values for $\small x$ .

$\small x = 0.9$ and $\small x = 0.1$

When $\small x = 0.9$

$\small \small \small 2\cdot0.9^2 = 1.62 > 0.9$

But when $\small x = 0.1$

$\small \small 2\cdot0.1^2 = 0.02 < 0.1$

Therefore we cannot tell which is greater based on the information given.

Report an Error

Example Question #2 : How To Square A Decimal

Which is greater, when $\small -1<x<0?$

Quantity A

$\small \left | x\right |$

Quantity B

$\small x^2$

Possible Answers:

Quantity A is greater

The relationship cannot be determined from the information given

Quantity B is greater

The two quantities are equal

Correct answer:

Quantity A is greater

Explanation:

When $\small \small -1<x<0$ , $\small x$ must be a negative decimal. Therefore it will decrease when it is squared and become positive. The first quantity $\small \left | x\right |$ is the distance to 0 from $\small x$ , in other words the positive value of $\small x$ . The second quantity $\small x^2$ will always be positive, but because it is a decimal it will always be less than $\small x$ , if $\small x$ was a positive quantity. Since $\small \left | x\right |\equiv x$ , then $\small \left | x\right |$ wiill always be greater than $\small x^2$ .

Quantity A is greater.

Report an Error

Example Question #3 : Basic Squaring / Square Roots

Which is greater, when $\small \small -1<x<0?$

Quantity A

$\small x^2$

Quantity B

$\small 1$

Possible Answers:

Quantity B is greater

Quantity A is greater

The two quantities are equal

The relationship cannot be determined from the information given

Correct answer:

Quantity B is greater

Explanation:

When $\small \small -1<x<0$ , $\small x$ must be a negative decimal. Therefore it will decrease when it is squared and become positive. Because $\small x$ must be a decimal, $\small x^2$ will also be a decimal. Therefore $\small x^2$ will never be greater than 1.

Quantity B is greater.

Report an Error

Example Question #1 : How To Find The Square Root Of A Decimal

Find the square root of the following decimal:

$\sqrt{.00081}=$

Possible Answers:

0.028

0.09

0.9

0.009

Correct answer:

0.028

Explanation:

The easiest way to find the square root of a fraction is to convert it into scientific notation.

$\dpi{100} \small .00081 = 8.1 \times 10^{-4}$

The key is that the exponent in scientific notation has to be even for a square root because the square root of an exponent is diving it by two. The square root of 9 is 3, so the square root of 8.1 is a little bit less than 3, around 2.8

$\dpi{100} \small \sqrt{8.1 \times 10^{-4}} \approx 2.8 \times 10^{-2} \approx 0.028$

Report an Error

Example Question #2 : How To Find The Square Root Of A Decimal

Find the square root of the following decimal:

$\small \sqrt{0.0049}$

Possible Answers:

$\small 0.022$

$\small 0.07$

$\small 0.7$

$\small 0.007$

Correct answer:

$\small 0.07$

Explanation:

To find the square root of this decimal we convert it into scientific notation.

$\small 0.0049 = 49 \cdot10^{-4}$

Because $\small 10^{-4}$ has an even exponent, we can divide the exponenet by 2 to get its square root.

$\small \sqrt{0.0049} = \sqrt{49}\cdot\sqrt{10^{-4}} = 7\cdot10^{-2} = 0.07$

Report an Error

Example Question #2 : Basic Squaring / Square Roots

Find the square root of the following decimal:

$\small \sqrt{0.025}$

Possible Answers:

$\small 0.005$

$\small 0.625$

$\small 0.158$

$\small 0.05$

Correct answer:

$\small 0.158$

Explanation:

This problem can be solve more easily by rewriting the decimal into scientific notation.

$\small 0.025 = 2.5 \times 10^{-2}$

Because $\small 10^{-2}$ has an even exponent, we can take the square root of it by dividing it by 2. The square root of 4 is 2, and the square root of 1 is 1, so the square root of 2.5 is less than 2 and greater than 1.

$\small \sqrt{0.025} = \sqrt{2.5}\times \sqrt{10^{-2}} = 1.58\times 10^{-1} = 0.158$

Report an Error

Example Question #2 : How To Find The Square Root Of A Decimal

Find the square root of the following decimal:

$\small \sqrt{0.00036}$

Possible Answers:

$\small 0.013$

$\small 0.019$

$\small 0.006$

$\small 0.06$

Correct answer:

$\small 0.019$

Explanation:

This problem becomes much simpler if we rewrite the decimal in scientific notation

$\small 0.00036 = 3.6\times 10^{-4}$

Because $\small 10^{-4}$ has an even exponent, we can take its square root by dividing it by two. The square root of 4 is 2, and because 3.6 is a little smaller than 4, its square root is a little smaller than 2, around 1.9

$\small \sqrt{0.025} = \sqrt{3.6}\times \sqrt{10^{-4}} \approx 1.9\times 10^{-2} = 0.019$

Report an Error

Example Question #1 : Basic Squaring / Square Roots

Find the square root of the following decimal:

$\small \sqrt{0.00064}$

Possible Answers:

$\small 0.0253$

$\small 0.08$

$\small 0.8$

$\small 0.008$

Correct answer:

$\small 0.0253$

Explanation:

To find the square root of this decimal we convert it into scientific notation.

$\small \small 0.00064 = 6.4 \times10^{-4}$

Because $\small 10^{-4}$ has an even exponent, we can divide the exponenet by 2 to get its square root. The square root of 9 is 3, and the square root of 4 is two, so the square root of 6.4 is between 3 and 2, around 2.53

$\small \small \sqrt{0.00064} = \sqrt{6.4}\times\sqrt{10^{-4}} \approx 2.53 \times10^{-2} = 0.0253$

Report an Error

All GRE Math Resources

13 Diagnostic Tests 452 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

GRE Tutors in Washington DC, MCAT Tutors in San Francisco-Bay Area, English Tutors in New York City, Chemistry Tutors in Boston, ISEE Tutors in Atlanta, MCAT Tutors in Boston, Reading Tutors in Chicago, ACT Tutors in Phoenix, SAT Tutors in Boston, Biology Tutors in Chicago

Popular Courses & Classes

GMAT Courses & Classes in Boston, ACT Courses & Classes in Los Angeles, ACT Courses & Classes in Boston, SSAT Courses & Classes in San Diego, ISEE Courses & Classes in Los Angeles, LSAT Courses & Classes in Miami, GMAT Courses & Classes in Denver, GRE Courses & Classes in Miami, ISEE Courses & Classes in New York City, LSAT Courses & Classes in Philadelphia

Popular Test Prep

ISEE Test Prep in Seattle, ISEE Test Prep in Dallas Fort Worth, SAT Test Prep in Atlanta, SAT Test Prep in San Francisco-Bay Area, ISEE Test Prep in Los Angeles, SSAT Test Prep in Chicago, GMAT Test Prep in Boston, ACT Test Prep in Boston, ISEE Test Prep in Phoenix, MCAT Test Prep in Phoenix

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

Email address:
Your name:
Feedback:

GRE Math : Basic Squaring / Square Roots

Study concepts, example questions & explanations for GRE Math

All GRE Math Resources

Example Questions

Example Question #1 : How To Square A Decimal

Example Question #1 : How To Square A Decimal

Example Question #22 : Decimals

Example Question #2 : How To Square A Decimal

Example Question #3 : Basic Squaring / Square Roots

Example Question #1 : How To Find The Square Root Of A Decimal

Example Question #2 : How To Find The Square Root Of A Decimal

Example Question #2 : Basic Squaring / Square Roots

Example Question #2 : How To Find The Square Root Of A Decimal

Example Question #1 : Basic Squaring / Square Roots

All GRE Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details