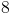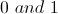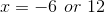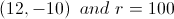All High School Math Resources
Example Questions
Example Question #1 : Understanding Circle Functions
Find the radius of the circle given by the equation:
To find the center or the radius of a circle, first put the equation in the standard form for a circle: 


From our equation, we see that it has not yet been factored, so we must do that now. We can use the formula 






Therefore, 
Because the constant, in this case 4, was not in the original equation, we need to add it to both sides:
Now we do the same for 
We can now find 
Example Question #2 : Understanding Circle Functions
Find the center of the circle given by the equation:
To find the center or the radius of a circle, first put the equation in standard form: 


From our equation, we see that it has not yet been factored, so we must do that now. We can use the formula 






This gives 
Because the constant, in this case 9, was not in the original equation, we must add it to both sides:
Now we do the same for 
We can now find the center: (3, -9)
Example Question #1 : Graphing Circle Functions
Find the 
To find the 


Because the left side of the equation is squared, it will always give us a positive answer. Thus if we want to take the root of both sides, we must account for this by setting up two scenarios, one where the value inside of the parentheses is positive and one where it is negative. This gives us the equations:

We can then solve these two equations to obtain 
Example Question #2 : Graphing Circle Functions
Find the 
To find the 


Because the left side of the equation is squared, it will always give us a positive answer. Thus if we want to take the root of both sides, we must account for this by setting up two scenarios, one where the value inside of the parentheses is positive and one where it is negative. This gives us the equations:

We can then solve these two equations to obtain
Example Question #1 : Quadratic Functions
Find the center and radius of the circle defined by the equation:
The equation of a circle is: 


In this problem, the equation is already in the format required to determine center and radius. To find the 



Example Question #2 : Quadratic Functions
Find the center and radius of the circle defined by the equation:
The equation of a circle is: 


In this problem, the equation is already in the format required to determine center and radius. To find the 





All High School Math Resources