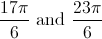We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

High School Math : Understanding Coterminal Angles

Study concepts, example questions & explanations for High School Math

Create An Account

All High School Math Resources

8 Diagnostic Tests 613 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #5 : Angles

Which of the following angles is coterminal with $\frac{2\pi}{7}$ ?

Possible Answers:

$-\frac{9\pi}{7}$

$\frac{12\pi}{7}$

$-\frac{2\pi}{7}$

$\frac{9\pi}{7}$

$-\frac{12\pi}{7}$

Correct answer:

$-\frac{12\pi}{7}$

Explanation:

For an angle to be coterminal with $\theta$ , that angle must be of the form $\theta + 2\pi N$ for some integer - or, equivalently, the difference of the angle measures multiplied by $\frac{1}{2\pi}$ must be an integer. We apply this test to all five choices.

$\frac{9\pi}{7}$ :

$\left ( \frac{1}{2\pi } \right ) \left ( \frac{2\pi}{7} - \frac{9\pi}{7} \right )$

$=\left ( \frac{1}{2\pi } \right )\left (- \frac{7\pi}{7} \right ) =\left ( \frac{1}{2\pi } \right ) \pi = \frac{1}{2 }$

$\frac{12\pi}{7}$ :

$\left ( \frac{1}{2\pi } \right ) \left ( \frac{2\pi}{7} - \frac{12\pi}{7} \right )$

$=\left ( \frac{1}{2\pi } \right )\left (- \frac{10\pi}{7} \right ) =- \frac{5}{7}$

$-\frac{2\pi}{7}$ :

$\left ( \frac{1}{2\pi } \right ) \left [\frac{2\pi}{7} -\left ( -\frac{2\pi}{7} \right ) \right ]$

$=\left ( \frac{1}{2\pi } \right )\left ( \frac{2\pi}{7} + \frac{2\pi}{7 }\right )$

$=\left ( \frac{1}{2\pi } \right )\left ( \frac{4\pi}{7} \right ) = \frac{2}{7}$

$-\frac{9\pi}{7}$ :

$\left ( \frac{1}{2\pi } \right ) \left [\frac{2\pi}{7} -\left ( -\frac{9\pi}{7} \right ) \right ]$

$=\left ( \frac{1}{2\pi } \right )\left ( \frac{2\pi}{7} + \frac{9\pi}{7 }\right )$

$=\left ( \frac{1}{2\pi } \right )\left ( \frac{11\pi}{7} \right ) = \frac{11}{14 }$

$-\frac{12\pi}{7}$ :

$\left ( \frac{1}{2\pi } \right ) \left [\frac{2\pi}{7} -\left ( -\frac{12\pi}{7} \right ) \right ]$

$=\left ( \frac{1}{2\pi } \right )\left ( \frac{2\pi}{7} + \frac{12\pi}{7 }\right )$

$=\left ( \frac{1}{2\pi } \right )\left ( \frac{14\pi}{7} \right ) = \left ( \frac{1}{2\pi } \right ) \cdot2\pi = 1$

$-\frac{12\pi}{7}$ is the correct choice, since only that choice passes our test.

Report an Error

Example Question #1 : Understanding Coterminal Angles

Find a coterminal angle for $-55^{\circ}$ .

Possible Answers:

$300^{\circ}$

$85^{\circ}$

$305^{\circ}$

$-15^{\circ}$

$360^{\circ}$

Correct answer:

$305^{\circ}$

Explanation:

Coterminal angles are angles that, when drawn in the standard position, share a terminal side. You can find these angles by adding or subtracting 360 to the given angle. Thus, the only angle measurement that works from the answers given is $305^{\circ}$ .

Report an Error

Example Question #22 : Graphing The Sine And Cosine Functions

Which of the following angles is coterminal with $\frac{5\pi}{9}$ ?

Possible Answers:

Each angle given in the other choices is coterminal with $\frac{5\pi}{9}$ .

$\frac{41\pi}{9}$

$-\frac{31\pi}{9}$

$\frac{23\pi}{9}$

$-\frac{13\pi}{9}$

Correct answer:

Each angle given in the other choices is coterminal with $\frac{5\pi}{9}$ .

Explanation:

$\frac{41\pi}{9}$ :

$\left ( \frac{1}{2\pi } \right ) \left (\frac{41\pi}{9}- \frac{5\pi}{9}\right )$

$=\left ( \frac{1}{2\pi } \right )\left ( \frac{36\pi}{9} \right ) =\left ( \frac{1}{2\pi } \right )4 \pi =2$

$\frac{23\pi}{9}$ :

$\left ( \frac{1}{2\pi } \right ) \left (\frac{23\pi}{9}- \frac{5\pi}{9}\right )$

$=\left ( \frac{1}{2\pi } \right )\left ( \frac{18\pi}{9} \right ) =\left ( \frac{1}{2\pi } \right )2 \pi =1$

$-\frac{13\pi}{9}$ :

$\left ( \frac{1}{2\pi } \right ) \left (-\frac{13\pi}{9}- \frac{5\pi}{9}\right )$

$=\left ( \frac{1}{2\pi } \right )\left ( \frac{-18\pi}{9} \right ) =\left ( \frac{1}{2\pi } \right )\left (- 2 \pi \right )=-1$

$-\frac{31\pi}{9}$ :

$\left ( \frac{1}{2\pi } \right ) \left (-\frac{31\pi}{9}- \frac{5\pi}{9}\right )$

$=\left ( \frac{1}{2\pi } \right )\left ( \frac{-36\pi}{9} \right ) =\left ( \frac{1}{2\pi } \right )\left (- 4 \pi \right )=-2$

All four choices pass the test, so all four angles are coterminal with $\frac{5\pi}{9}$ .

Report an Error

Example Question #3 : Understanding Coterminal Angles

$\textup{Which of the following angle measures, given in radians, is coterminal with an}$

$\textup{an angle of 90 degrees?}$

Possible Answers:

$-\frac{\pi }{2}$

$2\pi$

$\frac{5\pi }{2}$

$\frac{3\pi }{2}$

$\pi$

Correct answer:

$\frac{5\pi }{2}$

Explanation:

$90\textup{ degrees }=\frac{\pi }{2}\:\: \textup{ Coterminal angles share the same position on the unit circle.}$

$\textup{Therefore, coterminal angles can be found by adding or subtracting multiples of }2\pi$ .

$\frac{\pi }{2}+2\pi=\frac{5\pi }{2}$

Report an Error

Example Question #2 : Understanding Coterminal Angles

Which of the following choices represents a pair of coterminal angles?

Possible Answers:

$\frac{17\pi }{3} \textrm{ and } \frac{23\pi }{3}$

$\frac{17\pi }{5} \textrm{ and } \frac{23\pi }{5}$

$\frac{17\pi }{6} \textrm{ and } \frac{23\pi }{6}$

$\frac{17\pi }{4} \textrm{ and } \frac{23\pi }{4}$

$\frac{17\pi }{2} \textrm{ and } \frac{23\pi }{2}$

Correct answer:

$\frac{17\pi }{3} \textrm{ and } \frac{23\pi }{3}$

Explanation:

For two angles to be coterminal, they must differ by $2\pi N$ for some integer - or, equivalently, the difference of the angle measures multiplied by $\frac{1}{2\pi}$ must be an integer. We apply this test to all five choices.

$\frac{17\pi }{2} \textrm{ and } \frac{23\pi }{2}$ :

$\frac{1}{2\pi } \cdot \left ( \frac{23\pi }{2} - \frac{17\pi }{2} \right ) = \frac{1}{2\pi } \cdot \frac{6\pi }{2} = \frac{3 }{2}$

$\frac{17\pi }{3} \textrm{ and } \frac{23\pi }{3}$ :

$\frac{1}{2\pi } \cdot \left ( \frac{23\pi }{3} - \frac{17\pi }{3} \right ) = \frac{1}{2\pi } \cdot \frac{6\pi }{3} = 1$

$\frac{17\pi }{4} \textrm{ and } \frac{23\pi }{4}$ :

$\frac{1}{2\pi } \cdot \left ( \frac{23\pi }{4} - \frac{17\pi }{4} \right ) = \frac{1}{2\pi } \cdot \frac{6\pi }{4} = \frac{3 }{4}$

$\frac{17\pi }{5} \textrm{ and } \frac{23\pi }{5}$ :

$\frac{1}{2\pi } \cdot \left ( \frac{23\pi }{5} - \frac{17\pi }{5} \right ) = \frac{1}{2\pi } \cdot \frac{6\pi }{5} = \frac{3 }{5}$

$\frac{17\pi }{6} \textrm{ and } \frac{23\pi }{6}$ :

$\frac{1}{2\pi } \cdot \left ( \frac{23\pi }{6} - \frac{17\pi }{6} \right ) = \frac{1}{2\pi } \cdot \frac{6\pi }{6} = \frac{1 }{2}$

The only angles that pass the test - and are therefore coterminal - are $\frac{17\pi }{3} \textrm{ and } \frac{23\pi }{3}$ .

Report an Error

View Tutors

Aswathi
Certified Tutor

Kannur University , Master's/Graduate, English Language and Literature .

View Tutors

James
Certified Tutor

University of Georgia, Bachelor in Arts, Political Science and Government.

View Tutors

Onyinyechi
Certified Tutor

Imo State University, Nigeria, Bachelor in Arts, Mass Communications. Alabama A & M University, Masters in Education, Element...

All High School Math Resources

8 Diagnostic Tests 613 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Computer Science Tutors in New York City, ACT Tutors in Boston, LSAT Tutors in Seattle, Physics Tutors in Denver, GMAT Tutors in San Diego, Spanish Tutors in Atlanta, Statistics Tutors in Washington DC, GMAT Tutors in San Francisco-Bay Area, LSAT Tutors in Los Angeles, Algebra Tutors in San Francisco-Bay Area

Popular Courses & Classes

SSAT Courses & Classes in Chicago, SAT Courses & Classes in New York City, MCAT Courses & Classes in San Francisco-Bay Area, GRE Courses & Classes in Miami, Spanish Courses & Classes in Miami, SSAT Courses & Classes in Miami, ACT Courses & Classes in Philadelphia, ISEE Courses & Classes in Los Angeles, GRE Courses & Classes in Atlanta, ACT Courses & Classes in Seattle

Popular Test Prep

GRE Test Prep in New York City, SAT Test Prep in Miami, SAT Test Prep in Phoenix, LSAT Test Prep in Philadelphia, GRE Test Prep in San Francisco-Bay Area, ACT Test Prep in Seattle, MCAT Test Prep in Boston, ACT Test Prep in Philadelphia, GRE Test Prep in Houston, ACT Test Prep in Phoenix

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

High School Math : Understanding Coterminal Angles

Study concepts, example questions & explanations for High School Math

All High School Math Resources

Example Questions

Example Question #5 : Angles

Example Question #1 : Understanding Coterminal Angles

Example Question #22 : Graphing The Sine And Cosine Functions

Example Question #3 : Understanding Coterminal Angles

Example Question #2 : Understanding Coterminal Angles

All High School Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: