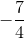Call Now to Set Up Tutoring:

(888) 888-0446

ACT Math : Lines

Study concepts, example questions & explanations for ACT Math

Create An Account

All ACT Math Resources

14 Diagnostic Tests 767 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Algebra

There is a circle on a coordinate plain. Its perimeter passes through the point (1,0)

. At this point meets a tangent line, which also passes through the point (5,7)

. What is the slope of the line perpindicular to this tangent line?

Possible Answers:

$\frac{7}{6}$

$\frac{4}{7}$

$\frac{7}{4}$

$-\frac{7}{4}$

$-\frac{4}{7}$

Correct answer:

$-\frac{4}{7}$

Explanation:

In this kind of problem, it's important to keep track of information given about your line of interest. In this case, the coordinates given set up the stage for us to be able to get to our line of focus - the line perpendicular to the tangent line. In order to determine the perpendicular line's slope, the tangent line's slope must be calculated. Keeping in mind that:

$slope =\frac{rise}{run}=\frac{\Delta y}{\Delta x} =\frac{y_2-y_1}{x_2-x_1}$

where y2,x2 and y1,x1 are assigned arbitrarily as long as the order of assignment is maintained.

$\frac{y_2-y_1}{x_2-x_1}=\frac{7-0}{5-1}=\frac{7}{4}$ which is the slope of the tangent line.

To calculate the perpendicular line, we have to remember that the product of the tangent slope and the perpendicular slope will equal -1.

$\frac{7}{4} x \frac{?}{?} = -1$ , the perpendicular slope can then be calculated as $\frac{-4}{7}$

Report an Error

Example Question #1 : Coordinate Plane

Find the slope of the tangent line to y=2x^2-16x+21 where x=2 .

Possible Answers:

Correct answer:

Explanation:

To find the slope of the tangent line, we must take the derivative.

By using the Power Rule we will be able to find the derivative:

$f(x)=a^n \rightarrow f'(x)=na^{n-1}$

Therefore derivative of 2x^2-16x+21 is 4x-16 .

Now we plug in x=2 , giving us 8-16=-8 .

Report an Error

Example Question #1 : Algebra

A line runs tangent to a circle at the point (4,2) . The line runs through the origin. Find the slope of the tangent line.

Possible Answers:

Cannot be determined

0.5

0.33

Correct answer:

0.5

Explanation:

The only two bits of information that are given for the tangent line is that it runs through the points (4,2) and (0,0) . With these two points, the line's slope can be easily calculated through the equation: $m=\frac{rise}{run}=\frac{\Delta y}{\Delta x}= \frac{y_{1}-y_{2}}{x_{1}-x_{2}}$

where is slope, is the -coordinate of the points, and is the -coordinates of the points.

Slope can be calculated through substituting in for the given values:

$m=\frac{2-0}{4-0}$

$m=\frac{2}{4}$

m= 0.5

Report an Error

Example Question #1 : How To Find The Equation Of A Tangent Line

Circle A is centered about the origin and has a radius of 5. What is the equation of the line that is tangent to Circle A at the point (–3,4)?

Possible Answers:

–3x + 4y = 1

3x – 4y = –1

3x + 4y = 7

3x – 4y = –25

Correct answer:

3x – 4y = –25

Explanation:

The line must be perpendicular to the radius at the point (–3,4). The slope of the radius is given by Actmath_7_113_q7

The radius has endpoints (–3,4) and the center of the circle (0,0), so its slope is –4/3.

The slope of the tangent line must be perpendicular to the slope of the radius, so the slope of the line is ¾.

The equation of the line is y – 4 = (3/4)(x – (–3))

Rearranging gives us: 3x – 4y = -25

Report an Error

Example Question #1 : How To Find The Equation Of A Tangent Line

Give the equation, in slope-intercept form, of the line tangent to the circle of the equation

$x^{2}+ y^{2} = 225$

at the point (12, -9) .

Possible Answers:

$y =-\frac{4}{3} x+7$

$y = \frac{4}{3} x- 25$

$y =- \frac{3} {4}x$

$y = \frac{3} {4}x-18$

None of the other responses gives the correct answer.

Correct answer:

$y = \frac{4}{3} x- 25$

Explanation:

The graph of the equation $x^{2}+ y^{2} = 225$ is a circle with center (0,0) .

A tangent to this circle at a given point is perpendicular to the radius to that point. The radius with endpoints (0,0) and (12, -9) will have slope

$\frac{ y_{2} - y_{1} }{x_{2} - x_{1} } =\frac{-9 -0}{12-0} =\frac{-9 }{12 }=- \frac{3}{4}$ ,

so the tangent line has the opposite of the reciprocal of this, or $\frac{4}{3}$ , as its slope.

The tangent line therefore has equation

$y - y_{1} = m (x-x_{1} )$

$y - (-9)= \frac{4}{3} (x-12 )$

$y +9= \frac{4}{3} x- 16$

$y = \frac{4}{3} x- 25$

Report an Error

Example Question #2 : Algebra

Give the equation, in slope-intercept form, of the line tangent to the circle of the equation

$x^{2}-6x + y^{2} - 91 = 0$

at the point (-3, 8) .

Possible Answers:

$y = \frac{4} {3}x+12$

$y =- \frac{3}{4} x+\frac{23}{4}$

None of the other responses gives the correct answer.

$y = \frac{3}{4} x+\frac{41}{4}$

$y =- \frac{4} {3}x+4$

Correct answer:

$y = \frac{3}{4} x+\frac{41}{4}$

Explanation:

Rewrite the equation of the circle in standard form to find its center:

$x^{2}-6x + y^{2} - 91 = 0$

$x^{2}-6x + y^{2} = 91$

Complete the square:

$x^{2}-6x+9 + y^{2} = 91+9$

$\left (x-3 \right )^{2} + y^{2} = 100$

The center is (3,0) .

A tangent to this circle at a given point is perpendicular to the radius to that point. The radius with endpoints (3,0) and (-3, 8) will have slope

$\frac{ y_{2} - y_{1} }{x_{2} - x_{1} } = \frac{8 -0}{-3-3} = \frac{8}{-6} = - \frac{4}{3}$ ,

so the tangent line has the opposite of the reciprocal of this, or $\frac{3}{4}$ , as its slope.

The tangent line therefore has equation

$y - 8= \frac{3}{4} (x- (-3))$

$y - 8= \frac{3}{4} (x+3)$

$y - 8= \frac{3}{4} x+\frac{9}{4}$

$y = \frac{3}{4} x+\frac{41}{4}$

Report an Error

Example Question #3 : How To Find The Equation Of A Tangent Line

What is the equation of a tangent line to

y=x^2+4x+2

at point (1,7) ?

Possible Answers:

y=3x+1

y=x+6

y=6x+1

y=4x+1

y=x^2+4x+2

Correct answer:

y=6x+1

Explanation:

To find an equation tangent to

y=x^2+4x+2

we need to find the first derviative of this equation with respect to to get the slope of the tangent line.

So,

y'=2x+4

due to power rule $y=x^n \rightarrow y'=nx^{n-1}$ .

First we need to find our slope by plugging our $x_{1}$ into the derivative equation and solving.

Thus, the slope is

$m=2x_{1}+4$

m=6 .

To find the equation of a tangent line of a given point $(x_{1},y_{1})$ we plug the point into

$y-y_{1}=m(x-x_{1})$ .

Therefore our equation becomes,

y-7=6(x-1)

Once we rearrange, the equation is

y=6x+1

Report an Error

Example Question #2 : Algebra

What is the tangent line equation of

y=x^4+4x

at point

(1,5) ?

Possible Answers:

y=4x^3+4

y=8x-3

y=8x+5

y=4

y=8x+8

Correct answer:

y=8x-3

Explanation:

To find an equation tangent to

y=x^4+4x

we need to find the first derviative of this equation with respect to to get the slope of the tangent line.

So,

y'=4x^3+4

due to power rule $y=ax^n \rightarrow y'=nax^{n-1}$ .

First we need to find the slope by plugging our $x_{1}$ into the derivative equation and solving.

Thus, the slope is

$m=4x_{1}^3+4$

m=8 .

To find the equation of a tangent line of a given point $(x_{1},y_{1})$ we plug it into

$y-y_{1}=m(x-x_{1})$ .

Therefore our equation is

y-5=8(x-1)

Once we rearrange, the equation is

y=8x-3

Report an Error

Example Question #4 : Lines

Find the equation of a tangent line to

y=x^2

for the point

(0,0) ?

Possible Answers:

y=2

y=2x

y=0

y=1

y=x^2

Correct answer:

y=0

Explanation:

To find an equation tangent to

y=x^2

we need to find the first derviative of this equation with respect to to get the slope of the tangent line.

So,

y'=2x

due to power rule $y=x^n \rightarrow y'=nx^{n-1}$ .

First we need to find the slope by plugging in our $x_{1}$ into the derivative equation and solving.

Thus, the slope is

$m=2x_{1}$

m=0 .

To find the equation of a tangent line of a given point $(x_{1},y_{1})$ we plug our point into

$y-y_{1}=m(x-x_{1})$ .

Therefore our equation is

y-0=0(x-0)

Once we rearrange, the equation is

y=0

Report an Error

Example Question #3 : How To Find The Equation Of A Tangent Line

What is the equation of a tangent line to

y=5x^5+2

at the point

(1,7) ?

Possible Answers:

y=27x

y=25x-18

y=18x-25

y=25x^4

y=25x^4+2

Correct answer:

y=25x-18

Explanation:

To find an equation tangent to

y=5x^5+2

we need to find the first derviative of this equation with respect to to get the slope of the tangent line.

So,

y'=25x^4

due to power rule $y=ax^n \rightarrow y'=nax^{n-1}$ .

First we need to find our slope by plugging in our $x_{1}$ into the derivative equation and solving.

Thus, the slope is

$m=25x_{1}^4$

m=25 .

To find the equation of a tangent line of a given point $(x_{1},y_{1})$ we plug the point into

$y-y_{1}=m(x-x_{1})$ .

Therefore our equation is

y-7=25(x-1)

Once we rearrange, the equation is

y=25x-18

Report an Error

View ACT Math Tutors

Joy
Certified Tutor

Boston University, Bachelor in Arts, Conservation Biology.

View ACT Math Tutors

Robert
Certified Tutor

Daytona State College, Bachelor in Arts, Teaching English as a Second Language (ESL).

View ACT Math Tutors

Obianuju
Certified Tutor

University of Miami, Bachelor of Science, Biomedical Engineering.

All ACT Math Resources

14 Diagnostic Tests 767 Practice Tests Question of the Day Flashcards Learn by Concept

ACT Math Tutors in Top Cities:

Atlanta ACT Math Tutors, Austin ACT Math Tutors, Boston ACT Math Tutors, Chicago ACT Math Tutors, Dallas Fort Worth ACT Math Tutors, Denver ACT Math Tutors, Houston ACT Math Tutors, Kansas City ACT Math Tutors, Los Angeles ACT Math Tutors, Miami ACT Math Tutors, New York City ACT Math Tutors, Philadelphia ACT Math Tutors, Phoenix ACT Math Tutors, San Diego ACT Math Tutors, San Francisco-Bay Area ACT Math Tutors, Seattle ACT Math Tutors, St. Louis ACT Math Tutors, Tucson ACT Math Tutors, Washington DC ACT Math Tutors

Popular Courses & Classes

GRE Courses & Classes in Seattle, GMAT Courses & Classes in San Francisco-Bay Area, ACT Courses & Classes in Phoenix, SSAT Courses & Classes in New York City, SSAT Courses & Classes in Washington DC, GRE Courses & Classes in Chicago, MCAT Courses & Classes in Los Angeles, SAT Courses & Classes in San Diego, GRE Courses & Classes in San Francisco-Bay Area, ACT Courses & Classes in Philadelphia

Popular Test Prep

ISEE Test Prep in Houston, LSAT Test Prep in Seattle, ACT Test Prep in Phoenix, SAT Test Prep in Denver, GRE Test Prep in San Diego, GRE Test Prep in Seattle, ISEE Test Prep in San Francisco-Bay Area, LSAT Test Prep in Los Angeles, SSAT Test Prep in Phoenix, SAT Test Prep in Chicago

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

ACT Math : Lines

Study concepts, example questions & explanations for ACT Math

All ACT Math Resources

Example Questions

Example Question #1 : Algebra

Example Question #1 : Coordinate Plane

Example Question #1 : Algebra

Example Question #1 : How To Find The Equation Of A Tangent Line

Example Question #1 : How To Find The Equation Of A Tangent Line

Example Question #2 : Algebra

Example Question #3 : How To Find The Equation Of A Tangent Line

Example Question #2 : Algebra

Example Question #4 : Lines

Example Question #3 : How To Find The Equation Of A Tangent Line

All ACT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: