All Algebra II Resources
Example Questions
Example Question #1 : Graphing Logarithmic Functions
Give the 
to two decimal places.
The graph has no 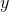
Set 
The 

Example Question #1 : Graphing Logarithmic Functions
Give the 
to two decimal places.
The graph has no 
Set 
The 

Example Question #2 : Graphing Logarithmic Functions
What is/are the asymptote(s) of the graph of the function 


The graph of the logarithmic function
has as its only asymptote the vertical line
Here, since 

Example Question #3 : Graphing Logarithmic Functions
Which is true about the graph of

All of the answers are correct
When 

The range of the function is infinite in both directions positive and negative.
The domain of the function is greater than zero
None of the answers are correct
All of the answers are correct
There is no real number 
Therefore in the equation 

However, 
Finally, when 

Example Question #4 : Graphing Logarithmic Functions
Which of the following is true about the graph of
The range must be greater than zero.
It is an odd function.
The graph is the mirror image of 
The domain is infinite in both directions.
It is an even function.
The graph is the mirror image of 


Example Question #227 : Logarithms
Give the equation of the horizontal asymptote of the graph of the equation

The graph of 
The graph of 
Let
In terms of 
This is the graph of 
The graph of 

Example Question #81 : Solving And Graphing Logarithms
Find the equation of the vertical asymptote of the graph of the equation

Let 


The graph of 








Certified Tutor
Certified Tutor
All Algebra II Resources













































