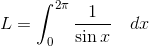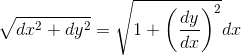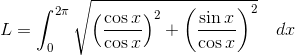We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

Calculus 1 : Length of Line

Study concepts, example questions & explanations for Calculus 1

Create An Account

All Calculus 1 Resources

10 Diagnostic Tests 438 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Length Of Line

Consider a function with first-derivative:

$\frac{dy}{dx}=\tan{x}$ .

Which integral can calculate the length along this curve between x=0 and $x=2\pi$ ?

Possible Answers:

$L=\int_{0}^{2\pi}{\frac{1}{\cos{x}}}\quad dx$

$L=\int_{0}^{2\pi}{\tan^2{x}}\quad dx$

$L=\int_{0}^{2\pi}{\frac{1}{\sin{x}}}\quad dx$

$L=\int_{0}^{2\pi}{\sqrt{1+\sin^2}}\quad dx$

This is not possible with the information given.

Correct answer:

$L=\int_{0}^{2\pi}{\frac{1}{\cos{x}}}\quad dx$

Explanation:

To determine the length of a curve between two points, we evaluate the integral

$L = \int_0^{2\pi}{\sqrt{1+\left(\frac{dy}{dx} \right )^2}}dx$

There are many reasons this works, but we'll give an informal explanation here: Canvas2

If we divide this curve into three line-segments, we can see that they become more and more similar to the original curve. By adding up all the little hypotenuses, we can arrive at the length of the curve. Note, that

$\sqrt{dx^2 + dy^2} = \sqrt{1+\left(\frac{dy}{dx} \right )^2}dx$

If we think of the integration symbol as a sum of infinitely small parts, this gets us the formula for length:

$L = \int_a^b{\sqrt{1+\left(\frac{dy}{dx} \right )^2}}dx$ .

Returning to the problem, we plug the derivative into the length formula:

$L=\int_{0}^{2\pi}{\sqrt{1+\tan^2{x}}}\quad dx$

substitute:

$L=\int_{0}^{2\pi}{\sqrt{\left( \frac{\cos{x}}{\cos{x}} \right)^2+\left(\frac{\sin{x}}{\cos{x}} \right )^2}}\quad dx$

Simplify:

$L=\int_{0}^{2\pi}{\sqrt{\frac{\cos^2{x}}{\cos^2{x}}+\frac{\sin^2{x}}{\cos^2{x}}}}\quad dx$

$L=\int_{0}^{2\pi}{\sqrt{\frac{\cos^2{x}+\sin^2{x}}{\cos^2{x}}}}\quad dx$

By trigonometric identities we get:

$L=\int_{0}^{2\pi}{\sqrt{\frac{1}{\cos^2{x}}}}\quad dx$

$L=\int_{0}^{2\pi}{\frac{1}{\cos{x}}}\quad dx$

Report an Error

Example Question #2 : Length Of Line

Find the length of the line segment between points A and B:

Possible Answers:

$3\sqrt{2}$

$\sqrt{58}$

$\sqrt{338}$

Correct answer:

$\sqrt{338}$

Explanation:

The distance between two points can be easily found using the Distance Formula:

$D=\sqrt{(x_1-x_2)^2+(y_1-y_2)^2}$

Applying the points we are given to this formula results in:

$D=\sqrt{((-5)-2)^2+(10-(-7))^2}=\sqrt{(-7)^2+(17)^2}=\sqrt{338}$

This is one of the answer choices.

Report an Error

View Calculus Tutors

Shane
Certified Tutor

The University of Texas at Dallas, Bachelor of Science, Computer Engineering, General.

View Calculus Tutors

Molly
Certified Tutor

Cornell University, Bachelor of Science, Biological and Physical Sciences. Cornell University, Bachelor of Science, Biochemis...

View Calculus Tutors

Saarim
Certified Tutor

St Edwards University, Bachelor of Science, Mathematics.

All Calculus 1 Resources

10 Diagnostic Tests 438 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Biology Tutors in Philadelphia, Chemistry Tutors in Phoenix, Computer Science Tutors in Chicago, Chemistry Tutors in Miami, Spanish Tutors in Denver, ACT Tutors in Philadelphia, Biology Tutors in New York City, Physics Tutors in Phoenix, Spanish Tutors in San Francisco-Bay Area, Physics Tutors in Chicago

Popular Courses & Classes

SSAT Courses & Classes in New York City, SAT Courses & Classes in San Francisco-Bay Area, MCAT Courses & Classes in Chicago, ISEE Courses & Classes in Philadelphia, GMAT Courses & Classes in Chicago, LSAT Courses & Classes in Houston, LSAT Courses & Classes in Miami, MCAT Courses & Classes in Seattle, ISEE Courses & Classes in Seattle, Spanish Courses & Classes in Washington DC

Popular Test Prep

ACT Test Prep in Denver, GRE Test Prep in Boston, GMAT Test Prep in San Francisco-Bay Area, MCAT Test Prep in Washington DC, LSAT Test Prep in Atlanta, SSAT Test Prep in Houston, LSAT Test Prep in Miami, GRE Test Prep in Philadelphia, SSAT Test Prep in Miami, SAT Test Prep in San Diego

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Calculus 1 : Length of Line

Study concepts, example questions & explanations for Calculus 1

All Calculus 1 Resources

Example Questions

Example Question #1 : Length Of Line

Example Question #2 : Length Of Line

Find the length of the line segment between points A and B:

All Calculus 1 Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: