All Calculus 1 Resources
Example Questions
Example Question #1 : How To Find Integral Expressions
What is the area under the curve 

By normal exponent rules 

which integrates to:
Example Question #2 : How To Find Integral Expressions
The Gaussian integral formula states that
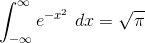
What is the integral of

Integrating by parts with




we get:
Example Question #3 : Equations
Determine 

This problem requires you to evaluate an indefinite integral of the given function f’(x). Integrating each term of the function with respect to x, we simply divide each coefficient by (n+1), where n is the value of the exponent on x for that particular term, and then add 1 to the value of the exponent on each x. This gives us:
A correct answer must include a constant C, as the original function may have had a constant that is not reflected in the equation for its derivative.
Example Question #2 : Equations
What is the value of the following definite integral?
We start by integrating the function in parentheses with respect to x, and we then subtract the evaluation of the lower limit from the evaluation of the upper limit:
Example Question #3 : How To Find Integral Expressions
Determine the amount of work required to push a box from 

This problem intends to demonstrate one possible application of calculus to the field of physics. An equation for work as given in physics is as follows:
Using this equation, we simply set up a definite integral with our bounds given by the interval of interest in the problem:

After evaluating our integral, we can see that the work required to push the box from x=2 meters to x=5 meters is 39.8 Joules.
Example Question #4 : How To Find Integral Expressions
Evaluate the definite integral within the interval 
In order to solve this problem we must know that

In this case we have:
Our first step is to integrate:

We then arrive to our solution by plugging in our values

Example Question #1 : Integral Expressions
Write out the expression of the area under the following curve from 

Simply use an integral expression with the given x values as your bounds.
Don't forget to add the dx!
Example Question #2 : Integral Expressions
Evaluate the definite integral within the interval 
In order to solve this problem we must remember that:
In this case we have:
Our first step is to integrate:
We then arrive at our solution by plugging in our values
Example Question #6 : How To Find Integral Expressions
Evaluate the definite integral within the interval
In order to solve this problem we must remember that:
In this case we have:
Our first step is to integrate:
We then arrive to our solution by plugging in our values
Example Question #3 : Equations
Evaluate the indefinite integral.
We are being asked to integrate the function.
To do this we need to remember the power rule of integrals,
Using this rule, we can evaluate the following integral:

Certified Tutor
Certified Tutor
All Calculus 1 Resources


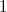
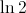


![\left[ \frac{e^{x \ln 2}}{\ln 2}\right]_{-\infty}^0 = \frac{2^0 - 2^{-\infty}}{\ln 2} = \frac{1 - 0}{\ln 2}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/126268/gif.latex)



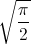

![\int_{-\infty}^{\infty} x^2 e^{-x^2} dx = \int_A^B u ~ dv = \left[ u v\right ]_A^B - \int_A^B v ~ du](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/129650/gif.latex)
![= \left[ \frac{-x}{2} e^{-x^2} \right ]_{-\infty}^{\infty} - \int_{-\infty}^{\infty} \frac{-1}{2} e^{-x^2}~ dx = [0 - 0] - \frac{-1}{2} \sqrt{\pi} = \frac{\sqrt{\pi}}{2}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/105514/gif.latex)

































![\left [ (1)^{5}+\frac{1}3{(1)^{3}-\frac{1}{2}(1)^{2}} \right ]-\left [ (0)^{5}+\frac{1}{3}(0)^{3}-\frac{1}{2}(0)^{2} \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/277086/gif.latex)















![\left[\frac{1}{2}(2)^4+\frac{1}{6}(2)^3+7(2)\right]-\left[\frac{1}{2}(1)^4+\frac{1}{6}(1)^3+7(1)\right]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/279458/gif.latex)











![\left [ 2\sqrt{9}+(9)^{3}-4(9) \right ]-\left [ 2\sqrt{4}+(4)^{3}-4(4) \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/279464/gif.latex)











