All GED Math Resources
Example Questions
Example Question #1 : Complementary Angles
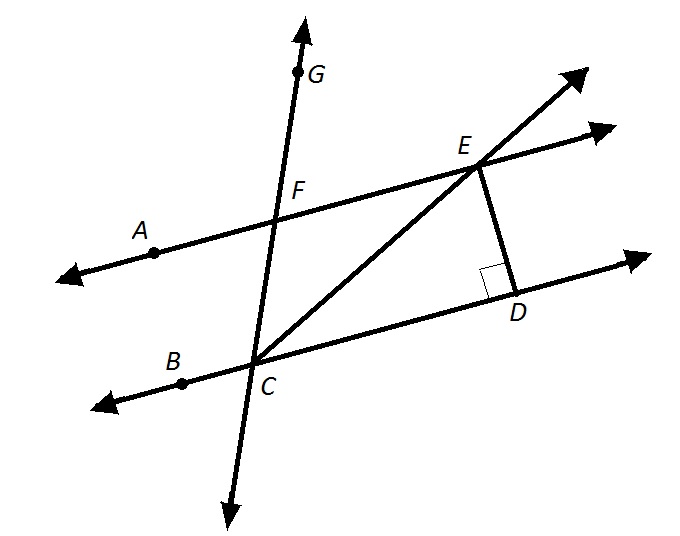
Refer to the above figure. You are given that 
Which two angles must be complementary?
















Example Question #2 : Complementary Angles
Angles A and B are complementary angles. The measure of angle A is 


Since angles A and B are complementary, their measures add up to 90 degrees. Therefore we can set up our equation as such:
-or-
Combine like terms and solve for 
Example Question #2 : Complementary Angles
What is the measure of an angle that is complementary to an angle measuring 
The sum of complementary angles is equal to 
Set up the following equation and solve for 
Example Question #3 : Complementary Angles
Angles A and B are complementary angles. The measure of angle A is 


Since angles A and B are complementary angles, their measurements add up to equal 90. Therefore, we need to set up our equation as follows:
-or-
Combine like terms:
And solve for 
Example Question #3 : Complementary Angles
Angles A and B are complementary angles. The measure of angle A is 

No solution
Since angles A and B are complementary, their measures add up to equal 90 degrees. Therefore we can set up an equation as follows:
-or-
Combine like terms and solve for 
Now that we have found 
Example Question #1 : Angle Geometry
What angle is complementary to 55?
The definition of a complementary angle means that two angles must add up to 90 degrees.
To find the angle, simply subtract 55 from 90.
The answer is:
Example Question #1 : Complementary Angles
What angle is complementary to 10 degrees?
Complementary angles must add up to ninety.
Subtract the given angle from 90 to find the other angle.
The answer is:
Example Question #5 : Angle Geometry
Suppose the angles 


Set up the equation such that both angles sum up to 90.
Add 5 on both sides.
Divide both sides by 8.
The value of 
The answer is:
Example Question #2 : Angle Geometry
Determine the value of 


Complementary angles must add up to 90 degrees.
Set up an equation so that the sum of two angles add up to 90.
Subtract 8 on both sides.
Simplify both sides.
Divide by two on both sides.
The answer is:
Example Question #10 : Complementary Angles
What angle is complementary to 
Note that this is in radians. Recall that 2 complementary angles must add up to 90 degrees.
Since 
To find the complementary angle, subtract 

The answer is:
All GED Math Resources




































































































