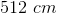36

A square has four equal sides and its area = side². Therefore, you can find the side length by taking the square root of the area √81 = 9. Then, find the perimeter by multiplying the side length by 4:

4 * 9 = 36 

The center of an inscribed square lies on the center of the circle. Thus, the line joining the center to a vertex of the square is also the radius. If we join the center with two adjacent vertices we can create a 45-45-90 right isosceles triangle, where the diagonal of the square is the hypotenuse. Since the radius is 2, the hypotenuse (a side of the square) must be  .

Finally, the perimeter of a square is . 

We know that our area can be represented by the following equation:

Instead of solving the algebra, you should immediately note several things.  16 = 4² and 4 = 2². If the side of the square is 4, then s = 4 would work out as:

 which is just what we need.

With s = 4, we know that 3 sides of our figure will have a perimeter of 12.  The remaining semicircle will be one half of the circumference of a circle with diameter of 4; therefore it will be 0.5 * 4 * π or 2π.

Therefore, the outer perimeter of our figure is 12 + 2π.

We start by writing the equations for the area and perimeter in terms of a side of length s.

Then, substitute both of these expressions into the given equation to solve for side length.

Finally, since four sides make up the perimeter, we substitue s back into our perimeter equation and solve for P.

First we must convert to inches.

The diagonal of a square divides the square into two isosceles-right triangles. Using the Pythagorean Theorem, we know that , where x is equal to the length of one side of the square.

This gives us .

Therefore, the perimeter of square  is equal to .

In order to solve this, we must first find the area of the containing square and then remove the inscribed circle.  Once this is done, we need to divide our result by 4 in order to get the one-forth that is the one shaded region.

One side of the square will be equal to the circle's diameter (2r).  Since r = 5, d = 10.  Therefore, the area of the square is d² = 10² = 100.  The area of the circle is πr² = 5²π = 25π.

Therefore, the area of the four "corner regions" is equal to 100 - 25π.  One of these is equal to (100 - 25π) / 4.  Simplified, this is 25 - (25/4)π.

All sides are equal in a square. To find the area of a square, multiply length times width. We know length = 4 but since all sides are equal, the width is also 4. 4 * 4 = 16.

To compare, first calculate the area of figure 1.  Since it shares dimensions with the semi-circle, we will put all our variables in terms of the radius of that semi-circle:

A₁ = (2r)² + πr²/2 = 4r² + πr²/2 = r²(4 + π)/2

If we double r, we get:

A₂ = (2 * 2r)² + π(2r)²/2 = 16r² + π4r²/2 = 4r²(4 + π)/2

This means that the new figure is 4x the size of the original.  This is an increase of 300%.

The obvious answer for this problem is that they are equal.  Remember quantitative comparisons are often tricky and require you to check your initial inclination. If 1 m equals 100 cm then a square with side 1 m (100 cm) has an area of 100 cm x 100 cm or 10,000 cm². The area of a square with a 1 cm side is 1 cm x 1 cm or 1 cm². One hundred times 1 cm² is 100 cm². 10,000 cm² is larger than 100 cm² so Quantity A is greater.

Since the diameter of the circle is 10, we know the radius of the circle is 5 feet. We can then draw radii that go from the center to two consecutive corners of the square. These radii are both 5 feet, and form a 90 degree angle (since they are diagonals of a square). Thus, with the enclosed side of the square they form a 45-45-90 triangle. Thus, the side of the square must be  (this can also be found with the Pythagorean theorem). Then, the area of the square is