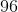All ISEE Upper Level Math Resources
Example Questions
Example Question #1 : How To Find The Greatest Common Factor
What is the greatest common factor of 72 and 80?
Find the factors of 72 and 80; the greatest common factor is the greatest number in both lists.
Example Question #1 : Numbers And Operations
What is the greatest common factor of 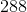

Fully factor 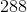


Multiple the common factors to find the greatest common factor:
Example Question #1 : Factors / Multiples
Which of these numbers is relatively prime with 10?
We are looking for a number that shares no factors with 10 other than 1. 20 and 28 can be eliminated since 2 divides 10, 20, 28. 25 and 35 can be eliminated since 5 divides 10, 25, and 25.
21 is the correct choice; 


Example Question #3 : Factors / Multiples
Which of these numbers is relatively prime with 
For a number 







We can eliminate 








Example Question #5 : Greatest Common Factor
Find the greatest common factor of 20 and 36.
To find the greatest common factor (GCF), you need to determine the factor that both numbers share that is of the greatest value. List the factors of each number and identify the largest number in value that is in both lists:
The GCF of 20 and 36 is 4 since it is the largest number in value that shows up in both lists.
Example Question #6 : Greatest Common Factor
What is the greatest common factor of 15, 30, and 40?
To find the greatest common factor (GCF), you need to determine the factor that all three numbers share that is of the greatest value. List the factors of each number and identify the largest number in value that is in all three lists:
The GCF of 15, 30, and 40 is 5 since it is the largest number in value that shows up in all three lists.
Example Question #1 : Factors / Multiples
What is the greatest common factor of 

Factor each number:

Identify the common factors (
Example Question #1 : How To Find The Greatest Common Factor
What is the greastest common factor of 

The greatest common factor of two numbers is the largest shared factor that the two numbers have in common.
The factors of 36 are
The factors of 108 are
The largest shared factor between 36 and 108 is 36. Therefore, 36 is the correct answer.
Example Question #2 : How To Find The Greatest Common Factor
Find the greatest common factor (GCF) of 56 and 64.
Find the greatest common factor (GCF) of 56 and 64.
To find the GCF, first find the prime factors of our two numbers:
Now, to find our GCF, we need to find the prime factors our two numbers have in common.
In this case, we have three 2's in common. In other words, we have an 8 in common. So, our GCF is 8.
Example Question #1 : How To Find The Greatest Common Factor
Find the greatest common factor (GCF) of 324 and 56.
Find the greatest common factor (GCF) of 324 and 56.
The GCF is the largest number which will can be evenly divided from either number.
To find the GCF, first find the prime factors of both numbers. A good trick here is to start by pulling a two out of each number until you cannot pull out a two anymore.
Beginning with 56
So the prime factorization of 56 looks like:
Next, let's do 324.
So for 324 we get:
Now, the GCF can be found by taking the prime factors which are common to both numbers. Now, in this case, we have two 2's in each number, and nothing else. This means that the GCF of 56 and 324 is
So our answer is 4
All ISEE Upper Level Math Resources