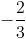We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

SSAT Upper Level Math : How to find the equation of a line

Study concepts, example questions & explanations for SSAT Upper Level Math

Create An Account

All SSAT Upper Level Math Resources

6 Diagnostic Tests 311 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Equations Of Lines

Give the equation of a line that passes through the point (4,7) and has slope 1.

Possible Answers:

x + y = 11

x = 4

x-y = 3

x-y = -3

y = 7

Correct answer:

x-y = -3

Explanation:

We can use the point slope form of a line, substituting $m = 1, x_{1} = 4, y _{1} = 7$ .

$y- y_{1} = m (x-x_{1})$

y- 7 = 1 (x-4)

y- 7 = x-4

y- 7 -y +4 = x-4 -y +4

-3 = x-y

x-y = -3

Report an Error

Example Question #2 : Equations Of Lines

A line can be represented by 2x+3y=6 . What is the slope of the line that is perpendicular to it?

Possible Answers:

$\frac{3}{4}$

$\frac{2}{3}$

$\frac{3}{2}$

$-\frac{2}{3}$

Correct answer:

$\frac{3}{2}$

Explanation:

You will first solve for Y, to get the equation in y=mx+b form.

represents the slope of the line, which would be $-\frac{2}{3}$ .

A perpendicular line's slope would be the negative reciprocal of that value, which is $\frac{3}{2}$ .

Report an Error

Example Question #3 : How To Find The Equation Of A Line

Find the equation the line goes through the points (3,4) and (0,2) .

Possible Answers:

$y=-\frac{3}{2}x+2$

$y=\frac{3}{2}x+2$

$y=\frac{2}{3}x+2$

$y=-\frac{2}{3}x+2$

Correct answer:

$y=\frac{2}{3}x+2$

Explanation:

First, find the slope of the line.

$\text{Slope}=\frac{y_2-y_1}{x_2-x_1}=\frac{2-4}{0-3}=\frac{-2}{-3}=\frac{2}{3}$

Now, because the problem tells us that the line goes through (0,2) , our y-intercept must be .

Putting the pieces together, we get the following equation:

$y=\frac{2}{3}x+2$

Report an Error

Example Question #3 : Equations Of Lines

A line passes through the points (-4, 6) and (-2, -2) . Find the equation of this line.

Possible Answers:

y=-4x-10

$y=\frac{1}{4}x-8$

$y=-\frac{1}{4}x+10$

y=4x+10

Correct answer:

y=-4x-10

Explanation:

To find the equation of a line, we need to first find the slope.

$\text{Slope}=\frac{y_2-y_1}{x_2-x_1}=\frac{-2-6}{-2-(-4)}=\frac{-8}{2}=-4$

Now, our equation for the line looks like the following:

y=-4x+b

To find the y-intercept, plug in one of the given points and solve for . Using (-4, 6) , we get the following equation:

6=-4(-4)+b

Solve for .

6=16+b

b=-10

Now, plug the value for into the equation.

y=-4x-10

Report an Error

Example Question #4 : Equations Of Lines

What is the equation of a line that passes through the points (8,1) and (-2, -7) ?

Possible Answers:

$y=\frac{5}{4}x+\frac{32}{5}$

$y=-\frac{4}{5}x+\frac{27}{5}$

$y=\frac{4}{5}x-\frac{27}{5}$

$y=\frac{27}{5}x-\frac{4}{5}$

Correct answer:

$y=\frac{4}{5}x-\frac{27}{5}$

Explanation:

First, we need to find the slope of the line.

$\text{Slope}=\frac{y_2-y_1}{x_2-x_1}=\frac{-7-1}{-2-8}=\frac{-8}{-10}=\frac{4}{5}$

Next, find the -intercept. To find the -intercept, plug in the values of one point into the equation y=mx+b , where is the slope that we just found and is the -intercept.

$1=8(\frac{4}{5})+b$

Solve for .

$1=\frac{32}{5}+b$

$b=-\frac{27}{5}$

Now, put the slope and -intercept together to get $y=\frac{4}{5}x-\frac{27}{5}$

Report an Error

Example Question #4 : Equations Of Lines

Lines

Examine the above diagram. What is x + y ?

Possible Answers:

125

145

135

Correct answer:

125

Explanation:

Use the properties of angle addition:

$\left ( x - 13\right )+ (y + 25) +43= 180$

x + y + 25+43- 13= 180

x + y + 55= 180

x + y + 55-55= 180-55

x + y = 125

Report an Error

Example Question #5 : Equations Of Lines

Are the following two equations parallel?

y=2x+13

5y=10x+95

Possible Answers:

Yes

Correct answer:

Yes

Explanation:

When two lines are parallal, they must have the same slope.

Look at the equations when they are in slope-intercept form, y=mx+b where b represents the slope.

We must first reduce the second equation since all of the constants are divisible by .

This leaves us with y=2x+19 . Since both equations have a slope of , they are parallel.

Report an Error

Example Question #192 : Algebra

Reduce the following expression:

$\frac{10a^{3}b^{5}c^{-2}}{2a^{2}}$

Possible Answers:

$\frac{5ab^{3}}{c^{2}}$

$10a^{1.5}b^{5}c^{2}$

$\frac{5a^{5}b^{5}}{c^{2}}$

$10a^{1.5}b^{-5}c^{2}$

$\frac{5ab^{5}}{c^{2}}$

Correct answer:

$\frac{5ab^{5}}{c^{2}}$

Explanation:

For this expression, you must take each variable and deal with them separately.

First divide you two constants 10/2=5 .

Then you move onto and when you divide like exponents you must subtract the exponents leaving you with .

is left by itself since it is already in a natural position.

Whenever you have a negative exponential term, you must it in the denominator.

This leaves the expression of $\frac{5ab^{5}}{c^{2}}$ .

Report an Error

Example Question #6 : Equations Of Lines

Give the equation of a line that passes through the point (4,7) and has an undefined slope.

Possible Answers:

y = 4

y = 7

x + y = 11

x = 4

x = 7

Correct answer:

x = 4

Explanation:

A line with an undefined slope has equation x = a for some number ; since this line passes through a point with -coordinate 4, then this line must have equation x = 4

Report an Error

Example Question #7 : How To Find The Equation Of A Line

Give the equation of the line through (2,9) and (8,1) .

Possible Answers:

4x-3y=29

3x-4y=20

4x+3y=35

-3x+4y=30

3x+4y=28

Correct answer:

4x+3y=35

Explanation:

First, find the slope:

$m=\frac{y_{2}-y_{1}}{x_{2}-x_{1}} =\frac{9-1}{2-8}=\frac{8}{-6}=-\frac{4}{3}$

Apply the point-slope formula:

$y-y_{1}= m (x-x_{1})$

$y-1= -\frac{4}{3} (x-8)$

$y-1= -\frac{4}{3} x- \left (-\frac{4}{3} \right )8$

$y-1= -\frac{4}{3} x+ \frac{32}{3}$

$y= -\frac{4}{3} x+ \frac{35}{3}$

Rewriting in standard form:

$\frac{4}{3} x + y= \frac{35}{3}$

4x+3y=35

Report an Error

View SSAT- Upper Level Tutors

Omar
Certified Tutor

Arizona State University, Bachelors, Civil Engineering.

View SSAT- Upper Level Tutors

Carneta
Certified Tutor

Langston University, Bachelor in Arts, Theater Arts.

View SSAT- Upper Level Tutors

Pamela
Certified Tutor

University of Colorado Boulder, Bachelor of Science, International Marketing.

All SSAT Upper Level Math Resources

6 Diagnostic Tests 311 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

French Tutors in Dallas Fort Worth, MCAT Tutors in Denver, ACT Tutors in Dallas Fort Worth, English Tutors in Atlanta, Spanish Tutors in Chicago, GRE Tutors in Atlanta, MCAT Tutors in Philadelphia, ACT Tutors in Los Angeles, Math Tutors in San Francisco-Bay Area, SSAT Tutors in Los Angeles

Popular Courses & Classes

ACT Courses & Classes in San Francisco-Bay Area, GRE Courses & Classes in San Diego, LSAT Courses & Classes in Phoenix, SSAT Courses & Classes in Dallas Fort Worth, SSAT Courses & Classes in Denver, ACT Courses & Classes in Boston, SSAT Courses & Classes in Chicago, ISEE Courses & Classes in Houston, SSAT Courses & Classes in San Francisco-Bay Area, Spanish Courses & Classes in Atlanta

Popular Test Prep

ISEE Test Prep in Seattle, LSAT Test Prep in Atlanta, SSAT Test Prep in Atlanta, SSAT Test Prep in Chicago, GRE Test Prep in Houston, GRE Test Prep in San Diego, SSAT Test Prep in Boston, GRE Test Prep in Seattle, SAT Test Prep in New York City, GRE Test Prep in Phoenix

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SSAT Upper Level Math : How to find the equation of a line

Study concepts, example questions & explanations for SSAT Upper Level Math

All SSAT Upper Level Math Resources

Example Questions

Example Question #1 : Equations Of Lines

Example Question #2 : Equations Of Lines

Example Question #3 : How To Find The Equation Of A Line

Example Question #3 : Equations Of Lines

Example Question #4 : Equations Of Lines

Example Question #4 : Equations Of Lines

Example Question #5 : Equations Of Lines

Example Question #192 : Algebra

Example Question #6 : Equations Of Lines

Example Question #7 : How To Find The Equation Of A Line

All SSAT Upper Level Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: