Algebra II : Setting Up Inequalities
Study concepts, example questions & explanations for Algebra II
All Algebra II Resources
Example Questions
Example Question #1 : Setting Up Inequalities
A grocer decides to mix cashews, almonds, and walnuts and sell the mixture for $14.99/lb. In order for the mixture to be profitable, he would like to make sure that the cost of making the product is less than the selling price.
Cashews cost $8.99/lb
Almonds cost $6.99/lb
Walnuts cost $10.99/lb
Which of the following inequality ensures that the grocer will make a profit by selling the mixture? For one pound of mixture, he uses the following quantities:
x: amount of cashews in the mix
y: amount of almonds in the mix
z: amount of walnuts in the mix
The grocer wants the cost of the mixture to be less than the selling price.
Selling price = 14.99
Find the cost of the mixture
1. cost of cashews in mix:
2. cost of almonds in mix:
3. cost of walnuts in mix:
The cost of the mixture =
Cost of the mixture is less than Selling price
Example Question #1 : Inequalities
You're running a pineapple delivery company and you have 
If we write the number of pineapples ordered as 
6 dollars * (number of pineapples) from 1000 dollars must be greater than or equal to 400 dollars.
This can easily be converted to mathematical symbols as
Example Question #1 : Inequalities
Angie and Kirsten are looking to buy a couch that is less than 
Which set of inequalities represents a solution for how much each girl will spend on the couch?
If Kirsten is represented by the variable 
Example Question #4 : Setting Up Inequalities
Write the following statements as a set of inequalities:
- The total number of shirts and pants I bought did not exceed 20.
- The cost of the total number of pants and shirts I bought was more than 50 dollars.
Where, s is the number of shirts, p is the number of pants and c, is the total cost.
The first statement says that the total number of pants and shirts does not exceed 20. This means that the total can be 20 but nothing more which is represented by:
The second sentence says that the cost was greater than 50 dollars which is represented by:
Example Question #5 : Setting Up Inequalities
Set up the inequality: Twice a number is less than twice the product of the number and five.
To set up this inequality, break up the statement into parts. Assume 
Twice a number:
Is less than:
Product of the number and five:
Twice the product of the number and five:
Connect the statement.
The answer is:
Example Question #6 : Setting Up Inequalities
Set up the inequality expressed in the word problem below:
Elise went to the grocery store, and bought a bunch of sweet potatoes and onions. She knows she has less than 12 altogether. What is the inequality that expresses how many sweet potatoes (x) and onions (y) she could have bought?
Example Question #1 : Inequalities
If 
The point will land on the x or y axis, therefore there is no quadrant for the scenario.
Quadrant III
Quadrant II
Quadrant IV
Quadrant I
Quadrant IV
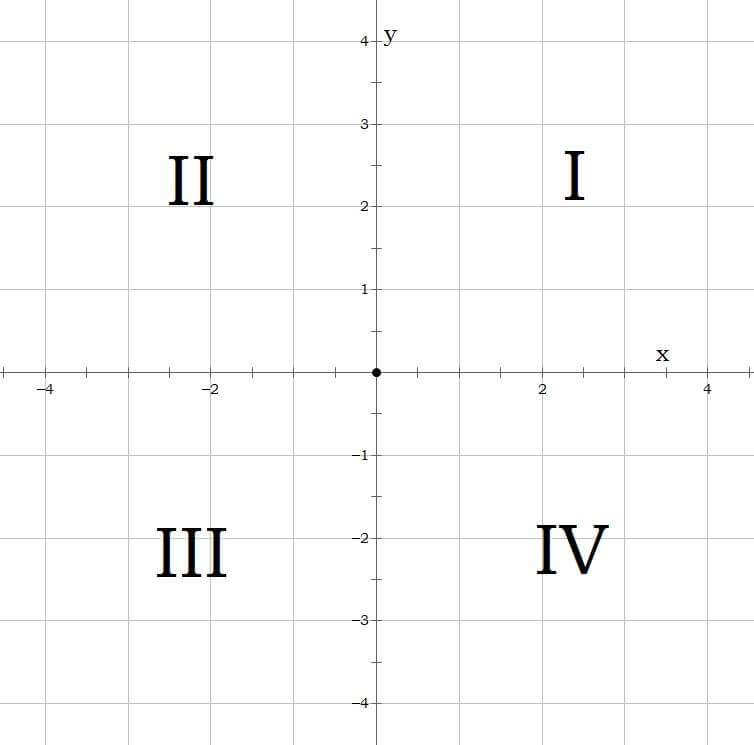
Because x is greater than zero, it will be to the right of the y axis. Because y is less than zero, it will be below the x axis. This is the fourth quadrant.
Example Question #1 : Inequalities
At a fair, there is a game where players step on a scale and weigh themselves. The objective of the game is for the host to guess the player's weight. A player loses if the host of the game can guess the player's weight within 
For the player to lose, the host has to guess within 
Example Question #9 : Setting Up Inequalities
Set up the following inequality: Four less than three times a number squared is at least six.
Split up the inequality into parts.
A number squared:
Three times a number squared:
Four less than three times a number squared:
Is at least six:
Combine all the parts to form an inequality:
The answer is:
Example Question #1 : Setting Up Inequalities
Set up the inequality: Four times the quantity of a number less than five is more than six.
Break up the sentence into parts.
A number less than five:
The quantity of a number less than five:
Four times the quantity of a number less than five:
More than six:
Combine the terms.
The answer is:
Certified Tutor
All Algebra II Resources
