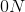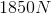All AP Physics 2 Resources
Example Questions
Example Question #1 : Buoyant Force
What is the net force on a ball of mass 

The buoyant force on the ball is simply the weight of water displaced by the ball:
The force of gravity on the ball is:
These forces oppose each other, so we can say:
Example Question #1 : Buoyant Force
A ball of mass 

More information is needed to solve
The net force on the ball is expressed as:
Since it is accelerating downward, we know that the force of gravity is stronger than the buoyant force, so we can write:
Substitute expressions for each variable:
Rearrange to solve for the volume of the ball:
Example Question #2 : Buoyant Force
Suppose that a hollow cylindrical object is floating on the surface of water. This object has a mass of 300g and is floating such that 4cm of its height is submerged under the surface of water, while 6cm of its height is above the water. How much mercury would need to be poured into this cylindrical object in order for it to sink?

Addition of mercury will not cause the cylinder to sink
To answer this question, we'll need to make use of the concept of buoyancy and apply the following equation:
Also, remember that in this equation, the density and volume are that of the fluid that is displaced, not that of the object!
Since we are told that the object has a mass of 300g and is initially floating, we can set the buoyant force equal to the weight of the object.
It's important to realize that the volume of the fluid displaced is going to be equal to that portion of the object's volume that is submerged underwater. Since we're told that the object is cylindrical, and that 4cm of its height is under water, we can set up the following relationship:
Where 

Plugging this value into the above equation and canceling common units on both sides, we obtain:
Now that we have found the area, we can calculate the mass that needs to be added to the cylinder in order to make it sink. To do this, we need to consider the scenario in which the cyclinder is completely submerged in the water the instant before it is about to sink. In this case, we're able to calculate the mass that needs to be added to the cylinder to make this happen.
Where 
Thus far, we have determined that 450g of mercury needs to be added. Now, we just need to use the density of mercury in order to determine the volume needed.
Example Question #1 : Buoyant Force
A metal sample is suspended from a thread and submerged in a beaker of water. The metal sample does not touch the sides or bottom of the beaker. The beaker of water is on a laboratory scale as the metal sample is submerged. What happens to the scale reading as the sample is submerged?
The scale reading does not change
The scale reading first decreases then increases
The scale reading first increases then decreases
The scale reading decreases
The scale reading increases
The scale reading increases
Since the water is exerting an upward force 
Example Question #1 : Buoyant Force
How large would a balloon filled with helium need to be in order to lift a 


The minimum size of the ballon will exert a buoyant force exactly equal to the gravitational force:
Now we have to be careful. The mass is not just the mass of the man, but it includes the mass of the helium, as well. Canceling the 
The mass of the helium is its density times its volume:
Rearrange and solve for 
Example Question #1 : Fluid Statics
A heavy rock is placed in a bucket of water; the rock sinks to the bottom. What is the value of the buoyant force on the rock?
The buoyant force is the weight of the volume of water displaced by the immersed object. Since the rock is completely submerged, the buoyant force is the weight of water with the same volume as the rock. Despite the rock sinking, there is still a buoyant force; it is just less than the weight of the rock.
Example Question #1 : Buoyant Force
Two spherical objects are placed in a bucket of water. One object has mass 


equal to
in opposite direction of
greater than
less than
equal to
Buoyant forces can be thought of as the force caused by displacing the amount of water that was previously there. The buoyant force is the weight of the volume of the liquid displaced by the object. Since the two masses have the same diameter, they have the same volume and thus, the same buoyant force.
Example Question #4 : Buoyant Force
Hanging from a scale is a 

The weight of the object is 


We may relate these parameters by Archimedes' principle:
This allows us to solve for the volume of the sphere, and thus, the radius of the sphere.
Now we may use the formula for volume of a sphere to solve for the radius:
Example Question #3 : Fluids
Suppose that two identical objects are submerged in water. Object A is submerged at a distance of 5m below the surface, whereas Object B is submerged at a distance of 10m below the surface. How does the buoyant force experienced by each object differ?
The buoyant force experienced by each object is the same
We need to know the atmospheric pressure to find out the relative buoyant forces on each object
The buoyant force experienced by Object B is twice that experienced by Object A
The buoyant force experienced by Object A is twice that experienced by Object B
The buoyant force experienced by each object is the same
In this question, we're told that two identical objects are submerged in water. The only thing that differs between them is the distance under the surface of water at which they're submerged. We're then asked to determine how this affects the buoyant force that each one experiences.
To answer this, we'll need to remember the expression for buoyant force.
The above expression tells us that the magnitude of the buoyant force is proportional to the density of the water or fluid, the volume of the water displaced, and the acceleration due to gravity.
It's important to also realize that no where in this expression does it say that the buoyant force is related to the depth of the object. Therefore, because the two objects are the same, and because they're both fully submerged, they will both be displacing the same volume of water. Consequently, the buoyant force on each is the same.
Example Question #10 : Fluids
Determine the net force on a copper ball 

None of these
Convert 

Calculate buoyant force:
Plug in values:
Calculate force due to gravity:
Plug in values and solve:
Plug in values:
All AP Physics 2 Resources