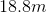We can begin this problem by writing the equation for pressure.

We have to remember that the value of atmospheric pressure is equal to 1atm, which is also equal to 101325Pa.

Now, if we set the gauge pressure equal to three times the atmospheric pressure, we can solve for the depth.

The cube has a volume of . It is necessary to know the sidelength of the cube to know the height of the cube.

Use the pressure formula.

Rewrite the equation to solve for (rho density of the fluid) then plug in known values.

This is approximately 

Pressure increases with depth according to:

Since the pressure at the surface of the ocean is the same as the pressure inside the bathysphere, we can ignore both. (This is called gauge pressure, or pressure above one atmosphere). Find the pressure at our depth:

Find the area of the hatch, and use the definition that force is pressure times area:

The interface between the liquids occurs at the bottom of the tube. Here, the pressure is the same. We also know that the tube is open to atmospheric pressure at the top of each side of the tube. Thus, we can create two equations for the pressure at the interface and set them equal to each other: 


By setting these equal to one another the result is: 
 
Since the density of water is greater than that of oil, we can conclude that the height of the oil column will be greater than that of the water. 

The pressure in a tire is a gauge pressure; the 25psi is the difference between the pressure in the tire and atmospheric pressure, and does not add atmospheric pressure to reach the measured number. Absolute pressure adds these quantities together. 

The pressure at the lake's surface is just the atmospheric pressure, . The pressure at depth  below the surface is . Therefore, the pressure difference is given as .

We can then substitute the values in to arrive at the answer in :

Recall that this pressure is known as gauge pressure, and that the total pressure at the point underwater in the lake includes gauge pressure and atmospheric pressure.

This question is presenting us with a situation in which an object (a person) submerged in a liquid is experiencing pressure. To solve for the correct pressure, we'll need to take a few things into account.

First, we'll need to consider the density of the liquid. In this case, we're told that the liquid is saltwater, and we're given the density in the question stem. Second, we'll need to consider how far below the surface of the liquid our object is. Again, this value is given to us in the question stem. And third, we also need to remember that there is a pressure above the liquid, which is the pressure coming from the atmosphere. This value (which should be committed to memory) is , or .

Putting all these considerations together, we have an equation that we can use to calculate the pressure.

Plug in the values that we know and solve for total pressure.

This is a fairly straight-forward question, providing us with a force vector that is acting upon a surface and asking us for the pressure.

First and foremost, we can examine the equation for pressure:

Next, we'll need to figure out the sign convention for pressure. Based on the above equation, it may seem like if we plug in a negative value for pressure and a positive value for area, we'll get a negative value for pressure. However, this is not the case.

As it turns out, pressure is a scalar quantity. The reason for this is that when any force acts on a surface, it is acting perpendicularly to that surface. Therefore, the direction in which the force is acting is dependent on how the surface is oriented. No matter which way you orient the surface, the force that is acting on that surface will always be perpendicular. Consequently, pressure is essentially just a proportionality between force and area that has no bearing on direction.

To answer this question, it's useful to think of this conceptually as the balloon being "submerged" in the atmosphere. Remember, buoyant forces refer to the force caused by the displacement of a fluid, and both liquids and gasses count as fluids. Thus, in this case, the balloon can be thought of as submerged in a fluid in the same way that any object can be submerged in a liquid such as water.

We're trying to look for the point at which the balloon stops rising. This is analogous to a situation in which the balloon is "floating" in the fluid. In such a case, the upward and downward forces are equal and balance each other out.

So now, we need to identify the upward and downward forces acting on the balloon and set them equal to each other. The upward force will be the buoyant force due to the displacement of air from the atmosphere. The downward force will be the weight of the balloon.

We can further rewrite the mass of the balloon in terms of its weight and density.

Canceling out the gravity term, we obtain:

Next, it's important to realize that the volume of atmosphere being displaced is exactly the same as the volume of the balloon, since it is the balloon that is causing the displacement of air in the atmosphere. Thus:

Furthermore, because these two values are equal, we can cancel them out in the above expression to obtain the following.

Here, we have shown that once the density of the balloon becomes equal to that of the surrounding atmosphere, it will cease rising.

In this question, we're told that three containers of different shapes are filled with water. We're also told that each container has the same depth of water.

To find the pressure at the bottom of any of the containers, we'll need to remember the equation for pressure.

Since each of the containers is open to the atmosphere, we can disregard the term for atmospheric pressure in the above expression. Therefore, the absolute pressure becomes the gauge pressure.

Also, since each container is filled with water, the density of the fluid in each container is identical. Moreover, the depth we are considering for each container is also the same. Therefore, the pressure at the bottom of each container is exactly the same.