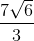All GRE Math Resources
Example Questions
Example Question #23 : Basic Squaring / Square Roots
Simplify:
None of the other answers
Take each fraction separately first:
(2√3)/(√2) = [(2√3)/(√2)] * [(√2)/(√2)] = (2 * √3 * √2)/(√2 * √2) = (2 * √6)/2 = √6
Similarly:
(4√2)/(√3) = [(4√2)/(√3)] * [(√3)/(√3)] = (4√6)/3 = (4/3)√6
Now, add them together:
√6 + (4/3)√6 = (3/3)√6 + (4/3)√6 = (7/3)√6
Example Question #1 : Square Roots And Operations
Compare the quantities.
Quantity A:
Quantity B:
Quantity B is larger.
The relationship cannot be determined from the information given.
The two quantities are equal.
Quantity A is larger.
The two quantities are equal.
Begin by breaking down each of the roots in question. Often, for the GRE, your answer arises out of doing such basic "simplification moves".
Quantity A
This is the same as:

Quantity B
This is the same as:

Thus, at the end of working through the proper math, you realize that the two values are equal!
Example Question #24 : Basic Squaring / Square Roots
Simplify the following expression:
Begin by factoring out each of the radicals:
For the first two radicals, you can factor out a 

The other root values cannot be simply broken down. Now, combine the factors with 
This is your simplest form.
Example Question #2 : Square Roots And Operations
Solve for 
Note, 
Begin by getting your 
Next, you can combine your radicals. You do this merely by subtracting their respective coefficients:
Now, square both sides:
Solve by dividing both sides by 
Example Question #2 : Square Roots And Operations
Simplify the following expression:
To solve this problem, we must realize that the only way to add or subtract square roots is if the number under to square root is equivalent to each other. Therefore we must find a way to simplify each square root.
First we attempt to simplify the first term,
We break apart the number under the square root and find
Simplifying
Therefore we know that in order to try and simplify the other terms, the number under the square root has to be 3. By removing 
For the second term,
Finally for the last term,
Our new equation becomes
Once all number under the square roots become the same, we can treat it as simple addition/subtraction and solve.
Example Question #621 : Gre Quantitative Reasoning
Which of the following is equal to



Example Question #1 : How To Divide Square Roots
Simplify:
Let's combine the two radicals into one radical and simplify.

Remember, when dividing exponents of same base, just subtract the power.
The final answer is 
Example Question #2 : How To Divide Square Roots
Solve for 
If we multiplied top and bottom by 


Then, square both sides to get rid of the radical.

Divide both sides by 

The reason the negative is not an answer is because a negative value in a radical is an imaginary number.
Example Question #2 : How To Divide Square Roots
Rationalize the denominator:
We don't want to have radicals in the denominator. To get rid of radicals, just multiply top and bottom by that radical.
Example Question #42 : Basic Squaring / Square Roots
Simplify:
There are two methods we can use to simplify this fraction:
Method 1:
Factor the numerator:

Remember, we need to factor out perfect squares.
Method 2:
You can combine the fraction into one big square root.

Then, you can simplify the fraction.
All GRE Math Resources