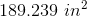All High School Math Resources
Example Questions
Example Question #2 : How To Find The Surface Area Of A Prism
Angie is painting a 2 foot cube for a play she is in. She needs 
It is impossible to convert between metric units and feet.
None of the available answers
First we must calculate the surface area of the cube. We know that there are six surfaces and each surface has the same area:
Now we will determine the amount of paint needed
Example Question #1 : How To Find The Surface Area Of A Prism
Find the surface area of the following triangular prism.

The formula for the surface area of a triangular prism is:
Where 



Use the formula for a 
Plugging in our values, we get:
Example Question #84 : Solid Geometry
Find the surface area of the following triangular prism.

The formula for the surface area of a triangular prism is:
Where 



Use the formula for a 
Plugging in our values, we get:
Example Question #1941 : High School Math
Find the surface area of the following triangular prism.

The formula for the surface area of an equilateral, triangular prism is:
Where 

Plugging in our values, we get:
Example Question #971 : Act Math
David wants to paint the walls in his bedroom. The floor is covered by a 



1 gallon and 1 quart
1 gallon and 2 quarts
1 gallon
2 gallons and 1 quart
3 quarts
1 gallon and 2 quarts
Find the surface area of the walls: SAwalls = 2lh + 2wh, where the height is 8 ft, the width is 10 ft, and the length is 16 ft.
This gives a total surface area of 416 ft2. One gallon covers 300 ft2, and each quart covers 75 ft2, so we need 1 gallon and 2 quarts of paint to cover the walls.
Example Question #1 : How To Find The Surface Area Of A Prism
A box is 5 inches long, 5 inches wide, and 4 inches tall. What is the surface area of the box?
The box will have six total faces: an identical "top and bottom," and identical "left and right," and an identical "front and back." The total surface area will be the sum of these faces.
Since the six faces consider of three sets of pairs, we can set up the equation as:
Each of these faces will correspond to one pair of dimensions. Multiply the pair to get the area of the face.
Substitute the values from the question to solve.
Example Question #2 : How To Find The Surface Area Of A Prism
What is the surface area of a rectangular brick with a length of 12 in, a width of 8 in, and a height of 6 in?
None of the answers are correct
The formula for the surface area of a rectangular prism is given by:
SA = 2LW + 2WH + 2HL
SA = 2(12 * 8) + 2(8 * 6) + 2(6 * 12)
SA = 2(96) + 2(48) + 2(72)
SA = 192 + 96 + 144
SA = 432 in2
216 in2 is the wrong answer because it is off by a factor of 2
576 in3 is actually the volume, V = L * W * H
Example Question #1 : Non Cubic Prisms
What is the surface area of an equilateral triangluar prism with edges of 6 in and a height of 12 in?
Let 

The surface area of the prism can be broken into three rectangular sides and two equilateral triangular bases.
The area of the sides is given by: 

The equilateral triangle is also an equiangular triangle by definition, so the base has congruent sides of 6 in and three angles of 60 degrees. We use a special right traingle to figure out the height of the triangle: 30 - 60 - 90. The height is the side opposite the 60 degree angle, so it becomes 
The area for a triangle is given by 

Therefore the total surface area is 
All High School Math Resources