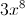When multiplying rational expressions, we simply have to multiply the numerators together and the denominators together. (Warning: you only need to find a lowest common denominator when adding or subtracting, but not when multiplying or dividing rational expression.)

In order to simplify this, we will need to factor  and . Because  looks a little simpler, let's start with it first.

We can easily factor out a four from both terms.

.

Next, notice that  fits the form of our difference of squares factoring formula. In general, we can factor  as . In the polynomial  we will let  and . Thus, . 

Now, we can see that.

We then factor . This also fits our difference of squares formula; however, this time  and . In other words, . Applying the formula, we see that

. Now, let's take our factorization one step further and factor , which we already did above.

. 

Be careful here. A common mistake that students make is to try to factor . There is no sum of squares factoring formula. In other words, in general, if we have , we can't factor it any further. (It is considered prime.)

We will then put all of these pieces of information in order to simplify our rational expression.

Lastly, we cancel the factors that appear in both the numerator and the denominator. We can cancel an  and a  term. 

.

The answer is .

To solve this problem, plug into and simplify. Then plug that expression into :

When multiplying an even number of negatives, you get a positive.

When multiplying an odd number of negative, you get a negative.

When multiplying an odd number of negatives, the answer is negative.

When multiplying an even number of negatives, the answer is positive.

.  However,  cannot be simplified any further because the terms have different exponents.

(Like terms are terms that have the same variables with the same exponents. Only like terms can be combined together.)

Change the mixed numbers into improper fractions by multiplying the whole number by the denominator and adding the numerator:

Dividing by a fraction is the same as multiplying by the reciprocal, so the problem becomes .

The domain of a function consists of all of the values of x for which f(x) is defined. When determining the domain of a funciton, the three most important things we want to consider are square roots, logarithms, and denominators of fractions. These tend to signal places where the function is not defined.

First, let's look at the  term. Remember we can only find the square root of nonnegative values. Thus, everything under the square root symbol must be greater than or equal to zero. This tells us that, for this function, .

Second, we need to look at the natural logarithm. The natural logarithm can only be applied to positive numbers (which don't include zero). Thus, everything within the paranethesis of the natural logarithm must be greater than zero.

There are several ways to solve this inequality. One way is to factor the left side and examine the factors. We know that  because of the difference of squares factorization formula. 

.

This statement will only be true in two situations; either both factors must be positive, or both must be negative.

We can see that if , then the factor  will be positive, but the  factor will be negative. If we were to multiply a negative and a positive number, we would get a negative number. Thus,  is not larger than zero when .

Let's consider the interval . In this case, both  and  would be positive. Thus,  when .

Third, consider the interval . In this case, the first factor will be negative, and the second will be positive, so their product would be negative, and  would not be greater than zero. 

To summarize,  only if .

We can see now that f(x) is only defined if  and .

There is one more piece of information we need to consider--the denominator of f(x). Remember that a fraction is not defined if its denominator equals zero. Thus, if the denominator is equal to zero at a certain value of x, we can't include this value of x in the domain of f(x).

We can set the denominator equal to zero and solve to see if there are any values of x where the denominator would be zero.

Rewrite this as an exponential equation. In general, the equation  can be rewritten as , provided that a is positive. 

If we put  into exponential form, we obtain

We can solve this for x.

So, let's put all of this information together. We know that f(x) is only defined if ALL of these conditions are met: 

The only interval for which this is true is if x is greater than (and not equal to) zero but less than (and not equal to) 1. Thus, the domain of f(x) is .

The answer is .