HiSET: Math : Special triangles
Study concepts, example questions & explanations for HiSET: Math
All HiSET: Math Resources
Example Questions
Example Question #2 : Understand Right Triangles
Two of a triangle's interior angles measure 
A triangle that has interior angles of 
Since we know this triangle is a 30-60-90 triangle, we can use the special ratios that always hold true for this triangle's sides and angles to figure out the lengths of its other sides. The following ratio holds true for all 30-60-90 triangles, where the side in a fraction with a given angle is the side opposite that angle.
We're told that the hypotenuse of our triangle has a length of 
As you can see, for this particular triangle, 
Thus, the correct answer is 
Example Question #131 : Measurement And Geometry
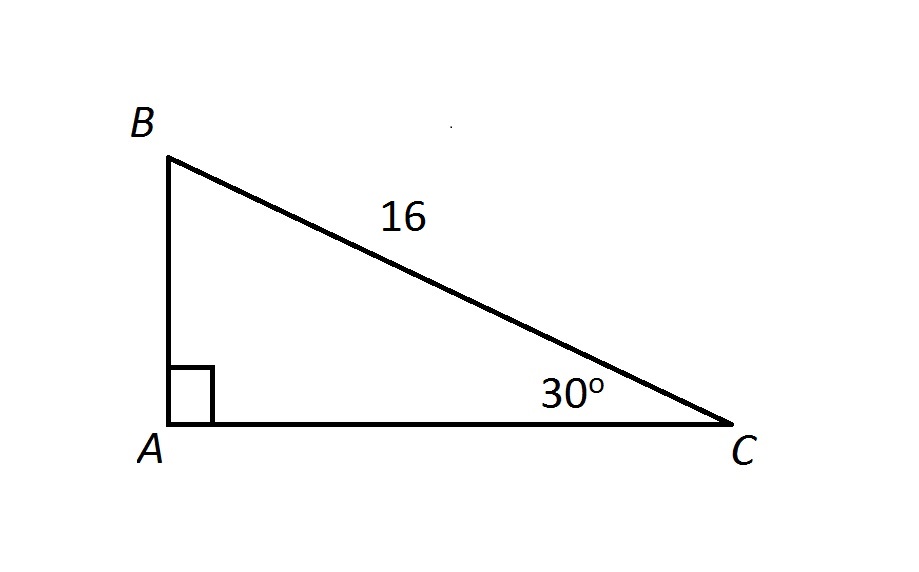
Examine the above triangle. Which of the following correctly gives the area of 
None of the other choices gives the correct response.
Since 
making 
By the 30-60-90 Triangle Theorem,
and
Refer to the diagram below:
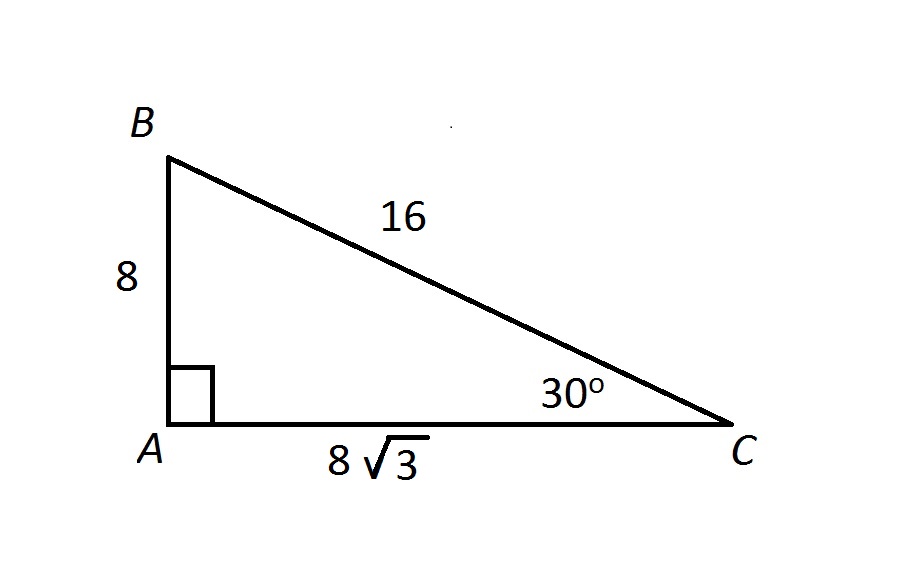
The area of a right triangle is equal to half the product of the lengths of its legs, so
the correct response.
Example Question #1 : Special Triangles
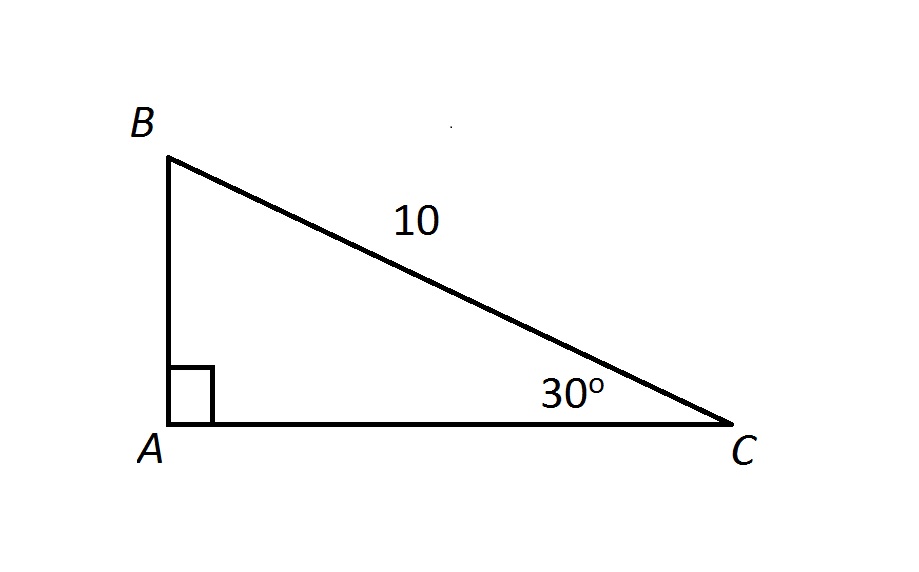
Examine the above triangle. Which of the following correctly gives the perimeter of 
Since 
making 
By the 30-60-90 Triangle Theorem,
and
Refer to the diagram below:
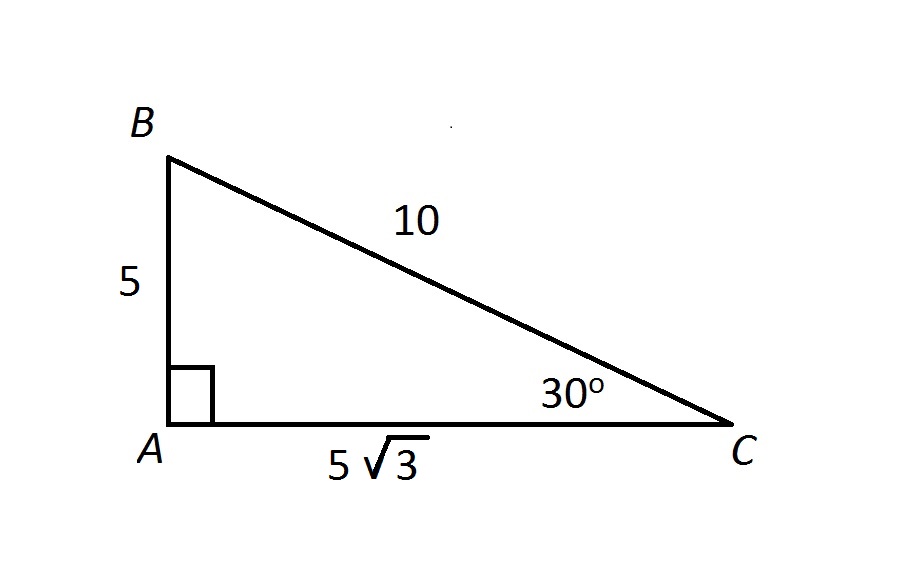
The perimeter - the sum of the sidelengths - is
All HiSET: Math Resources
