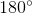We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

Intermediate Geometry : How to find an angle in a hexagon

Study concepts, example questions & explanations for Intermediate Geometry

Create An Account

All Intermediate Geometry Resources

8 Diagnostic Tests 250 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : How To Find An Angle In A Hexagon

There is a regular hexagon with a side length of $4 \:cm$ . What is the measure of an internal angle?

Possible Answers:

cannot be determined

$180^{\circ}$

$120^{\circ}$

$720^{\circ}$

$60^{\circ}$

Correct answer:

$120^{\circ}$

Explanation:

Given that the hexagon is a regular hexagon, this means that all the side length are congruent and all internal angles are congruent. The question requires us to solve for the measure of an internal angle. Given the aforementioned definition of a regular polygon, this means that there must only be one correct answer.

In order to solve for the answer, the question provides additional information that isn't necessarily required. The measure of an internal angle can be solved for using the equation:

$\frac{180^{\circ}(n-2)}{n}$ where is the number of sides of the polygon.

In this case, n = 6 .

For this problem, the information about the side length may be negated.

$\frac{180^{\circ}(6-2)}{6}$

$\frac{180^{\circ}(4)}{6}$

$\frac{180^{\circ}(2)}{3}$

$120^{\circ}$

Report an Error

Example Question #1 : How To Find An Angle In A Hexagon

What is the interior angle of a regular hexagon if the area is 15?

Possible Answers:

120

156

180

720

Correct answer:

120

Explanation:

The area has no relevance to find the angle of a regular hexagon.

There are 6 sides in a regular hexagon. Use the following formula to determine the interior angle.

$\theta=(n-2)\cdot 180$

Substitute n=6 sides to determine the sum of all interior angles of the hexagon in degrees.

$\sum\theta=(6-2)\cdot 180=720$

Since there are 6 sides, divide this number by 6 to determine the value of each interior angle.

$\frac{720}{6}=120$

Report an Error

Example Question #1 : How To Find An Angle In A Hexagon

Given: Regular Hexagon HEXAGO with center . Construct segments $\overline{CA}$ and $\overline{CO}$ to form Quadrilateral CAGO .

True or false: Quadrilateral CAGO is a rectangle.

Possible Answers:

True

False

Correct answer:

False

Explanation:

Below is regular Hexagon HEXAGO with center , a segment drawn from to each vertex - that is, each of its radii drawn.

Hexagon 2

Each angle of a regular hexagon measures $120^{\circ }$ ; by symmetry, each radius bisects an angle of the hexagon, so

$m \angle CAG = 60 ^{\circ }$ .

The angles of a rectangle must measure $90^{\circ }$ , so it has been disproved that Quadrilateral CAGO is a rectangle.

Report an Error

Example Question #901 : Plane Geometry

True or false: Each of the six angles of a regular hexagon measures $120^{\circ }$ .

Possible Answers:

True

False

Correct answer:

True

Explanation:

A regular polygon with sides has congruent angles, each of which measures

$\frac{(N-2)180^{\circ }}{N}$

Setting N = 6 , the common angle measure can be calculated to be

$\frac{(6-2)180^{\circ }}{6} = \frac{4 \cdot 180^{\circ }}{6} = \frac{720^{\circ }}{6} = 120^{\circ }$

The statement is therefore true.

Report an Error

Example Question #1 : How To Find An Angle In A Hexagon

True or false: Each of the exterior angles of a regular hexagon measures $80^{\circ }$ .

Possible Answers:

False

True

Correct answer:

False

Explanation:

If one exterior angle is taken at each vertex of any polygon, and their measures are added, the sum is $360^{\circ }$ . Each exterior angle of a regular hexagon has the same measure, so if we let be that common measure, then

$6t = 360 ^{\circ }$

Solve for :

$6t \div 6 = 360 ^{\circ } \div 6$

$t = 60^{\circ }$

The statement is false.

Report an Error

Example Question #1 : How To Find An Angle In A Hexagon

Given: Hexagon HEXAGO .

$m \angle H = 108 ^{\circ }$

True, false, or undetermined: Hexagon HEXAGO is regular.

Possible Answers:

True

Undetermined

False

Correct answer:

False

Explanation:

Suppose Hexagon HEXAGO is regular. Each angle of a regular polygon of sides has measure

$\frac{(N-2)180^{\circ }}{N}$

A hexagon has 6 sides, so set N = 6 ; each angle of the regular hexagon has measure

$\frac{(6-2)180^{\circ }}{6} = \frac{ 4 \cdot 180^{\circ }}{6} = 120^{\circ }$

Since one angle is given to be of measure $108 ^{\circ }$ , the hexagon cannot be regular.

Report an Error

Example Question #903 : Plane Geometry

What is the measure of one exterior angle of a regular twenty-sided polygon?

Possible Answers:

$18^{\circ }$

$12^{\circ }$

$20^{\circ }$

$15^{\circ }$

$16^{\circ }$

Correct answer:

$18^{\circ }$

Explanation:

The sum of the exterior angles of any polygon, one at each vertex, is $360^{\circ }$ . In a regular polygon, the exterior angles all have the same measure, so divide 360 by the number of angles, which, here, is 20, the same as the number of sides.

$360\div 20 = 18$

Report an Error

Example Question #2 : How To Find An Angle In A Hexagon

Which of the following cannot be the six interior angle measures of a hexagon?

Possible Answers:

$95^{\circ }, 105^{\circ }, 115^{\circ }, 125^{\circ }, 135^{\circ }, 145^{\circ }$

All of these can be the six interior angle measures of a hexagon.

$110^{\circ },110^{\circ }, 110^{\circ }, 110^{\circ }, 110^{\circ }, 170^{\circ }$

$118^{\circ },119^{\circ }, 120^{\circ }, 120^{\circ }, 121^{\circ }, 122^{\circ }$

$120^{\circ }, 120^{\circ }, 120^{\circ }, 120^{\circ }, 120^{\circ }, 120^{\circ }$

Correct answer:

All of these can be the six interior angle measures of a hexagon.

Explanation:

The sum of the interior angle measures of a hexagon is $180 (6-2)=720^{\circ }$

Add the angle measures in each group.

120+120+120+120+120+120=720

95+105+115+125+135+145=720

110+110+110+110+110+170=720

118+119+120+120+121+122=720

In each case, the angle measures add up to 720, so the answer is that all of these can be the six interior angle measures of a hexagon.

Report an Error

View Tutors

William
Certified Tutor

Indiana University-South Bend, Bachelor in Arts, Business, General. Indiana University-South Bend, Masters in Business Admini...

View Tutors

Taylor
Certified Tutor

McGill University, Bachelor in Arts, Music Teacher Education.

View Tutors

Juancarlos
Certified Tutor

CUNY Lehman College, Bachelor of Science, Computer Science. CUNY New York City College of Technology, Master of Science, Math...

All Intermediate Geometry Resources

8 Diagnostic Tests 250 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Math Tutors in Phoenix, Chemistry Tutors in Boston, ISEE Tutors in San Diego, ACT Tutors in Atlanta, Spanish Tutors in San Diego, Math Tutors in Los Angeles, LSAT Tutors in Seattle, Physics Tutors in Seattle, LSAT Tutors in San Francisco-Bay Area, Chemistry Tutors in Atlanta

Popular Courses & Classes

ISEE Courses & Classes in New York City, GMAT Courses & Classes in Phoenix, GRE Courses & Classes in Seattle, GRE Courses & Classes in Washington DC, ISEE Courses & Classes in San Francisco-Bay Area, SSAT Courses & Classes in Philadelphia, ACT Courses & Classes in New York City, Spanish Courses & Classes in Philadelphia, MCAT Courses & Classes in Chicago, MCAT Courses & Classes in Miami

Popular Test Prep

GMAT Test Prep in Denver, GRE Test Prep in Miami, ISEE Test Prep in Denver, SAT Test Prep in Houston, ACT Test Prep in New York City, GMAT Test Prep in Phoenix, SAT Test Prep in Washington DC, ISEE Test Prep in Philadelphia, LSAT Test Prep in Denver, SAT Test Prep in Los Angeles

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Intermediate Geometry : How to find an angle in a hexagon

Study concepts, example questions & explanations for Intermediate Geometry

All Intermediate Geometry Resources

Example Questions

Example Question #1 : How To Find An Angle In A Hexagon

Example Question #1 : How To Find An Angle In A Hexagon

Example Question #1 : How To Find An Angle In A Hexagon

Example Question #901 : Plane Geometry

Example Question #1 : How To Find An Angle In A Hexagon

Example Question #1 : How To Find An Angle In A Hexagon

Example Question #903 : Plane Geometry

Example Question #2 : How To Find An Angle In A Hexagon

All Intermediate Geometry Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: