ACT Math : Trigonometry
Study concepts, example questions & explanations for ACT Math
All ACT Math Resources
Example Questions
Example Question #1 : Trigonometry
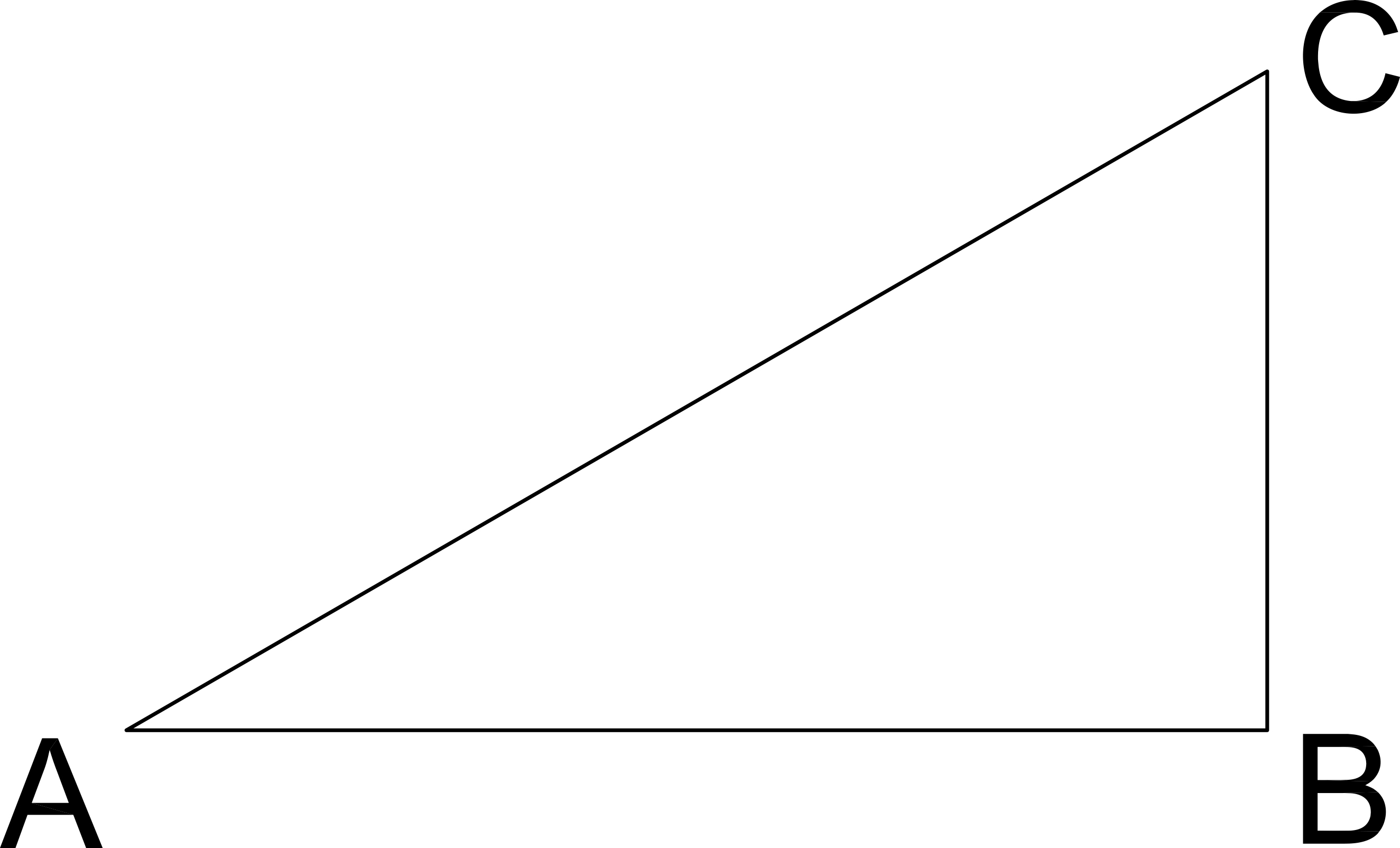
What is the tangent of C in the given right triangle??
Tangent = Opposite / Adjacent
Example Question #1 : Trigonometry
Consider a right triangle with an inner angle 
If
and
what is 
The tangent of an angle x is defined as
Substituting the given values for cos x and sin x, we get
Example Question #1 : Tangent
Triangle ABC shown is a right triangle. If the tangent of angle C is 
Use the definition of the tangent and plug in the values given:
tangent C = Opposite / Adjacent = AB / BC = 3 / 7
Therefore, BC = 7.
Example Question #4 : Tangent
If the sine of an angle equals 
The cosine of the angle is 
The sine of the angle equals 
Since 
Example Question #3 : Trigonometry
For the above triangle, 
This triangle cannot exist.
With right triangles, we can use SOH CAH TOA to solve for unknown side lengths and angles. For this problem, we are given the opposite and adjacent sides of the triangle with relation to the angle. With this information, we can use the tangent function to find the angle.
Example Question #1 : How To Find An Angle With Tangent
In the above triangle, 
With right triangles, we can use SOH CAH TOA to solve for unknown side lengths and angles. For this problem, we are given the opposite and adjacent sides of the triangle with relation to the angle. With this information, we can use the tangent function to find the angle.
Example Question #1 : How To Find An Angle With Tangent
For the above triangle, 
This triangle cannot exist.
With right triangles, we can use SOH CAH TOA to solve for unknown side lengths and angles. For this problem, we are given the opposite and adjacent sides of the triangle with relation to the angle. With this information, we can use the tangent function to find the angle.
Example Question #1 : How To Find An Angle With Tangent
A laser is placed at a distance of 
You can draw your scenario using the following right triangle:
Recall that the tangent of an angle is equal to the ratio of the opposite side to the adjacent side of the triangle. You can solve for the angle by using an inverse tangent function:
Example Question #4 : Trigonometry
What is the value of 
Recall that the tangent of an angle is equal to the ratio of the opposite side to the adjacent side of the triangle. You can solve for the angle by using an inverse tangent function:
Example Question #1 : How To Find A Missing Side With Tangent
7
6
8
10
9
9
First, solve for side MN. Tan(30°) = MN/16√3, so MN = tan(30°)(16√3) = 16. Triangle LMN and MNO are similar as they're both 30-60-90 triangles, so we can set up the proportion LM/MN = MN/NO or 16√3/16 = 16/x. Solving for x, we get 9.24, so the closest whole number is 9.
Certified Tutor
All ACT Math Resources
