All Calculus 1 Resources
Example Questions
Example Question #51 : Intervals
Find the interval(s) where the following function is concave down. Graph to double check your answer.
Never
Always
To find when a function is concave, you must first take the 2nd derivative, then set it equal to 0, and then find between which zero values the function is negative.
First, find the 2nd derivative:
Set equal to 0 and solve:
Now test values on all sides of these to find when the function is negative, and therefore decreasing. I will test the values of -3 and 0.
Since the value that is negative is when x=-3, the interval is decreasing on the interval that includes 0. Therefore, our answer is:
Example Question #1611 : Functions
Find the interval(s) where the following function is concave down. Graph to double check your answer.
Never
Always
To find when a function is concave, you must first take the 2nd derivative, then set it equal to 0, and then find between which zero values the function is negative.
First, find the 2nd derivative:
Set equal to 0 and solve:
Now test values on all sides of these to find when the function is negative, and therefore decreasing. I will test the values of 0 and 6.
Since the value that is negative is when x=0, the interval is decreasing on the interval that includes 0. Therefore, our answer is:
Example Question #1611 : Functions
Is 

Yes, 

Yes, 

No, 

No, 

Cannot be determined by the information given
Yes, 

To test concavity, we must first find the second derivative of f(x)
This function is concave down anywhere that f''(x)<0, so...
So,

So on the interval -5,-4 f(x) is concave down because f''(x) is negative.
Example Question #1621 : Functions
Is 
![[5,7]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/337522/gif.latex)
Concave up, because 
Concave up, because 
Concave down, because 
Concave down, because 

Concave up, because 
To test concavity, we need to perform the second derivative test. Bascially, assuming that our function is twice-differentiable, anywhere that h"(t) is positive will be concave up, and anywhere h"(t) is negative is concave down. Begin as follows:
Next, we need to evaluate h"(t) on the interval [5,7]
So, our h"(t) is positive on the interval, and therefore h(t) is concave up.
Example Question #172 : Graphing Functions
Find the intervals where 
The intervals where a function is concave up or down is found by taking second derivative of the function. Use the power rule which states:
Now, set 

In this case, 
To find the concave up region, find where 


If we plug in 

If we plug in 


Example Question #173 : Graphing Functions
Find the intervals that are concave down in 

To find which interval is concave down, find the second derivative of the function.
Now, find which 



Now to find which interval is concave down choose any value in each of the regions

and plug in those values into 
A test value of 



Example Question #7 : How To Find Concave Down Intervals By Graphing Functions
The function 

![[0, 2\pi]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/351759/gif.latex)
It is never concave down.
It is concave down over the entire interval
The derivative of 
The derivative of this is
This is the second derivative.
A function is concave down if its second derivative is less than 0.

This is true when:
Example Question #52 : Intervals
Given the equation of a graph is 
This graph is always concave up.
To find the concavity of a graph, the double derivative of the graph equation has to be taken. To take the derivative of this equation, we must use the power rule,

We also must remember that the derivative of an constant is 0.
After taking the first derivative of the equation using the power rule, we obtain

The double derivative of the equation we are given comes out to

Setting the equation equal to zero, we find that 


Example Question #9 : How To Find Concave Down Intervals By Graphing Functions
Determine the intervals on which the following function is concave down:
To find the invervals where a function is concave down, you must find the intervals on which the second derivative of the function is negative. To find the intervals, first find the points at which the second derivative is equal to zero. The first derivative of the function is equal to

The second derivative of the function is equal to

Both derivatives were found using the power rule

Solving for x, 


On the first interval, the second derivative is negative, which means the function is concave down. On the second interval, the second derivative is positive, which means the function is concave up. (Plug in values on the intervals into the second derivative and see if they are positive or negative.)
Thus, the first interval 
Example Question #1 : How To Find Concave Down Intervals By Graphing Functions
How many infelction points does the function
have on the interval ![[-5, 5]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/369991/gif.latex)
Three
Zero
Two
One
Three
Points of inflection occur where there second derivative of a function are equal to zero. Taking the first and second derivative of the function, we find:
To find the points of inflection, we find the values of x that satisfy the condition

Which occurs at
Within the defined interval [-5, 5], there are three values: 

All Calculus 1 Resources















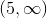
































![\left[\frac{3\pi }{2},\infty\right]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/344652/gif.latex)
![[0,2\pi ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/340903/gif.latex)
![\left[0,\frac{\pi }{2}\right]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/344650/gif.latex)
![\left[\pi ,\frac{3\pi }{2}\right]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/344651/gif.latex)
![[0,\pi ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/340902/gif.latex)


























