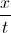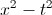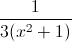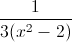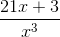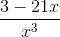GRE Math : Rational Expressions

Study concepts, example questions & explanations for GRE Math

Create An Account

All GRE Math Resources

13 Diagnostic Tests 452 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #911 : Algebra

Which of the following is equivalent to $\dpi{100} \frac{(\frac{1}{t}-\frac{1}{x})}{x-t}$ ? Assume that denominators are always nonzero.

Possible Answers:

$x^{2}-t^{2}$

$\frac{x}{t}$

$(xt)^{-1}$

$t-x$

$x-t$

Correct answer:

$(xt)^{-1}$

Explanation:

We will need to simplify the expression $\frac{(\frac{1}{t}-\frac{1}{x})}{x-t}$ . We can think of this as a large fraction with a numerator of $\frac{1}{t}-\frac{1}{x}$ and a denominator of $\dpi{100} x-t$ .

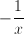
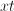
In order to simplify the numerator, we will need to combine the two fractions. When adding or subtracting fractions, we must have a common denominator. $\frac{1}{t}$ has a denominator of $\dpi{100} t$ , and $\dpi{100} -\frac{1}{x}$ has a denominator of $\dpi{100} x$ . The least common denominator that these two fractions have in common is $\dpi{100} xt$ . Thus, we are going to write equivalent fractions with denominators of $\dpi{100} xt$ .

In order to convert the fraction $\dpi{100} \frac{1}{t}$ to a denominator with $\dpi{100} xt$ , we will need to multiply the top and bottom by $\dpi{100} x$ .

$\frac{1}{t}=\frac{1\cdot x}{t\cdot x}=\frac{x}{xt}$

Similarly, we will multiply the top and bottom of $\dpi{100} -\frac{1}{x}$ by $\dpi{100} t$ .

$\frac{1}{x}=\frac{1\cdot t}{x\cdot t}=\frac{t}{xt}$

We can now rewrite $\frac{1}{t}-\frac{1}{x}$ as follows:

$\frac{1}{t}-\frac{1}{x}$ = $\frac{x}{xt}-\frac{t}{xt}=\frac{x-t}{xt}$

Let's go back to the original fraction $\frac{(\frac{1}{t}-\frac{1}{x})}{x-t}$ . We will now rewrite the numerator:

$\frac{(\frac{1}{t}-\frac{1}{x})}{x-t}$ = $\frac{\frac{x-t}{xt}}{x-t}$

To simplify this further, we can think of $\frac{\frac{x-t}{xt}}{x-t}$ as the same as $\frac{x-t}{xt}\div (x-t)$ . When we divide a fraction by another quantity, this is the same as multiplying the fraction by the reciprocal of that quantity. In other words, $a\div b=a\cdot \frac{1}{b}$ .

$\frac{x-t}{xt}\div (x-t)$ = $\frac{x-t}{xt}\cdot \frac{1}{x-t}$ $=\frac{x-t}{xt(x-t)}$ $= \frac{1}{xt}$

Lastly, we will use the property of exponents which states that, in general, $\frac{1}{a}=a^{-1}$ .

$\frac{1}{xt}=(xt)^{-1}$

The answer is $(xt)^{-1}$ .

Report an Error

Example Question #2 : How To Divide Rational Expressions

Simplify:

$\frac{x^{2}+x-2}{3x^{2} + 9x + 6} \div (x-1)$

Possible Answers:

$\frac{1}{3(x+1)}$

$\frac{1}{3(x-1)}$

$\frac{1}{3(x+2)}$

$\frac{1}{3(x^{2}+1)}$

$\frac{1}{3(x^{2}-2)}$

Correct answer:

$\frac{1}{3(x+1)}$

Explanation:

Multiply by the reciprocal of $\frac{x-1}1{}$ .

$\frac{x^{2}+x-2}{3x^{2}+9x+6} \cdot \frac{1}{x-1}$

Factor $\frac{(x-1)(x+2)}{3(x+2)(x+1)} \cdot \frac{1}{x-1}$

Divide by common factors.

$= \frac{1}{3(x+1)}$

Report an Error

Example Question #3 : Expressions

Solve for :

$\frac{\frac{2m^2 + 5}{4}}{ \frac{m}{2}} = 20$

Possible Answers:

m = 12

m = -10

m = -12,-8

m = 8

m=19.87,0.13

Correct answer:

m=19.87,0.13

Explanation:

To tackle this problem, you need to invert and multiply:

$\frac{\frac{2m^2 + 5}{4}} { \frac{m}{2}} = 20$

$\frac{2m^2 + 5}{4} \cdot \frac{2}{m} = 20$

$\frac{4m^2 + 10}{4m} = 20$

4m^2+10=80m

Here we see that we have created a quadratic equation. Therefore, we get all terms to one side, set it equal to zero and use the quadratic formula to solve.

4m^2-80m+10=0

The quadratic formula is:

$m=\frac{-b\pm\sqrt{b^2-4ac}}{2a}$ where a=4, b=-80, c=10

Plugging these values in we get the following:

$\frac{80\pm\sqrt{80^2-4(4)(10)}}{2(4)}=\frac{80\pm\sqrt{6240}}{8}=\frac{80\pm78.99}{8}$

m=19.87,0.13

Report an Error

Example Question #3 : Rational Expressions

Express the following as a single rational expression:

$\frac{\frac{2x^2 + 7}{12b} }{ \frac{y^2 + 2y}{3a^2 + 2}}$

Possible Answers:

$\frac{x^2a^2 +21a^2 +4x^2 +14}{12by^2 + 24by}$

$\frac{6x^2a^2 +84a^2x^2 +14}{12by^2 + 24by}$

$\frac{6x^2a^2 +21a^2 +4x^2 +14}{12by^2 + 24by}$

$\frac{6x^2a^2 +21a^2 +4x^2 +14}{36by}$

$\frac{6x^2a^2 +21a^2 +4x^2 +14}{12by^2 + by}$

Correct answer:

$\frac{6x^2a^2 +21a^2 +4x^2 +14}{12by^2 + 24by}$

Explanation:

To divide one rational expression by another, invert and multiply:

$\frac{\frac{2x^2 + 7}{12b}}{ \frac{y^2 + 2y}{3a^2 + 2}}$

$\frac{2x^2 + 7 }{12b} \cdot \frac{3a^2 + 2}{y^2 + 2y}$

Remember to foil the numerator meaning, multiply the first components of each binomial. Then multiply the outer components of each binomial. After that, multiply the inner components together, and lastly, multiply the components in the last position of the binomials together.

This arrives at the following:

$\frac{6x^2a^2 +21a^2 +4x^2 +14}{12by^2 + 24by}$

You can't factor anything out, so that's your final answer.

Report an Error

Example Question #1 : Expressions

Simplify the following rational expression: (9x - 2)/(x²) MINUS (6x - 8)/(x²)

Possible Answers:

$\frac{3x+6}{x^{2}}$

3x-10

$\frac{15x+6}{x}$

$\frac{12x-6}{x}$

Correct answer:

$\frac{3x+6}{x^{2}}$

Explanation:

Since both expressions have a common denominator, x², we can just recopy the denominator and focus on the numerators. We get (9x - 2) - (6x - 8). We must distribute the negative sign over the 6x - 8 expression which gives us 9x - 2 - 6x + 8 ( -2 minus a -8 gives a +6 since a negative and negative make a positive). The numerator is therefore 3x + 6.

Report an Error

Example Question #3 : How To Add Rational Expressions With A Common Denominator

Simplify the following rational expression:

$\frac{7x-18}{x^{2}}+\frac{6x-14}{x^{2}}$

Possible Answers:

$\frac{13x-28}{x^{2}}$

$\frac{13x-32}{x^{2}}$

$\frac{x-32}{x^{2}}$

$\frac{13x-4}{x^{2}}$

$\frac{x-4}{x^{2}}$

Correct answer:

$\frac{13x-32}{x^{2}}$

Explanation:

Since both fractions in the expression have a common denominator of $x^{2}$ , we can combine like terms into a single numerator over the denominator:

$\frac{7x-18}{x^{2}}+\frac{6x-14}{x^{2}}$

$=\frac{(7x-18)+(6x-14)}{x^{2}}$

$=\frac{13x-32}{x^{2}}$

Report an Error

Example Question #4 : How To Add Rational Expressions With A Common Denominator

Simplify the following expression:

$\frac{10x-9}{x^{3}}+\frac{11x+12}{x^{3}}$

Possible Answers:

$\frac{3-21x}{x^{3}}$

$\frac{21x+3}{x^{3}}$

$\frac{3-x}{x^{3}}$

$\frac{21x-3}{x^3}$

$\frac{x+3}{x^{3}}$

Correct answer:

$\frac{21x+3}{x^{3}}$

Explanation:

Since both terms in the expression have the common denominator $x^{3}$ , combine the fractions and simplify the numerators:

$\frac{10x-9}{x^{3}}+\frac{11x+12}{x^{3}}$

$=\frac{(10x-9)+(11x+12)}{x^{3}}$

$=\frac{21x+3}{x^{3}}$

Report an Error

Example Question #5 : How To Add Rational Expressions With A Common Denominator

Simplify the following rational expression:

$\frac{5x-5}{2x^{2}} + \frac{7x+9}{2x^{2}}$

Possible Answers:

$\frac{6x+4}{2x^{2}}$

$\frac{8x+4}{2x^{2}}$

$\frac{12x+8}{2x^{2}}$

$\frac{6x+2}{x^{2}}$

$\frac{6x+8}{2x^{2}}$

Correct answer:

$\frac{6x+2}{x^{2}}$

Explanation:

Since both rational terms in the expression have the common denominator $2x^{2}$ , combine the numerators and simplify like terms:

$\frac{5x-5}{2x^{2}} + \frac{7x+9}{2x^{2}}$

$=\frac{(5x-5)+(7x+9)}{2x^{2}}$

$=\frac{12x+4}{2x^{2}}$

$=\frac{6x+2}{x^2}$

Report an Error

Example Question #281 : Algebra

Add and simplify:

$\frac{3x^2 + 3x + 3}{2y} + \frac{10x^2 + 3x}{2y}$

Possible Answers:

$\frac{10x^2 + 3x^2 +3x + 3x + 3}{2y}$

$\frac{6x^2 + 3x +1}{2y}$

$\frac{13x^2 + 6x + 3}{2y}$

$\frac{13x^2 + 3x + 3}{2y}$

$\frac{13x^2 + 6x + 3}{4y}$

Correct answer:

$\frac{13x^2 + 6x + 3}{2y}$

Explanation:

When adding rational expressions with common denominators, you simply need to add the like terms in the numerator.

Therefore, $\frac{13x^2 + 6x + 3}{2y}$ is the best answer.

Report an Error

Example Question #3 : Expressions

Choose the answer which best simplifies the following expression:

$\frac{2p^2 + 3p}{2a} - \frac{5p}{3}$

Possible Answers:

$\frac{6p + 9p -10pa}{6a}$

$\frac{15p -10pa}{6a}$

$\frac{6p^2 + 9p -10p}{6}$

$\frac{6p^2 + 9p -10pa}{6a}$

$\frac{6p^2 + 9p +10pa}{6a}$

Correct answer:

$\frac{6p^2 + 9p -10pa}{6a}$

Explanation:

To simplify this expression, first multiply both terms by the denominator of the other over itself:

$\frac{2p^2 + 3p}{2a}\left(\frac{3}{3}\right) - \frac{5p}{3}\left(\frac{2a}{2a}\right)$

$\frac{6p^2 + 9p}{6a} - \frac{10pa}{6a}$

Now that you have a common denominator, you may subtract:

$\frac{6p^2 +9p - 10pa}{6a}$

Report an Error

All GRE Math Resources

13 Diagnostic Tests 452 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Spanish Tutors in Chicago, Math Tutors in San Francisco-Bay Area, Algebra Tutors in Philadelphia, Statistics Tutors in Miami, ACT Tutors in Seattle, MCAT Tutors in Seattle, Reading Tutors in Atlanta, Chemistry Tutors in Denver, Computer Science Tutors in New York City, Math Tutors in Houston

Popular Courses & Classes

GMAT Courses & Classes in Chicago, LSAT Courses & Classes in Houston, Spanish Courses & Classes in Los Angeles, MCAT Courses & Classes in Philadelphia, SSAT Courses & Classes in Philadelphia, SAT Courses & Classes in Miami, ACT Courses & Classes in New York City, MCAT Courses & Classes in San Francisco-Bay Area, GRE Courses & Classes in Dallas Fort Worth, GMAT Courses & Classes in San Francisco-Bay Area

Popular Test Prep

ACT Test Prep in Boston, ISEE Test Prep in Denver, GMAT Test Prep in Miami, ACT Test Prep in Washington DC, MCAT Test Prep in Dallas Fort Worth, SAT Test Prep in Miami, GRE Test Prep in Seattle, SAT Test Prep in Houston, LSAT Test Prep in Los Angeles, GMAT Test Prep in Chicago

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

Email address:
Your name:
Feedback:

GRE Math : Rational Expressions

Study concepts, example questions & explanations for GRE Math

All GRE Math Resources

Example Questions

Example Question #911 : Algebra

Example Question #2 : How To Divide Rational Expressions

Example Question #3 : Expressions

Example Question #3 : Rational Expressions

Example Question #1 : Expressions

Example Question #3 : How To Add Rational Expressions With A Common Denominator

Example Question #4 : How To Add Rational Expressions With A Common Denominator

Example Question #5 : How To Add Rational Expressions With A Common Denominator

Example Question #281 : Algebra

Example Question #3 : Expressions

All GRE Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details