All GRE Subject Test: Math Resources
Example Questions
Example Question #1 : Order Of Convergence
For which values of p is

convergent?
only
All positive values of
it doesn't converge for any values of
only
We can solve this problem quite simply with the integral test. We know that if
converges, then our series converges.
We can rewrite the integral as
and then use our formula for the antiderivative of power functions to get that the integral equals
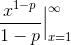
We know that this only goes to zero if 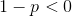

Example Question #12 : Functions
Solve the integral
using Simpson's rule with 
Simpson's rule is solved using the formula
where 

For this problem, 
The value of each approximation term is below.

The sum of all the approximation terms is 
Example Question #12 : Differential Functions
Solve the integral
using Simpson's rule with 
Simpson's rule is solved using the formula
where 

For this problem, 
The value of each approximation term is below.

The sum of all the approximation terms is 
Example Question #12 : Differential Functions
Solve the integral
using Simpson's rule with 
Simpson's rule is solved using the formula
where 

For this problem, 
The value of each approximation term is below.

The sum of all the approximation terms is 
Example Question #2 : Numerical Integration
Solve the integral
using Simpson's rule with 
Simpson's rule is solved using the formula
where 

For this problem, 
The value of each approximation term is below.

The sum of all the approximation terms is 
Example Question #1 : Numerical Approximation
Solve the integral
using the trapezoidal approximation with 
Trapezoidal approximations are solved using the formula
where 

For this problem, 
The value of each approximation term is below.

The sum of all the approximation terms is 
Example Question #11 : Differential Functions
Solve the integral
using the trapezoidal approximation with 
Trapezoidal approximations are solved using the formula
where 

For this problem, 
The value of each approximation term is below.

The sum of all the approximation terms is 
Example Question #11 : Midpoint Riemann Sums
Solve the integral
using the trapezoidal approximation with 
Trapezoidal approximations are solved using the formula
where 

For this problem, 
The value of each approximation term is below.

The sum of all the approximation terms is 
Example Question #2 : Numerical Approximation
Solve the integral
using the trapezoidal approximation with 
Trapezoidal approximations are solved using the formula
where 

For this problem, 
The value of each approximation term is below.

The sum of all the approximation terms is 
Example Question #9 : Numerical Integration
Evaluate 
1) n = 2 indicates 2 equal subdivisions. In this case, they are from 0 to 1, and from 1 to 2.
2) Trapezoidal Rule is:
3) For n = 2:
4) Simplifying:
All GRE Subject Test: Math Resources












![\int_{a}^{b}f(x))dx =S_{2m}=\frac{1}{3}(\frac{b-a}{2n})\left [ f(0)+4f(1)+2f(2)+...+2f(m-2)+4f(m-1)+f(m) \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/361760/gif.latex)
![(\frac{b-a}{2n})\left [ f(0)+2f(1)+...+f(m-1)+f(m) \right ]=(0.4)*(-8.23995)=-1.0987](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/361762/gif.latex)






![\int_{a}^{b}f(x))dx=S_{2m}=\frac{1}{3}(\frac{b-a}{2n})\left [ f(0)+4f(1)+2f(2)+...+2f(m-2)+4f(m-1)+f(m) \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/361739/gif.latex)
![(\frac{b-a}{2n})\left [ f(0)+2f(1)+...+f(m-1)+f(m) \right ]=(0.7)*(72.79378)=16.9852](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/361741/gif.latex)






![\int_{a}^{b}f(x))dx =S_{2m}=\frac{1}{3}(\frac{b-a}{2n})\left [ f(0)+4f(1)+2f(2)+...+2f(m-2)+4f(m-1)+f(m) \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/361742/gif.latex)
![(\frac{b-a}{2n})\left [ f(0)+2f(1)+...+f(m-1)+f(m) \right ]=(0.2)*(10.5840)=0.7056](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/361744/gif.latex)






![\int_{a}^{b}f(x))dx\approx S_{2m}=\frac{1}{3}(\frac{b-a}{2n})\left [ f(0)+4f(1)+2f(2)+...+2f(m-2)+4f(m-1)+f(m) \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/361736/gif.latex)
![(\frac{b-a}{2n})\left [ f(0)+2f(1)+...+f(m-1)+f(m) \right ]=(0.9)*(2.3081)=0.6924](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/361757/gif.latex)






![\int_{a}^{b}f(x))dx\approx T_{n}=(\frac{b-a}{2n})\left [ f(0)+2f(1)+...+f(m-1)+f(m) \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/369004/gif.latex)
![(\frac{b-a}{2n})\left [ f(0)+2f(1)+...+f(m-1)+f(m) \right ]=(0.5)*(0.86027754)=0.4301](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/369006/gif.latex)






![\int_{a}^{b}f(x))dx\approx T_{n}=(\frac{b-a}{2n})\left [ f(0)+2f(1)+...+f(m-1)+f(m) \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/369007/gif.latex)
![(\frac{b-a}{2n})\left [ f(0)+2f(1)+...+f(m-1)+f(m) \right ]=(0.1)*(28.786699)=2.8787](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/361699/gif.latex)






![\int_{a}^{b}f(x))dx\approx =T_{n}=(\frac{b-a}{2n})\left [ f(0)+2f(1)+...+f(m-1)+f(m) \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/361690/gif.latex)
![(\frac{b-a}{2n})\left [ f(0)+2f(1)+...+f(m-1)+f(m) \right ]=(0.4)*(83.89553)=33.5582](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/361706/gif.latex)






![\int_{a}^{b}f(x))dx\approx T_{n}=(\frac{b-a}{2n})\left [ f(0)+2f(1)+...+f(m-1)+f(m) \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/368964/gif.latex)
![(\frac{b-a}{2n})\left [ f(0)+2f(1)+...+f(m-1)+f(m) \right ]=(0.1)*(11.7257431)=1.1726](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/361709/gif.latex)





![\int_{a}^{b} f(x)dx \approx \left [ b-a\right ]\left [ \frac{f(a)+f(b)}{2}\right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/724677/gif.latex)
![\int_{0}^2 x^{x^{2}} dx \approx [1-0]\left [ \frac{f(0)+f(1)}{2} \right ]+ [2-1]\left [ \frac{f(1)+f(2)}{2} \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/724678/gif.latex)
![\int_{0}^2 x^{x^{2}} dx \approx [1]\left [ \frac{0+1}{2} \right ]+ [1]\left [ \frac{1+16}{2} \right ] = \frac{1}{2}+\frac{17}{2} = \frac{18}{2} = 9.](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/724679/gif.latex)



