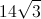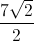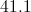We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

High School Math : Triangles

Study concepts, example questions & explanations for High School Math

Create An Account

All High School Math Resources

8 Diagnostic Tests 613 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Applying Trigonometric Functions

Rt_triangle_letters In this figure, side X=20 , Y=13 , and $c=90^\circ$ . What is the value of angle ?

Possible Answers:

$49.46^\circ$

$90^\circ$

$6.5^\circ$

$40.54^\circ$

Undefined

Correct answer:

$49.46^\circ$

Explanation:

Since $c=90^\circ$ , we know we are working with a right triangle.

That means that $\cos(\theta)=\frac{\text{adjacent}}{\text{hypotenuse}}$ .

In this problem, that would be:

$\cos(a)=\frac{\text{Y}}{\text{X}}$

Plug in our given values:

$\cos(a)=\frac{\text{13}}{\text{20}}$

$\cos(a)=0.65$

$a=\cos^{-1}(0.65)$

$a=49.46^\circ$

Report an Error

Example Question #1 : Triangles

Let ABC be a right triangle with sides = 3 inches, = 4 inches, and = 5 inches. In degrees, what is the $\sin \Theta$ where $\Theta$ is the angle opposite of side ?

Possible Answers:

Correct answer:

Explanation:

3-4-5_triangle

We are looking for $\sin(\theta )$ . Remember the definition of sin in a right triangle is the length of the opposite side divided by the length of the hypotenuse.

So therefore, without figuring out $\Theta$ we can find

$\sin \Theta =\frac{3}{5}=.6$

Report an Error

Example Question #1 : Triangles

Rt_triangle_letters

In this figure, if angle $c=90^\circ$ , side X=12 , and side Z=8 , what is the measure of angle ?

Possible Answers:

$\frac{2}{3}^\circ$

$41.8^\circ$

Undefined

$48.2^\circ$

$90^\circ$

Correct answer:

$41.8^\circ$

Explanation:

Since $c=90^\circ$ , we know we are working with a right triangle.

That means that $\sin(\theta)=\frac{\text{opposite}}{\text{hypotenuse}}$ .

In this problem, that would be:

$\sin(a)=\frac{\text{Z}}{\text{X}}$

Plug in our given values:

$\sin(a)=\frac{8}{12}$

$\sin(a)=\frac{2}{3}$

$a=\sin^{-1}(\frac{2}{3})$

$a=41.8^\circ$

Report an Error

Example Question #1 : Understanding 30 60 90 Triangles

Rt_triangle_letters

In this figure, X=12 , $Y=6\sqrt{3}$ , and Z=6 . What is the value of angle ?

Possible Answers:

Undefined

$45^\circ$

$90^\circ$

$30^\circ$

$60^\circ$

Correct answer:

$30^\circ$

Explanation:

Notice that these sides fit the pattern of a 30:60:90 right triangle: $x:x\sqrt{3}:2x$ .

In this case, x=6 .

Since angle is opposite , it must be $30^\circ$ .

Report an Error

Example Question #2 : Understanding 30 60 90 Triangles

A triangle has angles of $30^\circ:60^\circ:90^\circ$ . If the side opposite the $30^\circ$ angle is , what is the length of the side opposite $60^\circ$ ?

Possible Answers:

$7\sqrt{2}$

$7\sqrt{3}$

$\frac{7\sqrt{2}}{2}$

$14\sqrt{3}$

Correct answer:

$7\sqrt{3}$

Explanation:

The pattern for $30^\circ:60^\circ:90^\circ$ is that the sides will be $x:x\sqrt{3}:2x$ .

If the side opposite $30^\circ$ is , then the side opposite $60^\circ$ will be $7\sqrt{3}$ .

Report an Error

Example Question #1 : Applying The Law Of Cosines

In $\Delta ABC$ , AB = 26 , BC = 32 , and AC = 23 . To the nearest tenth, what is $m \angle A$ ?

Possible Answers:

$171.3^{ \circ }$

$98.7^{ \circ }$

$81.3 ^{\circ }\textrm{ or } 98.7^{ \circ }$

A triangle with these sidelengths cannot exist.

$81.3 ^{\circ }$

Correct answer:

$81.3 ^{\circ }$

Explanation:

By the Triangle Inequality, this triangle can exist, since 23 + 26 > 32 .

By the Law of Cosines:

$\left ( BC\right )^{2} = \left ( AB\right )^{2} + \left ( AC\right )^{2} - 2 \cdot AB \cdot AC \cdot \cos m \angle A$

Substitute the sidelengths and solve for $\cos m \angle A$ :

$32^{2} = 26^{2} + 23^{2} - 2\cdot 26 \cdot 23 \cdot \cos m \angle A$

$1,024 =676 + 529 - 1,196 \cdot \cos m \angle A$

$1,024 =1,205 - 1,196 \cdot \cos m \angle A$

$-181 = - 1,196 \cdot \cos m \angle A$

$\cos m \angle A = 181 \div 1,196 \approx 0.1513$

$m \angle A \approx \cos^{-1} 0.1513 \approx 81.3 ^{\circ }$

Report an Error

Example Question #1 : Law Of Cosines

A triangle has sides of length 12, 17, and 22. Of the measures of the three interior angles, which is the greatest of the three?

Possible Answers:

$97.2^{\circ }$

$130.1^{\circ }$

$93.6^{\circ }$

$86.4^{\circ }$

$119.2^{\circ }$

Correct answer:

$97.2^{\circ }$

Explanation:

We can apply the Law of Cosines to find the measure of this angle, which we will call :

$c^{2} = a^{2} + b^{2} -2ab \cos C$

The widest angle will be opposite the side of length 22, so we will set:

c=22 , a=12 , b=17

$22^{2} = 12^{2} + 17^{2} -2\cdot 12\cdot 17 \cos C$

$484 = 144 + 289 -408 \cos C$

$51= -408 \cos C$

$\cos C = -\frac{51}{408} = -0.125$

$C = \cos^{-1} 0.125 = 97.2^{\circ }$

Report an Error

Example Question #2 : Law Of Cosines

In $\Delta ABC$ , $m \angle A = 66^{\circ }$ , AB = 26 , and AC = 23 . To the nearest tenth, what is ?

Possible Answers:

A triangle with these characteristics cannot exist.

112.4

41.1

47.9

26.8

Correct answer:

26.8

Explanation:

By the Law of Cosines:

$\left ( BC\right )^{2} = \left ( AB\right )^{2} + \left ( AC\right )^{2} - 2 \cdot AB \cdot AC \cdot \cos m \angle A$

or, equivalently,

$BC =\sqrt{ \left ( AB\right )^{2} + \left ( AC\right )^{2} - 2 \cdot AB \cdot AC \cdot \cos m \angle A}$

Substitute:

$BC =\sqrt{ 26^{2} +23^{2} - 2 \cdot 26 \cdot 23 \cdot \cos 66 ^{\circ }}$

$\approx \sqrt{ 676 +529 - 1,196 \cdot 0.4067}$

$\approx \sqrt{ 718.6} \approx 26.8$

Report an Error

Example Question #1 : Applying The Law Of Sines

Rt_triangle_letters
In this figure, angle $a=30^\circ$ and side Z=15 . If angle $b=45^\circ$ , what is the length of side ?

Possible Answers:

$30\sqrt{2}$

7.5

$\frac{15\sqrt{2}}{2}$

$15\sqrt{2}$

Correct answer:

$15\sqrt{2}$

Explanation:

For this problem, use the law of sines:

$\frac{\text{side opposite }a}{\sin(a)}=\frac{\text{side opposite }b}{\sin(b)}=\frac{\text{side opposite }c}{\sin(c)}$ .

In this case, we have values that we can plug in:

$\frac{\text{side opposite }a}{\sin(a)}=\frac{\text{side opposite }b}{\sin(b)}$

$\frac{Z}{\sin(a)}=\frac{Y}{\sin{(b)}}$

$\frac{15}{\sin(30^\circ)}=\frac{Y}{\sin{(45^\circ)}}$

$\frac{15}{\frac{1}{2}}=\frac{Y}{\frac{\sqrt{2}}{2}}$

Cross multiply:

$15*\frac{\sqrt{2}}{2}=\frac{1}{2}Y$

Multiply both sides by :

$15\sqrt{2}=Y$

Report an Error

Example Question #2 : Applying The Law Of Sines

Rt_triangle_letters

In this figure $a=22^\circ$ and $c=85^\circ$ . If X=30 , what is ?

Possible Answers:

30.09

11.28

20.1

0.374

0.997

Correct answer:

11.28

Explanation:

For this problem, use the law of sines:

$\frac{\text{side opposite }a}{\sin(a)}=\frac{\text{side opposite }b}{\sin(b)}=\frac{\text{side opposite }c}{\sin(c)}$ .

In this case, we have values that we can plug in:

$\frac{\text{side opposite }a}{\sin(a)}=\frac{\text{side opposite }c}{\sin(c)}$

$\frac{Z}{\sin(a)}=\frac{X}{\sin{(c)}}$

$\frac{Z}{\sin(22^\circ)}=\frac{30}{\sin{85^\circ}}$

$\frac{Z}{0.375}=\frac{30}{0.997}$

$\frac{Z}{0.375}=30.09$

Z=30.09*0.375

Z=11.28

Report an Error

View Tutors

Bhavika
Certified Tutor

Stony Brook University, Bachelor of Science, Biology, General.

View Tutors

Caroline
Certified Tutor

University of Florida, Master's/Graduate, Religion.

View Tutors

Laura
Certified Tutor

Indiana University-Bloomington, Bachelor in Arts, Italian.

All High School Math Resources

8 Diagnostic Tests 613 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Physics Tutors in Washington DC, Math Tutors in San Francisco-Bay Area, Statistics Tutors in Miami, Physics Tutors in Houston, Algebra Tutors in Phoenix, Math Tutors in Dallas Fort Worth, Chemistry Tutors in Chicago, Chemistry Tutors in Washington DC, Spanish Tutors in San Francisco-Bay Area, French Tutors in Atlanta

Popular Courses & Classes

GMAT Courses & Classes in Boston, SSAT Courses & Classes in Los Angeles, MCAT Courses & Classes in Seattle, Spanish Courses & Classes in Miami, GMAT Courses & Classes in Seattle, MCAT Courses & Classes in Dallas Fort Worth, ISEE Courses & Classes in Phoenix, LSAT Courses & Classes in Dallas Fort Worth, ACT Courses & Classes in Washington DC, LSAT Courses & Classes in Denver

Popular Test Prep

GMAT Test Prep in San Diego, SAT Test Prep in Seattle, MCAT Test Prep in Chicago, GRE Test Prep in Philadelphia, LSAT Test Prep in Houston, GRE Test Prep in San Diego, SAT Test Prep in New York City, LSAT Test Prep in New York City, MCAT Test Prep in Philadelphia, GRE Test Prep in Atlanta

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

High School Math : Triangles

Study concepts, example questions & explanations for High School Math

All High School Math Resources

Example Questions

Example Question #1 : Applying Trigonometric Functions

Example Question #1 : Triangles

Example Question #1 : Triangles

Example Question #1 : Understanding 30 60 90 Triangles

Example Question #2 : Understanding 30 60 90 Triangles

Example Question #1 : Applying The Law Of Cosines

Example Question #1 : Law Of Cosines

Example Question #2 : Law Of Cosines

Example Question #1 : Applying The Law Of Sines

Example Question #2 : Applying The Law Of Sines

All High School Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: