All Intermediate Geometry Resources
Example Questions
Example Question #1 : How To Find The Length Of The Side Of A Trapezoid
Suppose the area of the trapezoid is 


Write the formula for finding the area of a trapezoid.
Substitute the givens and solve for either base.
Example Question #1 : How To Find The Length Of The Side Of A Trapezoid
If the area of a trapezoid is 


Write the formula for the area of a trapezoid.
Substitute all the given values and solve for the base.
Example Question #3 : How To Find The Length Of The Side Of A Trapezoid
An isosceles trapezoid has base measurements of 


To solve this problem, first note that an isosceles trapezoid has two parallel bases that are nonequivalent in length. Additionally, an isosceles trapezoid must have two nonparallel sides that have equivalent lengths. Since this problem provides the length for both of the bases as well as the total perimeter, the missing sides can be found using the following formula: Perimeter= Base one 


Thus, the solution is: 
Check the solution by plugging in the answer:
Example Question #4 : How To Find The Length Of The Side Of A Trapezoid
An isosceles trapezoid has base measurements of 


In order to solve this problem, first note that an isosceles trapezoid has two parallel bases that are nonequivalent in length. Additionally, an isosceles trapezoid must have two nonparallel sides that have equivalent lengths.
This problem provides the lengths for each of the bases as well as the height of the isosceles trapezoid. In order to find the length for one of the two equivalent nonparallel legs of the trapezoid, first use the height of the trapezoid to form right triangles on the interior of the trapezoid that each have a base length of 

Note: the base length of 
Now, apply the formula 

Thus, the solution is:
Example Question #5 : How To Find The Length Of The Side Of A Trapezoid
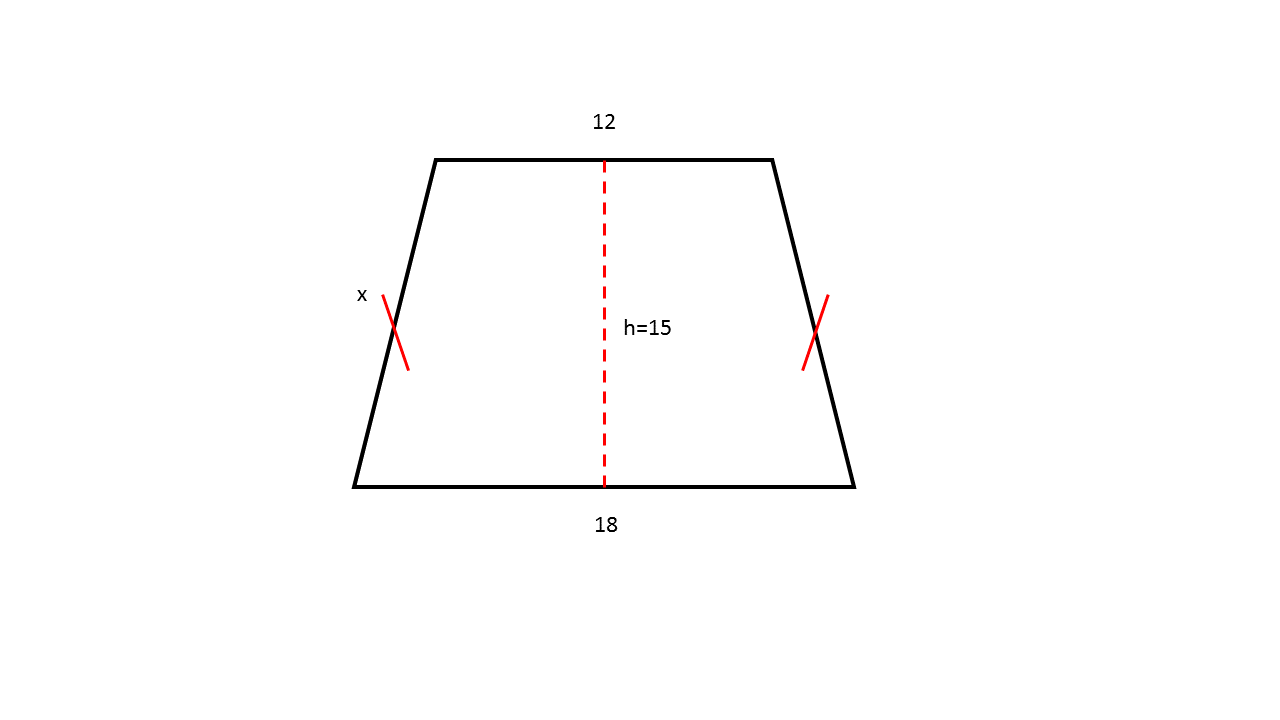
The isosceles trapezoid shown above has base measurements of 



In this problem the lengths for each of the bases and the height of the isosceles trapezoid is provided in the question prompt. In order to find the length for one of the two equivalent nonparallel legs of the trapezoid (side 

The base of the interior triangles is equal to 

Now, apply the pythagorean theorem: 

Thus,
Example Question #6 : How To Find The Length Of The Side Of A Trapezoid

Using the isosceles trapezoid shown above, find the length for one of the two nonparallel equivalent sides.
To solve this problem, first note that an isosceles trapezoid has two parallel bases that are nonequivalent in length. Additionally, an isosceles trapezoid must have two nonparallel sides that have equivalent lengths. Since this problem provides the length for both of the bases as well as the total perimeter, the missing sides can be found using the following formula: Perimeter= Base one 


Thus, the solution is:
Example Question #7 : How To Find The Length Of The Side Of A Trapezoid
An isosceles trapezoid has one base measurement of 


Not enough information is provided in this problem.
To solve this problem, first note that an isosceles trapezoid has two parallel bases that are nonequivalent in length. Additionally, an isosceles trapezoid must have two nonparallel sides that have equivalent lengths.
Therefore, use the given information to apply the formula:
Perimeter= Base one 


Thus, the solution is:
Example Question #8 : How To Find The Length Of The Side Of A Trapezoid
An isosceles trapezoid has base measurements of 


In order to solve this problem, first note that an isosceles trapezoid has two parallel bases that are nonequivalent in length. Additionally, an isosceles trapezoid must have two nonparallel sides that have equivalent lengths.
This problem provides the lengths for each of the bases as well as informataion regarding the height of the isosceles trapezoid. In order to find the length for one of the two equivalent nonparallel legs of the trapezoid, use the height of the trapezoid to form right triangles on the interior of the trapezoid that each have a base length of 

In order to calculate the exact height of the isosceles trapezoid (as well as the interior triangle), find 

Now you have enough information to apply the formula 

The final solution is:

Example Question #9 : How To Find The Length Of The Side Of A Trapezoid
An isosceles trapezoid has base measurements of 


To solve this problem, first note that an isosceles trapezoid has two parallel bases that are nonequivalent in length. Additionally, an isosceles trapezoid must have two nonparallel sides that have equivalent lengths. Since this problem provides the length for both of the bases as well as the total perimeter, the missing sides can be found using the following formula: Perimeter= Base one 


Thus, the solution is:
Check the solution by plugging in the answer:
Example Question #10 : How To Find The Length Of The Side Of A Trapezoid
An isosceles trapezoid has one base measurement of 


To solve this problem, first note that an isosceles trapezoid has two parallel bases that are nonequivalent in length. Additionally, an isosceles trapezoid must have two nonparallel sides that have equivalent lengths.
Therefore, use the given information to apply the formula:
Perimeter= Base one 


Thus, the solution is:
All Intermediate Geometry Resources






































































































