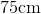Divide the perimeter 16 feet by 6 to get the sidelength of the hexagon in feet:

\(\displaystyle 16 \div 6 = 2\frac{2}{3} \textrm{ ft }\)

Two-thirds of a foot is equal to eight inches, so this sidelength is 2 feet 8 inches.

One mile is equal to 5,280 feet, so divide this by 6:

\(\displaystyle 5,280 \div 6 = 880\) feet

A hexagon has 6 equal sides.  The formula to find perimeter of a hexagon is:

\(\displaystyle P = 6a\)

where a is the length of any side.  Now, to find the length of one side, we will solve for a. 

We know the perimeter of the hexagon is 114in.  So, we will substitute and solve for a.  We get

\(\displaystyle 114\text{in} = 6a\)

\(\displaystyle \frac{114\text{in}}{6} = \frac{6a}{6}\)

\(\displaystyle 19\text{in} = a\)

\(\displaystyle a = 19\text{in}\)

Therefore, the length of one side of the hexagon is 19in.

A hexagon has 6 equal sides. To find the length of one side of a hexagon, we will use the formula to find the perimeter.  

\(\displaystyle P = 6a\)

where a is the length of one side. So, we will solve for a.

Now, we know the perimeter of the hexagon is 96cm. So, we get

\(\displaystyle 96\text{cm} = 6a\) 

\(\displaystyle \frac{96\text{cm}}{6} = \frac{6a}{6}\)

\(\displaystyle 16\text{cm} = a\)

\(\displaystyle a = 16\text{cm}\)

Therefore, the length of one side of the hexagon is 16cm.

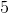
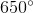
The sum of the angles in a polygon can be found using the equation below, in which t is equal to the total sum of the angles, and n is equal to the number of sides. 

\(\displaystyle t=(n-2)(180)\)

Given that a hexagon has 6 angles, the total number of angles will be:

\(\displaystyle t=(6-2)(180)\)

\(\displaystyle t=4\cdot 180\)

\(\displaystyle t=720\)

Given that 5 of the angles have a sum of 650 degrees, we would subtract 650 from 720, resulting in 70 degrees. 

To find the perimeter of a hexagon, we will use the following formula:

\(\displaystyle P = 6 \cdot a\)

where a is the length of one side of the hexagon.

Now, we know the length of one side of the hexagon is 15cm.

Knowing this, we can substitute into the formula.  We get

\(\displaystyle P = 6 \cdot 15\text{cm}\)

\(\displaystyle P = 90\text{cm}\)

A hexagon has 6 sides.  So, to find the perimeter, we will use the following formula:

\(\displaystyle P = 6a\)

where a is the length of one side.

Now, we know a side has a length of 13cm.  Knowing this, we can substitute into the formula. 

\(\displaystyle P = 6 \cdot 13\text{cm}\)

\(\displaystyle P = 78\text{cm}\)

To find the perimeter of a hexagon, we will use the following formula:

\(\displaystyle P = 6a\)

where a is the length of one side of the hexagon.  We multiply it by 6 because a hexagon has 6 equal sides.

So, we know the length of one side is 13cm.  Knowing this, we can substitute into the formula.  We get

\(\displaystyle P = 6 \cdot 13\text{in}\)

\(\displaystyle P = 78\text{in}\)

To find the perimeter of a hexagon, we will use the following formula:

\(\displaystyle P = 6 \cdot a\)

where a is the length of one side of the hexagon.  Because a hexagon has 6 equal sides, we can use any side in the formula.

So, we know the length of one side is 12in.  So, we can substitute.  We get

\(\displaystyle P = 6 \cdot 12\text{in}\)

\(\displaystyle P = 72\text{in}\)

To find the perimeter of a hexagon, we will use the following formula:

\(\displaystyle P = 6 \cdot a\)

where a is the length of one side of the hexagon.

Now, we know the length of one side of the hexagon is 17cm.

Knowing this, we can substitute into the formula.  We get

\(\displaystyle P = 6 \cdot 17\text{cm}\)

\(\displaystyle P = 102\text{cm}\)