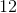Call Now to Set Up Tutoring:

(888) 888-0446

PSAT Math : How to find the area of a polygon

Study concepts, example questions & explanations for PSAT Math

Create An Account

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #12 : Other Polygons

A square has an area of 36 cm². A circle is inscribed and cut out. What is the area of the remaining shape? Use 3.14 to approximate π.

Possible Answers:

7.74 cm²

15.48 cm²

3.69 cm²

12.14 cm²

28.26 cm²

Correct answer:

7.74 cm²

Explanation:

We need to find the area of both the square and the circle and then subtract the two. Inscribed means draw within a figure so as to touch in as many places as possible. So the circle is drawn inside the square. The opposite is circumscribed, meaning drawn outside.

A_square = s² = 36 cm² so the side is 6 cm

6 cm is also the diameter of the circle and thus the radius is 3 cm

A circle = πr² = 3.14 * 3² = 28.28 cm²

The resulting difference is 7.74 cm²

Report an Error

Example Question #1 : How To Find The Area Of A Polygon

Gre10

In the square above, the radius of each half-circle is 6 inches. What is the area of the shaded region?

Possible Answers:

144 – 36π

144 – 6π

36 – 6π

36 – 9π

144 – 9π

Correct answer:

144 – 36π

Explanation:

We can find the area of the shaded region by subtracting the area of the semicircles, which is much easier to find. Two semi-circles are equivalent to one full circle. Thus we can just use the area formula, where r = 6:

π(6²) → 36π

Now we must subtract the area of the semi-circles from the total area of the square. Since we know that the radius also covers half of a side, 6(2) = 12 is the full length of a side of the square. Squaring this, 12² = 144. Subtracting the area of the circles, we get our final terms,

= 144 – 36π

Report an Error

Example Question #1 : How To Find The Area Of A Polygon

If square A has a side of length 5 inches, how many times bigger is the area of square B if it has a side of length 25 inches?

Possible Answers:

25 times

625 times

5 times

4 times

2 times

Correct answer:

25 times

Explanation:

First find the area of both squares using the formula A=s^2 .

For square A, s = 5.

A_A=5^2=25

For square B, s = 25.

A_B=25^2=625

The question is asking for the ratio of these two areas, which will tell us how many times bigger square B is. Divide the area of square B by the area of square A to find the answer.

$\frac{A_B}{A_A}=\frac{625}{25}=25$

Report an Error

Example Question #2 : How To Find The Area Of A Polygon

Sat_math_picture3

If Bailey paints the wall shaped like above and uses one bucket per 5 square units, how many buckets does Bailey need?

Possible Answers:

Correct answer:

Explanation:

To solve, we will need to find the area of the wall. We can do this by finding the areas of each section and adding them together. Break the area into a rectange and two triangles.

Sat_math_picture3

The area of the rectangle will be equal to the base times the height. The area of each triangle will be one half its given base times its height.

$A_{rec}=bh$

$A_{tri}=\frac{1}{2}bh$

For the rectangle, the base is 12 and the height is 4 (both given in the figure).

$A_{rec}=(12)(4)=48$

The triangle to the right has a given base of 6, but we need to solve for its height. The height will be equal to the difference between the total height (6) and the height of the rectangle (4).

$h_{tri}=6-4=2$

We now have the base and height of the triangle to the right, allowing us to calculate its area.

$A_{tri_r}=\frac{1}{2}(6)(2)=6$

Now we need to solve the triangle to the left. We solved for its height (2), but we still need to solve for its base. The total base of the rectangle is 12. Subtract the base of the right-side triangle (6) and the small segment at the top of the rectangle (3) from this total length to solve for the base of the left triangle.

12-6-3=3

The left-side triangle has a base of 3 and a height of 2, allowing us to calculate its area.

$A_{tri_l}=\frac{1}{2}(3)(2)=3$

Add together the two triangles and the rectangle to find the total area.

A=48+6+3=57

We know that each bucket of paint will cover 5 square units, and we have 57 square units total. Divide to find how many buckets are required.

$\frac{57}{5}=11.4$

We will need 11 full buckets and part of a twelfth bucket to cover the wall, meaning that we will need 12 buckets total.

Report an Error

Example Question #3 : How To Find The Area Of A Polygon

A square is inscribed within a circle with a radius $\small 3\sqrt{2}$ . Find the area of the circle that is not covered by the square.

Possible Answers:

$\small 9\pi-6$

$9\pi-3\sqrt{2}$

$\small 9\pi-36$

$\small 18\pi-6$

$\small 18\pi-36$

Correct answer:

$\small 18\pi-36$

Explanation:

First, find the area of the circle.

$A=\pi(3\sqrt{2})^2$

$\small A=18\pi$

Next, find the length of 1 side of the square using the Pythagorean Theorem. Two radii from the center of the circle to adjacent corners of the square will create a right angle at the center of the circle. The radii will be the legs of the triangle and the side of the square will be the hypotenuse.

$\small (3\sqrt{2})^2 +(3\sqrt{2})^2 =c^2$

$\small 18+18=36=c^2$

$\small c=6$

Find the area of the square.

$\small A=c^2=6^2=36$

Subtract the area of the square from the area of the circle.

$\small 18\pi-36$

Report an Error

Example Question #4 : How To Find The Area Of A Polygon

Thingy

Refer to the above figure. Quadrilateral BCDE is a square. Give the area of Polygon ABCDE in terms of .

Possible Answers:

$6x^{2}$

$7x^{2}$

$\left ( 2+ \sqrt{5}\right )x^{2}$

$\left ( 1+ \sqrt{5}\right )x^{2}$

$5x^{2}$

Correct answer:

$6x^{2}$

Explanation:

$\overline{BE }$ is both one side of Square BCDE and the hypotenuse of $\Delta ABE$ ; its hypotenuse can be calculated from the lengths of the legs using the Pythagorean Theorem:

$BE = \sqrt{(AB)^{2}+ \left ( AE\right )^{2}}$

$BE = \sqrt{x^{2}+ \left ( 2x\right )^{2}} = \sqrt{x^{2}+ 4x ^{2}} = \sqrt{5x ^{2}} = x\sqrt{5}$

Polygon ABCDE is the composite of $\Delta ABE$ and Square BCDE , so its area is the sum of those of the two figures.

The area of $\Delta ABE$ is half the product of its legs:

$A= \frac{1}{2} \cdot x \cdot 2x = x^{2}$

The area of Square BCDE is the square of the length of a side:

$A = \left ( x\sqrt{5} \right )^{2} = 5x^{2}$

Add the areas:

$x^{2}+ 5x^{2} = 6x^{2}$

This is the area of the polygon.

Report an Error

Example Question #7 : Other Polygons

Stuff_1

Note: Figure NOT drawn to scale.

The above figure shows Rectangle RCAN ; is the midpoint of $\overline{CA}$ ; $EC = 2 \cdot RE$ ; $RC = 2 \cdot RN$ .

What percent of Rectangle RCAN is shaded?

Possible Answers:

$72 \%$

$80 \%$

$60 \%$

$66 \frac{2}{3} \%$

$75 \%$

Correct answer:

$66 \frac{2}{3} \%$

Explanation:

The area of ETAN , the shaded region in question, is that of the rectangle minus those of $\Delta ERN$ and $\Delta ECT$ . We look at both.

The answer is independent of the sidelengths of the rectangle, so to ease calculations - this will become more apparent later - we will arbitrarily assign to the rectangle the dimensions

RN = CA = 6

and, subsequently,

RC = NA = 12

and, since is the midpoint of $\overline{CA}$ ,

CT = 3 .

The area of Rectangle RCAN is equal to $RN \cdot RC = 6 \cdot 12 = 72$ .

Since $EC = 2 \cdot RE$ , and RE + EC = RC ,

RE + 2 (RE)= 12

3 (RE)= 12

RE = 4 and EC = 8

The area of $\Delta ERN$ is equal to $\frac{1}{2}(RE)(RN)= \frac{1}{2} \cdot 4 \cdot 6 = 12$ .

The area of $\Delta ECT$ is equal to $\frac{1}{2}(EC)(CT)= \frac{1}{2} \cdot 8 \cdot 3 = 12$

The area of the shaded region is therefore 72-12-12 = 48 , which is

$\frac{48}{72} \times 100 \% = 66 \frac{2}{3} \%$ of the rectangle.

Report an Error

View PSAT Mathematics Tutors

Leonard
Certified Tutor

Florida State University, Bachelor of Science, Environmental Science.

View PSAT Mathematics Tutors

Paul
Certified Tutor

University of Phoenix-Albuquerque Campus, Bachelor of Science, Computer and Information Systems Security.

View PSAT Mathematics Tutors

Liban
Certified Tutor

Emory University, Bachelor of Science, Mathematics/Economics.

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Biology Tutors in San Francisco-Bay Area, Math Tutors in Denver, Physics Tutors in San Diego, French Tutors in San Diego, ISEE Tutors in Denver, Math Tutors in Philadelphia, Chemistry Tutors in Atlanta, ACT Tutors in Boston, ACT Tutors in Seattle, Chemistry Tutors in Houston

Popular Courses & Classes

GRE Courses & Classes in Chicago, SSAT Courses & Classes in Chicago, SAT Courses & Classes in Dallas Fort Worth, ACT Courses & Classes in Philadelphia, GRE Courses & Classes in Atlanta, MCAT Courses & Classes in Chicago, ACT Courses & Classes in Atlanta, ISEE Courses & Classes in Denver, ACT Courses & Classes in Phoenix, SSAT Courses & Classes in Dallas Fort Worth

Popular Test Prep

ISEE Test Prep in Washington DC, ACT Test Prep in Phoenix, SSAT Test Prep in Seattle, LSAT Test Prep in Denver, GRE Test Prep in Dallas Fort Worth, ISEE Test Prep in Miami, MCAT Test Prep in Seattle, ISEE Test Prep in Denver, GRE Test Prep in Atlanta, LSAT Test Prep in Miami

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

PSAT Math : How to find the area of a polygon

Study concepts, example questions & explanations for PSAT Math

All PSAT Math Resources

Example Questions

Example Question #12 : Other Polygons

Example Question #1 : How To Find The Area Of A Polygon

Example Question #1 : How To Find The Area Of A Polygon

Example Question #2 : How To Find The Area Of A Polygon

Example Question #3 : How To Find The Area Of A Polygon

Example Question #4 : How To Find The Area Of A Polygon

Example Question #7 : Other Polygons

All PSAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: