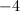All SAT Math Resources
Example Questions
Example Question #1 : How To Find Out When An Equation Has No Solution
Find the solution to the following equation if x = 3:
y = (4x2 - 2)/(9 - x2)
no possible solution
0
6
3
no possible solution
Substituting 3 in for x, you will get 0 in the denominator of the fraction. It is not possible to have 0 be the denominator for a fraction so there is no possible solution to this equation.
Example Question #2 : How To Find Out When An Equation Has No Solution
I. x = 0
II. x = –1
III. x = 1
II only
III only
I only
II and III only
I, II, and III
I only
Example Question #3 : Equations / Inequalities
3
–3
1
–1/2
There is no solution
There is no solution
Example Question #3 : How To Find Out When An Equation Has No Solution

None of the other answers
A fraction is considered undefined when the denominator equals 0. Set the denominator equal to zero and solve for the variable.
Example Question #2 : How To Find Out When An Equation Has No Solution
Solve:
First, distribute, making sure to watch for negatives.
Combine like terms.
Subtract 7x from both sides.
Add 18 on both sides and be careful adding integers.
Example Question #3 : Equations / Inequalities
Solve:
No Solution
Infinitely Many Solutions
No Solution
First, distribute the 
Add 6x to both sides.
This is false for any value of 
Example Question #1 : Linear / Rational / Variable Equations
Solve 
No solutions
No solutions
By definition, the absolute value of an expression can never be less than 0. Therefore, there are no solutions to the above expression.
Example Question #111 : Equations / Inequalities
, 
In the above graphic, approximately determine the x values where the graph is neither increasing or decreasing.
We need to find where the graph's slope is approximately zero. There is a straight line between the x values of 


Certified Tutor
All SAT Math Resources