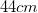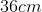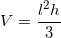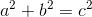Call Now to Set Up Tutoring:

(888) 888-0446

ACT Math : How to find the length of an edge of a pyramid

Study concepts, example questions & explanations for ACT Math

Create An Account

All ACT Math Resources

14 Diagnostic Tests 767 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Pyramids

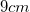
A square pyramid has a volume of 363cm^3 and a height of $\small 9cm$ . What is the perimeter of the base of the pyramid?

Possible Answers:

36cm

44cm

80cm

100cm

121cm

Correct answer:

44cm

Explanation:

The formula for the volume of a pyramid is:

$\small V=\frac{lwh}{3}$

We know that V=363 and h=9 ; however, this still leaves us with two variables in the equation: and . By definition, a square pyramid's base has sides of equal length, meaning that and are the same. Therefore, we can substitute $\small lw$ for $\small l\cdot l$ , or $\small l^2$ .

This gives us a new equation of:

$\small V=\frac{l^2h}{3}$

We then plug in the variables we know:

$\small 363=\frac{9l^2}{3}$

Multiply both sides by 3:

$\small 1089=9l^2$

Divide both sides by 9:

l^2=121

l=11

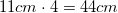
Therefore, we now know that the length of the pyramid's base is $\small 11cm$ . The question, however, asks for the perimeter of the pyramid's base. Since all of the sides of the base are the same, they must all be $\small 11cm$ . So we multiply $11cm\cdot 4=44cm$ . Therefore, the perimeter of the pyramid's base is $\small 44cm$ .

Report an Error

Example Question #2 : How To Find The Length Of An Edge Of A Pyramid

A square pyramid has a slant height of 8. The edge of a face is 12. What is the measure of an edge of its base?

Possible Answers:

15.6

8.3

8.9

9.0

17.8

Correct answer:

17.8

Explanation:

In this kind of a problem, it's helpful to draw out all information that's given.

Pyramid

Because the triangular faces of a pyramid are isosceles triangles, the slant height can be seen to divide it into two right triangles. The goal is to find the measure of the edge of the square base. This quickly becomes a problem revolving around the use of the Pythagorean theorem.

If we take the measures 12 and 8 to be the hypotenuse and one of the legs, respectively, we can use the Pythagoream theorem to solve for the "base leg." Then, realizing that this measure if only half of the the total length of the square base edge, that value must be multiplied by 2.

a^2+b^2=c^2

a^2+8^2=12^2

a^2+64=144

a^2=80

$a=\sqrt{80}$

$a={\color{Green} 8.9}$

Therefore, 2a=17.8

Report an Error

View ACT Math Tutors

Satweka
Certified Tutor

The University of Texas at Dallas, Master of Science, Biomedical Engineering.

View ACT Math Tutors

Leonard
Certified Tutor

Florida State University, Bachelor of Science, Environmental Science.

View ACT Math Tutors

Abe
Certified Tutor

CUNY Brooklyn College, Bachelor of Science, Accounting and Business Management. CUNY Graduate School and University Center, M...

All ACT Math Resources

14 Diagnostic Tests 767 Practice Tests Question of the Day Flashcards Learn by Concept

ACT Math Tutors in Top Cities:

Atlanta ACT Math Tutors, Austin ACT Math Tutors, Boston ACT Math Tutors, Chicago ACT Math Tutors, Dallas Fort Worth ACT Math Tutors, Denver ACT Math Tutors, Houston ACT Math Tutors, Kansas City ACT Math Tutors, Los Angeles ACT Math Tutors, Miami ACT Math Tutors, New York City ACT Math Tutors, Philadelphia ACT Math Tutors, Phoenix ACT Math Tutors, San Diego ACT Math Tutors, San Francisco-Bay Area ACT Math Tutors, Seattle ACT Math Tutors, St. Louis ACT Math Tutors, Tucson ACT Math Tutors, Washington DC ACT Math Tutors

Popular Courses & Classes

SSAT Courses & Classes in San Diego, GMAT Courses & Classes in Miami, Spanish Courses & Classes in Houston, Spanish Courses & Classes in Philadelphia, Spanish Courses & Classes in Washington DC, MCAT Courses & Classes in Philadelphia, ISEE Courses & Classes in Phoenix, Spanish Courses & Classes in New York City, ISEE Courses & Classes in San Diego, GMAT Courses & Classes in Dallas Fort Worth

Popular Test Prep

MCAT Test Prep in San Diego, SAT Test Prep in Philadelphia, GRE Test Prep in New York City, GMAT Test Prep in New York City, ISEE Test Prep in Chicago, SAT Test Prep in San Francisco-Bay Area, GRE Test Prep in Dallas Fort Worth, LSAT Test Prep in Dallas Fort Worth, MCAT Test Prep in Chicago, ISEE Test Prep in Philadelphia

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

ACT Math : How to find the length of an edge of a pyramid

Study concepts, example questions & explanations for ACT Math

All ACT Math Resources

Example Questions

Example Question #1 : Pyramids

Example Question #2 : How To Find The Length Of An Edge Of A Pyramid

All ACT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: