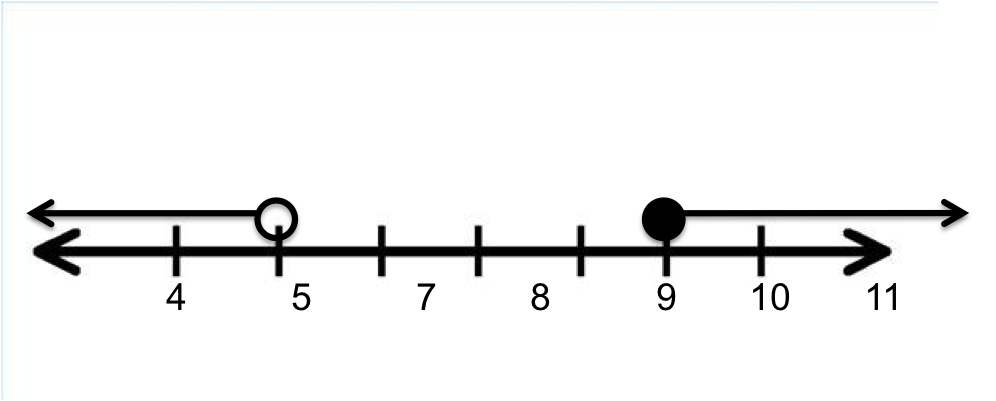
The graph shows an arrow beginning on 5 with an open circle and pointing to the left, thus that portion of the graph says, all real numbers less than 5. There is a second arrow beginning on 9 with a closed circle and pointing to the right, representing all real numbers greater than or equal to 9. Since we are joining the two parts of the graph, we have a compound inequality utilizing the "or" statement. So our answer is $\displaystyle \small x< 5 \quad or \quad x \ge 9$.   

"The product of seven and a number " is $\displaystyle 7x$. "Twenty subtracted from the product of seven and a number" is $\displaystyle 7x - 20$ . "Exceeds one hundred" means that this is greater than one hundred, so the correct inequality is

$\displaystyle 7x - 20 > 100$

"The sum of a number and sixteen" is translates to $\displaystyle x + 16$; twice that sum is $\displaystyle 2(x+16)$. " Is no less than sixty" means that this is greater than or equal to sixty, so the desired inequality is

 $\displaystyle 2(x+16) \geq 60$.

"The sum of a number and sixteen" translates to $\displaystyle x + 16$; twice that sum is $\displaystyle 2 (x+16)$. "Does not exceed eighty" means that it is less than or equal to eighty, so the desired inequality is

$\displaystyle 2 (x+16) \leq 80$

In terms of inequalities, we know two things. $\displaystyle x$ is greater than or equal to 14 (which is the same as $\displaystyle x$ being greater than 13). And we know $\displaystyle x$ is less than or equal to 22 (which is the same as $\displaystyle x$ being less than 23).

So, we have to find the correct statement where we can find the two inequalities. We see we need  $\displaystyle 14\leq x \text{ or } 13< x$  and $\displaystyle x\leq 22 \text{ or }x< 23$.

Therefore the only answer that fits our needs is $\displaystyle 13< x\leq 22$.

On the number line, the graph starts at –1 and ends at 3.

The line runs between –1 and 3, so we know our inequality involves only values of x that fall between these two numbers. The open circle at –1 indicates that –1 is not included, while the shaded circle on 3 indicates that 3 is included.

$\displaystyle x>-1$

$\displaystyle x\leq3$

Combining these two inequalities into one give us our answer.

$\displaystyle -1< x\leq3$

$\displaystyle 6x + 22 \leq 97$

$\displaystyle 6x + 22 -22 \leq 97-22$

$\displaystyle 6x \leq 75$

$\displaystyle 6x \div 6 \leq 75\div 6$

$\displaystyle x \leq 12.5$

or, in interval notation, $\displaystyle (-\infty , 12.5]$

$\displaystyle 6x + 22 > 67$

$\displaystyle 6x + 22 -22 > 67 -22$

$\displaystyle 6x > 45$

$\displaystyle 6x \div 6 > 45\div 6$

$\displaystyle x > 7.5$

or, in interval notation, $\displaystyle \left ( 7.5, \infty \right )$

The first step is to distribute (multiply) through the parentheses:

$\displaystyle 3(x + 4) \leq x - 6$

$\displaystyle 3x + 12 \leq x - 6$

Then subtract $\displaystyle x$ from both sides of the inequality:

$\displaystyle 2x + 12 \leq -6$

Next, subtract the 12:

$\displaystyle 2x \leq -18$

Finally, divide by two:

$\displaystyle x \leq -9$

To solve $\displaystyle 3x-4\leq 12x+16$, it is necessary to isolate the variable and the integers.

Subtract $\displaystyle 3x$ and $\displaystyle 16$ from both sides of the equation.

$\displaystyle -20\leq 9x$

Divide by nine on both sides.

$\displaystyle -\frac{20}{9}\leq x$

This answer is also the same as:  $\displaystyle x\geq -\frac{20}{9}$