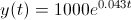All AP Calculus AB Resources
Example Questions
Example Question #1 : Solving Separable Differential Equations And Using Them In Modeling
Find (dy/dx).
sin(xy) = x + cos(y)
None of the above
dy/dx = (cos(xy) + sin(y))/(1 – cos(xy))
dy/dx = (1 – ycos(xy))/(xcos(xy) + sin(y))
dy/dx = (xcos(xy) + sin(y))/(1 – ycos(xy))
dy/dx = (1 – cos(xy))/(cos(xy) + sin(y))
dy/dx = (1 – ycos(xy))/(xcos(xy) + sin(y))
The first step of the problem is to differentiate with respect to (dy/dx):
cos(xy)[(x)(dy/dx) + y(1)] = 1 – sin(y)(dy/dx)
*Note: When differentiating cos(xy) remember to use the product rule. (xy' + x'y)
Step 2: Clean the differentiated problem up
cos(xy)(x)(dy/dx) + cos(xy)y = 1 – sin(y)(dy/dx)
cos(xy)(x)(dy/dx) + sin(y)(dy/dx) = 1 – cos(xy)y
Step 3: Solve for (dy/dx)
dy/dx = (1 – ycos(xy))/(xcos(xy) + sin(y))
Example Question #2 : Solving Separable Differential Equations And Using Them In Modeling
Find the equation of the normal line at 

The answer is 

Now plug in 


Example Question #3 : Solving Separable Differential Equations And Using Them In Modeling
What is the derivative of 
Use the quotient rule.
Example Question #4 : Solving Separable Differential Equations And Using Them In Modeling
Find 
The answer is
Example Question #1 : Solving Separable Differential Equations And Using Them In Modeling
Find the derivative:
To find the derivative, multiply the exponent by the coefficent in front of the x term and then decrease the exponent by 1:
Example Question #1 : Applications Of Antidifferentiation
Find the solution to the equation 


First, we need to solve the differential equation of 




To find 

Therefore, 
Finally, at 

Example Question #5 : Solving Separable Differential Equations And Using Them In Modeling
Solve the differential equation:
Note that 
In order to solve differential equations, you must separate the variables first.
Since point 

To get rid of the log, raise every term to the power of e:
Example Question #3 : Applications Of Antidifferentiation
Suppose $1000 is invested in an account that pays 4.3% interest compounded continuously. Find an expression for the amount in the account after time 
The differential equation is 

This is a separable first order differential equation.
Integrate both sides.
Plug in the initial condition above to see that 
Example Question #2 : Applications Of Antidifferentiation
Find the solution to the differential equation


First, separate the variables of the original differential equation:

Then, take the antiderivative of both sides, which gives

Use the given condition 





Example Question #1 : Applications Of Antidifferentiation
Differentiate 
While differentiating, multiply the exponent with the coefficient then subtract the exponent by one.
Certified Tutor
Certified Tutor
All AP Calculus AB Resources