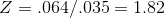AP Statistics : Significance
Study concepts, example questions & explanations for AP Statistics
All AP Statistics Resources
Example Questions
Example Question #1 : Inference
We are comparing the Democratic percentage of Detroit to the Democratic percentage of Dallas.
In Detroit, we sampled 300 people and 208 were Democrats (.693)
In Dallas, we sampled 350 people and 265 were Democrats (.757)
What is the p-value for the .064 difference?
(Assume a 2-tailed test.)
.0577
.0688
.0244
.0344
.0765
.0688
Overall percentage =
.0344 is for one tail, so .0688 is for both tails.
(Percentages are different at the .9312 level.)
Example Question #1 : Ap Statistics
Example Question #1 : How To Test Of Significance For The Slope Of A Least Squares Regression Line
A statistician conducts a regression analysis and obtains a p-value of 0.1. It is more likely than not that there is a relationship between the variables in the study.
True
False
True
A p-value of 0.1 is generally not sufficient to reject the null hypothesis, but this is only because we want a high degree of confidence before finding a relationship between variables. Here, there is most likely a relationship between the variables even though the statistician could not reject the null hypothesis.
Example Question #1 : How To Test Of Significance For The Slope Of A Least Squares Regression Line
For a data set, the least-squares regression line has a 
Based on this confidence interval, what can you do with a hypothesis test at 
Reject the null hypothesis because this confidence interval does not include 
Fail to reject the null hypothesis because this confidence interval does not include 
Reject the null hypothesis because this confidence interval does not include 
Not enough information to be able to decide.
Fail to reject the null hypothesis because this confidence interval does not include 
Reject the null hypothesis because this confidence interval does not include
Notice that the interval does not include 
Any confidence interval can be used to create a hypothesis test by inverting it, and it is fairly simple, but the concept is tested into graduate-level statistics theory.
Example Question #1 : Inference
Which of the following is an incorrect condition requirement for regression inference?
Response must vary normally about the regression line for any given value of
The standard deviation of the response must be constan
A trend/pattern of some sort in the residual plot
Ordered pairs must be independent of each other
A linear relationship between 
A trend/pattern of some sort in the residual plot
All of the following choices are correct conditions except for the choice concerning a trend/pattern of some sort in the residual plot. For regression inference to be accurate, we need to look at the residual plot of the data of interest and make sure there is random scatter. Random scatter indicates that the ordered pairs are indeed independent of each other. Any sort of pattern present in the residual plot would not satisfy that requirement, and therefore would not enable us to successfully use regression inference.
Example Question #1 : How To Do Tests Of Significance For A Mean
Example Question #2 : Inference
Suppose you conduct a paired 
The critical value for a 
Example Question #1 : How To Do Tests Of Significance For A Difference Between Two Means
Bob wants to statistically determine if the mean height of middle school boys is greater than the mean height of middle school girls. He wants to use a significance level of 
Step 1: We need to use a 2-sample z test because there are 2 samples, boys and girls. The population standard deviation, 
Step 2: This is a one-sided z test because the questions asks if the mean height of boys is greater than the mean height of girls.
Step 3: significance level, or alpha, is 
Example Question #1 : How To Define A Type Ii Error
If a test has a power of 
From the statistical definition of power (of a test), the power is equal to 
Therefore our equation to solve becomes:
Example Question #1 : Significance
You and a classmate wanted to test the effect of sugars and fats on levels of blood sugar.
Your classmate told you that they found the null hypothesis valid, which was what there is no difference between the effects of sugars and fats on blood sugar levels.
If the null hypothesis was actually false, what type of error was made?
Type II
Type I and II
Type I
Neither
Type II
A type I error occurs when the null hypothesis is valid but rejected.
A type II error occurs when the null hypothesis is false, but fails to be rejected.
Because the null hypothesis was false, but had failed to be rejected, they made a Type II error.
Certified Tutor
All AP Statistics Resources
