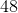The correct answer is 36. The best way to approach this problem is to take each denominator and list out its multiples. You can then find the common multiple between each denominator. 

Multiples of 6: 6, 12, 18, 24, 30, 36, 42, 48

Multiples of 3: 3, 6, 9, 12, 15, 18, 21, 24, 27, 30, 33, 36, 39, 42

Multiples of 12: 12, 24, 36, 48, 60

Multiples of 36: 36, 72, 108

Multiples of 18: 18, 36, 54, 72

From the above multiples, we can see that the least common denominator is 36.

The least common denominator is the lowest number that has each denominator is a potential factor.

1. Multiply the two lowest denominators:

2. Check to see if the other denominators can factor into that denominator:

So 24 is your least common denominator. (48 is also a common denominator of all of these fractions, but it is not the lowest one)

To find the least common denominator, list out the multiples of both denominators until you find the smallest multiple that is shared by both.

4: 4, 8, 12, 16, 20, 24, 28, 32

7: 7, 14, 21, 28, 35

Because 28 is the first shared multiple of 4 and 7, it must be the least common denominator for these two fractions.

To find the least common denominator, list out the multiples of both denominators until you find the smallest multiple that is shared by both.

4: 4, 8, 12, 16, 20, 24

5: 5, 10, 15, 20, 25

Because 20 is the first shared multiple of 4 and 5, it must be the least common denominator for these two fractions.

The least common denominator is the lowest common multiple of the denominators.

Multiple of 27: 27, 54, 81, 108, 135, 162, 189, 216, 243, 270

Multiple of 9: 9, 18, 27, 36, 45, 54, 63, 72, 81, 90

The first step of finding the LCD of a set of fractions is to make sure each of the fractions are simplified.  and  are already simplified. However,  can be reduced to . This makes the problem much easier because we now only have two different denominators to work with. From here, we simply multiply each denominator by increasing integers until we get a common denominator. It is important to always increase the lower of the two denominators. For instance, we have 4 and 3 as denominators in this problem. Since 3 is lower, we will multiply it by 2, getting 6. Now we have 4 and 6. 4 is lower, so we multiply it by 2 to get 8. Now we have 8 and 6. 6 is lower, so we multiply the original denominator of 3 by 3, resulting in denominators of 8 and 9. Following this trend, we get: 12 and 9, then 12 and 12. Therefore, 12 will be the least common denominator.

While simply multiplying all of the denominators will get you a common denominator between the fractions, it does not always give you the LCD.

When finding the least common denominator, the quickest way is to multiply the numbers out.

In this case  and  are both primes and don't share any factors other than .

We can multiply them to get  as the final answer.

Another approach is to list out all the factors of each number and see which factor is in both sets first.

Notice  appears in both sets before any other number therefore, this is the least common denominator.

When finding the least common denominator, the quickest way is to multiply the numbers out.

In this case  and  share a factor other than  which is . We can divide those numbers by  to get  and  leftover.

Now, they don't share a common factor so basically multiply them out with the shared factor. Answer is .

Another approach is to list out the factors of both number and find the factor that appears in both sets first.

We can see that  appears in both sets before any other number thus, this is our answer.

When finding the least common denominator, the quickest way is to multiply the numbers out. In this case  and  share a factor other than  which is . We can divide those numbers by  to get  and  leftover. Now, they don't share a common factor so basically multiply them out with the shared factor. Answer is .

Another approach is to list out the factors of each number. The factor that appears first in both set is the least common denominator.

We see that  appears first in both sets and thus, is the least common denominator.

When finding the least common denominator, the quickest way is to multiply the numbers out. In the case of finding least common denominators among three or more numbers, it's critical there are no common factors between two of the denominators and of course all 3. This will ensure the answer will always be the least common denominator. 

Say we just multiplied the numbers out. It's basically  or . That number seems big but lets cut this in half and check   divides evenly into , , and . Lets check .  doesn't divide evenly into  so  is the answer. 

So this goes back to the statement: "In the case of finding least common denominators among three or more numbers, it's critical there are no common factors between two of the denominators and of course all 3." If I factored a , I can reduce the  and  but not the . That is ok. Now the leftover values are , , and . They only share a factor of . So let's multiply the leftover values and the factored value to get