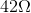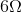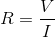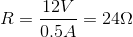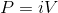All College Physics Resources
Example Questions
Example Question #21 : Electromagnetics, Waves, And Optics
You are given three resistors with known values:
You are asked to create a circuit with a total resistance of between 



















This question requires no math to correctly answer! You should not need to 'brute force' it. Although it is designed to appear time consuming, it should be relatively easily once the principle of resistors in parallel is understood. Whenever two resistors are connected in parallel, the net resistance must be less than the resistance of either of the two alone. When resistors are connected in series, the net resistance must be more than the resistance of either alone.
Explanation of correct answer:










Explanations of incorrect answers:






















Example Question #92 : College Physics
Which of the following expressions gives the capacitance for a capacitor in a circuit in which the only factors known are a) the current through the circuit, b) the resistance of the circuit, and c) the charge that has accumulated on the capacitor?
In this question, we're given a number of parameters of a circuit and are asked to find how we can use these various parameters to show the capacitance of the circuit's capacitor.
First, recall what a capacitor is; something that stores charge for a given voltage difference. In other words, when there is a voltage difference between the two plates of a capacitor, a certain amount of charge can be stored on these plates. The more charge that can be stored for a given voltage difference, the greater that capacitor's capacitance. This can be shown by the following equation.
From the above expression, 


To put the voltage into different terms, we'll need to use Ohm's law, which states the following.
In other words, the voltage is proportional to both the current and the resistance of the circuit.
Since we are given both current and resistance as known parameters in the question stem, we can use these for our final answer. By substituting the 

Example Question #1 : Circuits
A parallel plate capacitor with no dielectric has capacitance 
The capacitance of a parallel plate capacitor is proportional to the inverse of the distance between the plates. If the distance is halved, the capacitance is doubled.
Example Question #1 : Capacitors
An RC circuit is connected to a 


The formula for finding the voltage of a simple RC circuit is





We want to know when the capacitor will reach the voltage of the power source 

Using the properties of natural logs yields
Solving for 
Example Question #22 : Electromagnetics, Waves, And Optics
A resistive heating element can be modeled as a resistor connected across the terminals of a battery.
If the battery is selected to be 

We are asked to calculate the power dissipated through a resistor connected to a battery. We know that 


Example Question #1 : Circuits
What is the RMS voltage of a 
This question requires two steps: the first is to calculate the peak voltage of the AC current, and the second requires conversion of the peak voltage to an RMS voltage. We are told that the current is 


Example Question #3 : Power
A 

The power dissipated in each light bulb is 
Example Question #2 : Circuits
What size resistor should be connected across the terminals of a 

Ohm's law is 
In this case, 

Solving for the resistance, 
Substitute known values and solve for the unknown resistance:
Example Question #1 : Ohm's Law And Current
A 

Ohm's law relates voltage to current and resistance, 
Example Question #2 : Ohm's Law And Current
Suppose that a circuit with a single resistor is connected to a 

For this problem, let's first take note of what we know and what we don't. We're given voltage and power, but we're not given resistance or current. Since we have two unknowns, we're going to need two equations.
One of the equations that we can use is the power generated by current flowing through a resistor.
In addition, we also need to use Ohm's law.
With these two equations in mind, we can relate them to one another by isolating the variable for current in one equation and then substituting it into the other one.
Manipulating Ohm's law:
Then substituting this into the power equation:
And rearranging to isolate the variable for resistance:
Now we just need to plug in the values from the question stem to obtain the answer:
All College Physics Resources