All GED Math Resources
Example Questions
Example Question #1 : Perimeter And Sides

Identify the above polygon.
Octagon
Pentagon
Hexagon
Quadrilateral
Octagon
A polygon with eight sides is called an octagon.
Example Question #1 : Perimeter And Sides
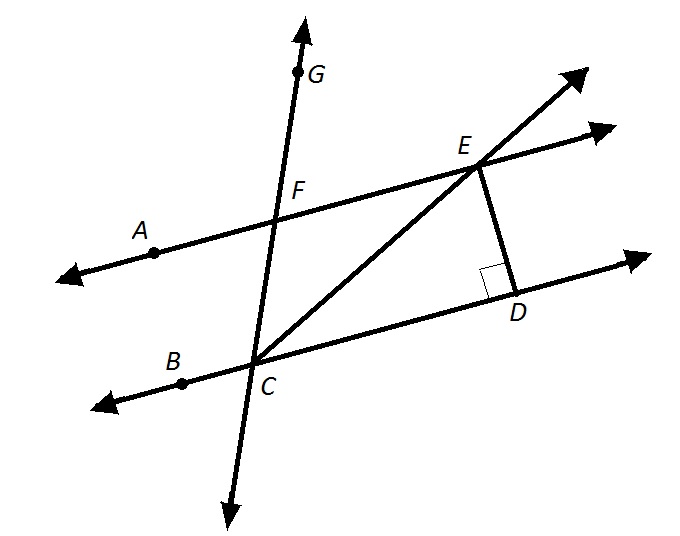
Refer to the above figure.
Which of the following is not a valid alternative name for Polygon 
Polygon
Polygon
Polygon
Polygon
Polygon
In naming a polygon, the vertices must be written in the order in which they are positioned, going either clockwise or counterclockwise. Of the four choices, only Polygon 




Example Question #2 : Perimeter And Sides

Refer to the above figure. All angles shown are right angles.
What is the perimeter of the figure?
The figure can be viewed as the composite of rectangles. As such, we can take advantage of the fact that opposite sides of a rectangle have the same length, as follows:

Now that the missing sidelengths are known, we can add the sidelengths to find the perimeter:
Example Question #3 : Perimeter And Sides

Refer to the above figure.
Which of the following segments is a diagonal of Pentagon 
A diagonal of a polygon is a segment whose endpoints are nonconsecutive vertices of the polygon. Of the four choices, only 
Example Question #5 : Perimeter And Sides

Classify the above polygon.
Hexagon
Pentagon
Rhombus
Octagon
Octagon
A polygon with eight sides is called an octagon.
Example Question #3 : Other Shapes

Classify the above polygon.
Pentagon
Hexagon
Trapezoid
Octagon
Hexagon
A polygon with six sides is called a hexagon.
Example Question #4 : Perimeter And Sides
Hexagon 



I) Rectangle
II) Rhombus
III) Square
IV) Trapezoid
I only
I, II, and III only
II only
IV only
I only
The figure described is below.

Since the hexagon is regular, its sides are congruent, and its angles each have measure 
Also, each of the triangles are isosceles, and their acute angles measure 



The correct response is I only.
Example Question #5 : Perimeter And Sides

The above figure is a regular octagon. Give its perimeter in yards.
A regular octagon has eight sides of equal length, so multiply the length of one side by eight:

Divide by three to get the equivalent in yards:

Example Question #401 : 2 Dimensional Geometry
What is the perimeter of a semicircle with an area of 
Write the formula for the area of a semicircle.
Substitute the area.
Multiply by 2, and divide by pi on both sides.
The equation becomes:
Square root both sides and factor the right side.
The diameter is double the radius.
The circumference is half the circumference of a full circle.
The perimeter is the sum of the diameter and the half circumference.
The answer is:
Example Question #401 : Geometry And Graphs
A hexagon has a perimeter of 90in. Find the length of one side.
A hexagon has 6 equal sides. The formula to find perimeter of a hexagon is:
where a is the length of any side. Now, to find the length of one side, we will solve for a.
We know the perimeter of the hexagon is 90in. So, we will substitute and solve for a. We get
Therefore, the length of one side of the hexagon is 15in.
All GED Math Resources












































