All High School Math Resources
Example Questions
Example Question #1 : Equilateral Triangles
You are given that the perimeter of an equilateral triangle is 






By definition, an equilateral triangle has three congruent sides. The perimeter is the sum of those sides. Thus, to find the length of just one of those sides, we can divide the perimeter of the triangle by three.



Example Question #1 : How To Find The Area Of An Equilateral Triangle
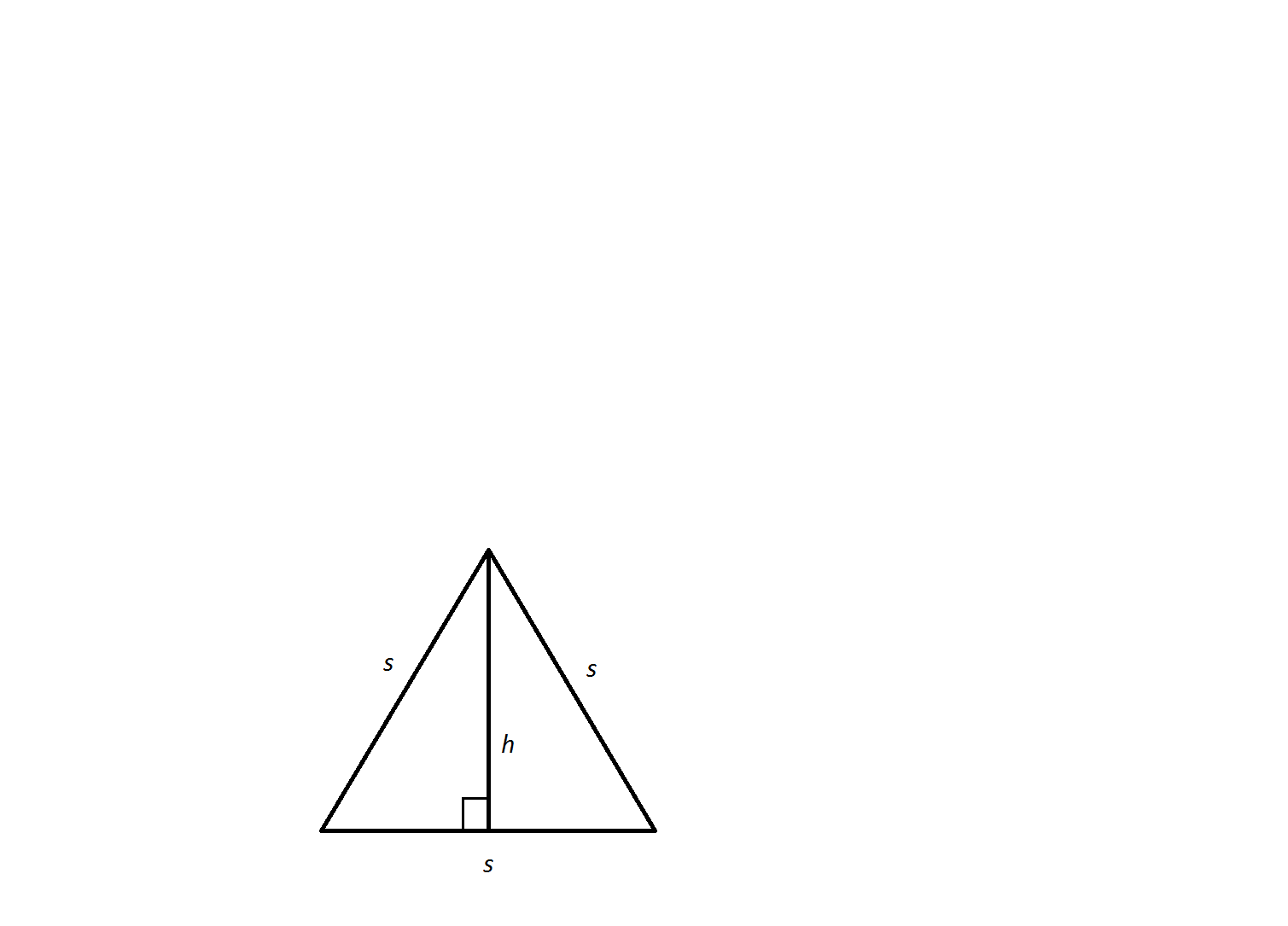
An equilateral triangle has a side length of 
Not enough information to solve
The area of an equilateral triangle is found using the following formula.

Example Question #1 : Equilateral Triangles
The area of square ABCD is 50% greater than the perimeter of the equilateral triangle EFG. If the area of square ABCD is equal to 45, then what is the area of EFG?
25√3
50
50√3
25
30
25√3
If the area of ABCD is equal to 45, then the perimeter of EFG is equal to x * 1.5 = 45. 45 / 1.5 = 30, so the perimeter of EFG is equal to 30. This means that each side is equal to 10.
The height of the equilateral triangle EFG creates two 30-60-90 triangles, each with a hypotenuse of 10 and a short side equal to 5. We know that the long side of 30-60-90 triangle (here the height of EFG) is equal to √3 times the short side, or 5√3.
We then apply the formula for the area of a triangle, which is 1/2 * b * h. We get 1/2 * 10 * 5√3 = 5 * 5√3 = 25√3.
In general, the height of an equilateral triangle is equal to √3 / 2 times a side of the equilateral triangle. The area of an equilateral triangle is equal to 1/2 * √3s/ 2 * s = √3s2/4.
Example Question #2 : Equilateral Triangles
What is the area of an equilateral triangle with sides 12 cm?
36√3
72√3
12√2
54√2
18√3
36√3
An equilateral triangle has three congruent sides and results in three congruent angles. This figure results in two special right triangles back to back: 30° – 60° – 90° giving sides of x - x √3 – 2x in general. The height of the triangle is the x √3 side. So Atriangle = 1/2 bh = 1/2 * 12 * 6√3 = 36√3 cm2.
Example Question #4 : Equilateral Triangles
The length of one side of an equilateral triangle is ten. What is the area of the triangle?
To calculate the height, the length of a perpendicular bisector must be determined. If a perpendicular bisector is drawn in an equilateral triangle, the triangle is divided in half, and each half is a congruent 30-60-90 right triangle. This type of triangle follows the equation below.
The length of the hypotenuse will be one side of the equilateral triangle.

The side of the equilateral triangle that represents the height of the triangle will have a length of 
To calculate the area of the triangle, multiply the base (one side of the equilateral triangle) and the height (the perpendicular bisector) and divide by two.
Example Question #5 : Equilateral Triangles

What is the area of an equilateral triangle with a side length of 
Not enough information to solve
In order to find the area of the triangle, we must first calculate the height of its altitude. An altitude slices an equilateral triangle into two 



Therefore, we can find the height of the altitude of this triangle by designating a value for 



Now, we can calculate the area of the triangle via the formula 
Example Question #2 : Equilateral Triangles

An equilateral triangle has a side length of 
Not enough information to solve
In order to find the area of the triangle, we must first calculate the height of its altitude. An altitude slices an equilateral triangle into two 



Therefore, we can find the height of the altitude of this triangle by designating a value for 

that 

Now, we can calculate the area of the triangle via the formula
Now convert to meters.
Example Question #1 : How To Find The Area Of An Equilateral Triangle
Triangle A: A right triangle with sides length 

Triangle B: An equilateral triangle with side lengths 
Which triangle has a greater area?
There is not enough information given to determine which triangle has a greater area.
Triangle B
The areas of the two triangles are the same.
Triangle A
Triangle B
The formula for the area of a right triangle is 


The formula for the area of an equilateral triangle is 


To determine which of the two areas is greater without using a calculator, rewrite the areas of the two triangles with comparable factors. Triangle A's area can be expressed as 



Example Question #2 : How To Find The Area Of An Equilateral Triangle
What is the area of an equilateral triangle with side 11?
Since the area of a triangle is
you need to find the height of the triangle first. Because of the 30-60-90 relationship, you can determine that the height is 
Then, multiply that by the base (11).
Finally, divide it by two to get 52.4.
Example Question #7 : How To Find The Area Of An Equilateral Triangle
Find the area of the following equilateral triangle:

The formula for the area of an equilateral triangle is:
Where 
Plugging in our values, we get:
Certified Tutor
Certified Tutor
All High School Math Resources
























































