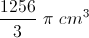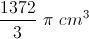All Intermediate Geometry Resources
Example Questions
Example Question #1 : Spheres
A sphere has a circumference of 
The circumference is given by 
Example Question #2 : Spheres
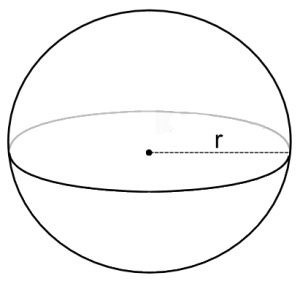
Example Question #3 : Spheres
What is the volume of a sphere with a diameter of 6 in?
The formula for the volume of a sphere is:
where 
Example Question #1 : Spheres
The circumference of a sphere is 
The formula to find the volume of a sphere is:
Finding the volume is simple. All that we need is the radius!
The only information the problem provides is the circumference. In order to find the radius, we have to think how circumference relates to radius.
Since the equation for circumference is 
The first step in solving this problem is to determine the diameter from the circumference:
Because the diameter is 

Now we are ready to solve for the volume after substituting in our 
Example Question #4 : How To Find The Volume Of A Sphere
A cube with a side length of 20 inches has a sphere inscribed within. What is the volume of the sphere?
The cube has a side length of 20 inches. Since the sphere is inscribed within the cube its diameter measures 20 inches; the radius will be 10 inches.
The volume of a sphere is given by

Example Question #5 : How To Find The Volume Of A Sphere
A sphere is cut in half as shown by the figure below.

If the radius of the sphere is 
Recall how to find the volume of a sphere:
Now since we only have half a sphere, divide the volume by 
Plug in the given radius to find the volume of the figure.
Make sure to round to 
Example Question #6 : How To Find The Volume Of A Sphere
A sphere is cut in half as shown by the figure below.

If the radius of the sphere is 
Recall how to find the volume of a sphere:
Now since we only have half a sphere, divide the volume by 
Plug in the given radius to find the volume of the figure.
Make sure to round to 
Example Question #7 : How To Find The Volume Of A Sphere
A sphere is cut in half as shown by the figure below.

If the radius of the sphere is 
Recall how to find the volume of a sphere:
Now since we only have half a sphere, divide the volume by 
Plug in the given radius to find the volume of the figure.
Make sure to round to 
Example Question #1 : Spheres
A sphere is cut in half as shown by the figure below.

If the radius of the sphere is 
Recall how to find the volume of a sphere:
Now since we only have half a sphere, divide the volume by 
Plug in the given radius to find the volume of the figure.
Make sure to round to 
Example Question #9 : How To Find The Volume Of A Sphere
A sphere is cut in half as shown by the figure below.

If the radius of the sphere is 
Recall how to find the volume of a sphere:
Now since we only have half a sphere, divide the volume by 
Plug in the given radius to find the volume of the figure.
Make sure to round to 
All Intermediate Geometry Resources