We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

Introduction to Analysis : Intro Analysis

Study concepts, example questions & explanations for Introduction to Analysis

Create An Account

All Introduction to Analysis Resources

12 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : The Real Number System

Identify the following property.

On the space $\mathbb{R}\times \mathbb{R}$ where , $b\ \epsilon\ \mathbb{R}$ only one of the following statements holds true a<b , b<a , or a=b .

Possible Answers:

Transitive Property

Existence of Multiplicative Identity

Multiplicative Property

Distributive Law

Trichotomy Property

Correct answer:

Trichotomy Property

Explanation:

The real number system, $\mathbb{R}\times \mathbb{R}$ contains order axioms that show relations and properties of the system that add completeness to the ordered field algebraic laws.

The properties are as follows.

Trichotomy Property:

Given , $b\ \epsilon\ \mathbb{R}$ only one of the following statements holds true a<b , b<a , or a=b .

Transitive Property:

For , , and $c\ \epsilon\ \mathbb{R}$ where a<b and b<c then this implies a<c .

Additive Property:

For , , and $c\ \epsilon\ \mathbb{R}$ where a<b and $c\ \epsilon\ \mathbb{R}$ then this implies a+b<b+c .

Multiplicative Properties:

For , , and $c\ \epsilon\ \mathbb{R}$ where a0 then this implies ac<bc and a<b and c<0 then this implies bc<ac .

Therefore looking at the options the Trichotomy Property identifies the property in this particular question.

Report an Error

Example Question #2 : The Real Number System

Identify the following property.

For , , and $c\ \epsilon\ \mathbb{R}$ where a<b and b<c then this implies a<c .

Possible Answers:

Distribution Laws

Transitive Property

Trichotomy Property

Additive Property

Multiplicative Properties

Correct answer:

Transitive Property

Explanation:

The real number system, $\mathbb{R}\times \mathbb{R}$ contains order axioms that show relations and properties of the system that add completeness to the ordered field algebraic laws.

The properties are as follows.

Trichotomy Property:

Given , $b\ \epsilon\ \mathbb{R}$ only one of the following statements holds true a<b , b<a , or a=b .

Transitive Property:

For , , and $c\ \epsilon\ \mathbb{R}$ where a<b and b<c then this implies a<c .

Additive Property:

For , , and $c\ \epsilon\ \mathbb{R}$ where a<b and $c\ \epsilon\ \mathbb{R}$ then this implies a+b<b+c .

Multiplicative Properties:

For , , and $c\ \epsilon\ \mathbb{R}$ where a0 then this implies ac<bc and a<b and c<0 then this implies bc<ac .

Therefore looking at the options the Transitive Property identifies the property in this particular question.

Report an Error

Example Question #3 : The Real Number System

Identify the following property.

For , , and $c\ \epsilon\ \mathbb{R}$ where a<b and $c\ \epsilon\ \mathbb{R}$ then this implies a+b<b+c .

Possible Answers:

Multiplicative Properties

Additive Property

Distribution Laws

Transitive Property

Trichotomy Property

Correct answer:

Additive Property

Explanation:

The real number system, $\mathbb{R}\times \mathbb{R}$ contains order axioms that show relations and properties of the system that add completeness to the ordered field algebraic laws.

The properties are as follows.

Trichotomy Property:

Given , $b\ \epsilon\ \mathbb{R}$ only one of the following statements holds true a<b , b<a , or a=b .

Transitive Property:

For , , and $c\ \epsilon\ \mathbb{R}$ where a<b and b<c then this implies a<c .

Additive Property:

For , , and $c\ \epsilon\ \mathbb{R}$ where a<b and $c\ \epsilon\ \mathbb{R}$ then this implies a+b<b+c .

Multiplicative Properties:

For , , and $c\ \epsilon\ \mathbb{R}$ where a0 then this implies ac<bc and a<b and c<0 then this implies bc<ac .

Therefore looking at the options the Additive Property identifies the property in this particular question.

Report an Error

Example Question #4 : The Real Number System

Identify the following property.

For , , and $c\ \epsilon\ \mathbb{R}$ where a0 then this implies ac<bc and a<b and c<0 then this implies bc<ac .

Possible Answers:

Distribution Laws

Multiplicative Properties

Additive Property

Transitive Property

Trichotomy Property

Correct answer:

Multiplicative Properties

Explanation:

The real number system, $\mathbb{R}\times \mathbb{R}$ contains order axioms that show relations and properties of the system that add completeness to the ordered field algebraic laws.

The properties are as follows.

Trichotomy Property:

Given , $b\ \epsilon\ \mathbb{R}$ only one of the following statements holds true a<b , b<a , or a=b .

Transitive Property:

For , , and $c\ \epsilon\ \mathbb{R}$ where a<b and b<c then this implies a<c .

Additive Property:

For , , and $c\ \epsilon\ \mathbb{R}$ where a<b and $c\ \epsilon\ \mathbb{R}$ then this implies a+b<b+c .

Multiplicative Properties:

For , , and $c\ \epsilon\ \mathbb{R}$ where a0 then this implies ac<bc and a<b and c<0 then this implies bc<ac .

Therefore looking at the options the Multiplicative Properties identifies the property in this particular question.

Report an Error

Example Question #5 : The Real Number System

Determine whether the following statement is true or false:

If 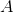is a nonempty subset of $\mathbb{N}$ , then has a finite infimum and it is an element of .

Possible Answers:

True

False

Correct answer:

True

Explanation:

According to the Well-Ordered Principal this statement is true. The following proof illuminate its truth.

Suppose $A\subseteq \mathbb{N}$ is nonempty. From there, it is known that is bounded above, by .

Therefore, by the Completeness Axiom the supremum of exists.

Furthermore, if $A\subset \mathbb{Z}$ has a supremum, then $\text{sup}A\ \epsilon\ A$ , thus in this particular case $\text{sup}(-A)\ \epsilon\ -A$ .

Thus by the Reflection Principal,

$\text{inf}A=-\text{sup}(-A)$

exists and

$\text{inf}A\ \epsilon\ -(-A)=A$ .

Therefore proving the statement in question true.

Report an Error

Example Question #1 : Sequences In Real Numbers (R)

What term does the following define.

A sequence of sets $\begin{Bmatrix} I_n \end{Bmatrix}_{n\ \epsilon\ \mathbb{N}}$ is __________ if and only if $I_1\supseteq I_2\supseteq ...$ .

Possible Answers:

Increasing

Decreasing

Unbounded

Nested

Bounded

Correct answer:

Nested

Explanation:

This statement:

A sequence of sets $\begin{Bmatrix} I_n \end{Bmatrix}_{n\ \epsilon\ \mathbb{N}}$ is __________ if and only if $I_1\supseteq I_2\supseteq ...$

is the definition of nested.

This means that the sequence 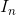I_n for all elements, for which belongs to the natural numbers, is considered a nested set if and only if the subsequent sets are subsets of it.

Other theorems in intro analysis build off this understanding.

Report an Error

Example Question #1 : Intro Analysis

Determine whether the following statement is true or false:

Let , , , and $d\ \epsilon\ \mathbb{R}$ . If a<b and c<d<0 then ac<bd .

Possible Answers:

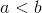
True

False

Correct answer:

False

Explanation:

Determine this statement is false by showing a contradiction when actual values are used.

Let

a=1, b=2, c=-3, d=-2

First make sure the inequalities hold true.

$\\a<b \\1<2$

and

$\\c<d<0 \\-3<-2<0$

Now find the products.

$\\ac<bd \\(1)(-3)<(2)(-2) \\-3\nless -4$

Therefore, the statement is false.

Report an Error

Example Question #1 : Riemann Integral, Riemann Sums, & Improper Riemann Integration

What conditions are necessary to prove that the upper and lower integrals of a bounded function exist?

Possible Answers:

, $b\ \epsilon\ \mathbb{Z}$ , a<b , and $f:[a,b]\rightarrow \mathbb{R}$ be bounded

, $b\ \epsilon\ \mathbb{R}$ , a<b , and $f:[a,b]\rightarrow \mathbb{Z}$ be bounded

, $b\ \epsilon\ \mathbb{R}$ , a<b , and $f:[a,b]\rightarrow \mathbb{R}$ be bounded

, $b\ \epsilon\ \mathbb{R}$ , a>b , and $f:[a,b]\rightarrow \mathbb{R}$

, $b\ \epsilon\ \mathbb{R}$ , a>b , and $f:[a,b]\rightarrow \mathbb{Z}$

Correct answer:

, $b\ \epsilon\ \mathbb{R}$ , a<b , and $f:[a,b]\rightarrow \mathbb{R}$ be bounded

Explanation:

Using the definition for Riemann sums to define the upper and lower integrals of a function answers the question.

According the the Riemann sum where U(f,P) represents the upper integral and L(f,P) the following are defined:

1. The upper integral of on [a,b] is

$(U)\int_a^bf(x)dx:=\text{inf}\begin{Bmatrix} U(f,p)) \end{Bmatrix}$ where is a partition of [a,b] .

2. The lower integral of on [a,b] is

$(L)\int_a^bf(x)dx:=\text{sup}\begin{Bmatrix} L(f,p)) \end{Bmatrix}$ where is a partition of [a,b] .

3. If 1 and 2 are the same then the integral is said to be

$\int_a^bf(x):=(U)\int_a^bf(x)dx=(L)\int_a^bf(x)dx$

if and only if , $b\ \epsilon\ \mathbb{R}$ , a<b , and $f:[a,b]\rightarrow \mathbb{R}$ be bounded.

Therefore the necessary condition for the proving the upper and lower integrals of a bounded function exists is if and only if , $b\ \epsilon\ \mathbb{R}$ , a<b , and $f:[a,b]\rightarrow \mathbb{R}$ be bounded.

Report an Error

Example Question #2 : Riemann Integral, Riemann Sums, & Improper Riemann Integration

What term has the following definition.

, $b\ \epsilon\ \mathbb{R}$ and a<b . Over the interval [a,b] is a set of points $P=\begin{Bmatrix} x_0,x_1,...,x_n \end{Bmatrix}$ such that

a=x_0<x_1<...<x_n=b

Possible Answers:

Partition

Norm

Refinement of a partition

Lower Riemann sum

Upper Riemann sum

Correct answer:

Partition

Explanation:

By definition

If , $b\ \epsilon\ \mathbb{R}$ and a<b .

A partition over the interval [a,b] is a set of points $P=\begin{Bmatrix} x_0,x_1,...,x_n \end{Bmatrix}$ such that

a=x_0<x_1<...<x_n=b .

Therefore, the term that describes this statement is partition.

Report an Error

Example Question #3 : Riemann Integral, Riemann Sums, & Improper Riemann Integration

What term has the following definition.

The __________ of a partition $P=\begin{Bmatrix} x_0,x_1,...,x_n \end{Bmatrix}$ is

$||P||=\max_{1\leq j\leq n}|x_j-x_{j-1}|$

Possible Answers:

Refinement of a partition

Norm

Lower Riemann sum

Partition

Upper Riemann Sum

Correct answer:

Norm

Explanation:

By definition

If , $b\ \epsilon\ \mathbb{R}$ and a<b .

A partition over the interval [a,b] is a set of points $P=\begin{Bmatrix} x_0,x_1,...,x_n \end{Bmatrix}$ such that

a=x_0<x_1<...<x_n=b .

Furthermore,

The norm of the partition

$P=\begin{Bmatrix} x_0,x_1,...,x_n \end{Bmatrix}$ is

$||P||=\max_{1\leq j\leq n}|x_j-x_{j-1}|$

Therefore, the term that describes this statement is norm.

Report an Error

View Tutors

Timothy
Certified Tutor

Bethune-Cookman University, Bachelor of Science, Mathematics. University of Phoenix, Master of Science, Computer and Informat...

View Tutors

Thomas
Certified Tutor

California State University-Dominguez Hills, Bachelor in Arts, Studio Arts. University of California-San Diego, Doctor of Phi...

View Tutors

Melissa
Certified Tutor

University of Houston, Bachelors, Political Science and Government.

All Introduction to Analysis Resources

12 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

LSAT Tutors in Washington DC, Reading Tutors in Los Angeles, Computer Science Tutors in Seattle, GRE Tutors in Boston, SSAT Tutors in Boston, Math Tutors in Dallas Fort Worth, English Tutors in Washington DC, Calculus Tutors in Denver, SAT Tutors in New York City, Reading Tutors in San Francisco-Bay Area

Popular Courses & Classes

MCAT Courses & Classes in Washington DC, ISEE Courses & Classes in Philadelphia, LSAT Courses & Classes in Chicago, ACT Courses & Classes in Los Angeles, ISEE Courses & Classes in Seattle, SAT Courses & Classes in Boston, SSAT Courses & Classes in Washington DC, ACT Courses & Classes in Philadelphia, ACT Courses & Classes in Phoenix, GRE Courses & Classes in San Francisco-Bay Area

Popular Test Prep

SSAT Test Prep in Los Angeles, ACT Test Prep in Los Angeles, GMAT Test Prep in Denver, GMAT Test Prep in Chicago, ACT Test Prep in Phoenix, LSAT Test Prep in Philadelphia, LSAT Test Prep in New York City, GMAT Test Prep in Miami, ISEE Test Prep in San Francisco-Bay Area, ACT Test Prep in Houston

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Introduction to Analysis : Intro Analysis

Study concepts, example questions & explanations for Introduction to Analysis

All Introduction to Analysis Resources

Example Questions

Example Question #1 : The Real Number System

Example Question #2 : The Real Number System

Example Question #3 : The Real Number System

Example Question #4 : The Real Number System

Example Question #5 : The Real Number System

Example Question #1 : Sequences In Real Numbers (R)

Example Question #1 : Intro Analysis

Example Question #1 : Riemann Integral, Riemann Sums, & Improper Riemann Integration

Example Question #2 : Riemann Integral, Riemann Sums, & Improper Riemann Integration

Example Question #3 : Riemann Integral, Riemann Sums, & Improper Riemann Integration

All Introduction to Analysis Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: