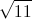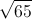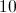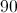Call Now to Set Up Tutoring:

(888) 888-0446

ISEE Upper Level Math : Right Triangles

Study concepts, example questions & explanations for ISEE Upper Level Math

Create An Account

All ISEE Upper Level Math Resources

6 Diagnostic Tests 244 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #4 : Triangles

A right triangle has a hypotenuse of 10 and a side of 6. What is the missing side?

Possible Answers:

Correct answer:

Explanation:

To find the missing side, use the Pythagorean Theorem (a^2+b^2=c^2) . Plug in (remember c is always the hypotenuse!) so that 6^2+b^2=10^2 . Simplify and you get 36+b^2=100. Subtract 36 from both sides so that you get b^2=64. Take the square root of both sides. B is 8.

Report an Error

Example Question #44 : Geometry

Right_triangle

Refer to the above diagram. Which of the following quadratic equations would yield the value of as a solution?

Possible Answers:

$2x^{2}+6x-391 = 0$

$x^{2}+6x+409 = 0$

$2x^{2}+6x+409 = 0$

$2x^{2}+6x-11 = 0$

$x^{2}+6x-391 = 0$

Correct answer:

$2x^{2}+6x-391 = 0$

Explanation:

By the Pythagorean Theorem,

$x^{2}+ (x+3)^{2} = 20^{2}$

$x^{2}+ x^{2}+6x+9= 400$

$2x^{2}+6x-391 = 0$

Report an Error

Example Question #1 : How To Find The Length Of The Side Of A Right Triangle

Right_triangle

Note: Figure NOT drawn to scale.

Refer to the above diagram. Which of the following quadratic equations would yield the value of as a solution?

Possible Answers:

$2x^{2} +4x-24= 0$

$x^{2} -4x+74= 0$

$x^{2} +4x+74= 0$

$2x^{2} -4x-24= 0$

$x^{2} -4x-24= 0$

Correct answer:

$x^{2} -4x-24= 0$

Explanation:

By the Pythagorean Theorem,

$x^{2}+ (x+5)^{2} = (x+7)^{2}$

$x^{2}+ x^{2} +10x+25 = x^{2} +14x +49$

$2x^{2} +10x+25 = x^{2} +14x +49$

$x^{2} -4x-24= 0$

Report an Error

Example Question #1 : Right Triangles

Right_triangle

Note: Figure NOT drawn to scale.

Refer to the above diagram.

AF = 6 , FD = 8

Find the length of $\overline{BC}$ .

Possible Answers:

$22\frac{2}{9}$

$16\frac{2}{3}$

$33\frac{1}{3}$

Correct answer:

$22\frac{2}{9}$

Explanation:

First, find .

Since $\overline{DF }$ is an altitude of right $\Delta ADB$ to its hypotenuse,

$\frac{FB}{FD}= \frac{FD}{AF}$

$\frac{FB}{8}= \frac{8}{6}$

$FB= \frac{8}{6} \cdot 8 = 10 \frac{2}{3}$

$AB = AF + FB = 6 + 10 \frac{2}{3} = 16 \frac{2}{3}$

$\Delta AFD \sim \Delta ABC$ by the Angle-Angle Postulate, so

$\frac{BC}{FD} = \frac{AB}{AF}$

$\frac{BC}{8} = \frac{16 \frac{2}{3}}{6}$

$BC = 16 \frac{2}{3}\cdot 8 \div 6 = 22\frac{2}{9}$

Report an Error

Example Question #51 : Geometry

Right_triangle

Note: Figure NOT drawn to scale.

Refer to the above diagram.

DE = 6, EC = 12

Find the length of $\overline{AB}$ .

Possible Answers:

$7\frac{1}{2}$

$7\frac{1}{4}$

$7\frac{3}{4}$

Correct answer:

$7\frac{1}{2}$

Explanation:

First, find .

Since $\overline{DE}$ is an altitude of $\Delta CDB$ from its right angle to its hypotenuse,

$\Delta DEC \sim \Delta BED$

$\frac{BE}{DE}= \frac{ED}{EC}$

$\frac{BE}{6}= \frac{6}{12}$

$BE= \frac{6}{12} \cdot 6 = 3$

CB =BE + EC = 3+ 12 = 15

$\Delta DEC \sim \Delta ABC$ by the Angle-Angle Postulate, so

$\frac{AB}{DE} = \frac{CB}{CE}$

$\frac{AB}{6} = \frac{15}{12}$

$AB= \frac{15}{12} \cdot 6$

$AB = 7\frac{1}{2}$

Report an Error

Example Question #4 : Triangles

Right_triangle

Note: Figure NOT drawn to scale.

Refer to the above diagram. Evaluate .

Possible Answers:

$30\frac{3}{4}$

$3\frac{3}{8}$

$15\frac{3}{8}$

$6\frac{3}{4}$

Correct answer:

$3\frac{3}{8}$

Explanation:

By the Pythagorean Theorem,

$x^{2}+ 15^{2} = (x+12)^{2}$

$x^{2}+ 225 = x^{2}+24x+144$

225 = 24x+144

24x= 81

$x= 3\frac{3}{8}$

Report an Error

Example Question #52 : Geometry

A right triangle $\Delta ABC$ with hypotenuse $\overline{AC}$ is inscribed in $\odot O$ , a circle with radius 26. If AB = 20 , evaluate the length of $\overline{BC}$ .

Possible Answers:

Insufficient information is given to answer the question.

Correct answer:

Explanation:

The arcs intercepted by a right angle are both semicircles, so hypotenuse $\overline{AC}$ shares its endpoints with two semicircles. This makes $\overline{AC}$ a diameter of the circle, and $AC = 2r = 2 \cdot 26 = 52$ .

By the Pythagorean Theorem,

$BC = \sqrt{(AC)^{2} - (AB)^{2}} = \sqrt{52^{2} -20^{2}} = \sqrt{2,704-400} = \sqrt{2,304} = 48$

Report an Error

Example Question #1 : How To Find If Right Triangles Are Similar

$\Delta ABC \sim \Delta DEF$

$\angle B$ is a right angle; DE = 21 , DF = 42 .

Which is the greater quantity?

(a) $m \angle A$

(b) $60 ^{\circ }$

Possible Answers:

(b) is greater.

(a) and (b) are equal.

(a) is greater.

It is impossible to tell from the information given.

Correct answer:

(a) and (b) are equal.

Explanation:

$\Delta ABC \sim \Delta DEF$ . Corresponding angles of similar triangles are congruent, so since $\angle B$ is a right angle, so is $\angle E$ .

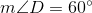
The hypotenuse $\overline{DF}$ of $\Delta DEF$ is twice as long as leg $\overline{DE}$ ; by the $30 ^{\circ } - 60^{\circ } -90^{\circ }$ Theorem, $m \angle D = 60 ^{\circ }$ . Again, by similiarity,

$m \angle A = m \angle D = 60 ^{\circ }$ .

Report an Error

Example Question #1 : How To Find The Length Of The Hypotenuse Of A Right Triangle : Pythagorean Theorem

If a right triangle has a base of and a height of , what is the length of the hypotenuse?

Possible Answers:

$\sqrt{14}$

$\sqrt{11}$

$\sqrt{65}$

Correct answer:

$\sqrt{65}$

Explanation:

To solve this problem, we must utilize the Pythagorean Theorom, which states that:

$a^{2}+b^{2}=c^{2}$

We know that the base is , so we can substitute in for . We also know that the height is , so we can substitute in for .

$7^{2}+4^{2}=c^{2}$

Next we evaluate the exponents:

$7^{2}=49$

$4^{2}=16$

Now we add them together:

49+16=65

Then, $65=c^{2}$ .

is not a perfect square, so we simply write the square root as $\sqrt{65}$ .

$c=\sqrt{65}$

Report an Error

Example Question #51 : Geometry

If a right triangle has a base of and a height of , what is the length of the hypotenuse?

Possible Answers:

$\sqrt{90}$

144

Correct answer:

$\sqrt{90}$

Explanation:

To solve this problem, we are going to use the Pythagorean Theorom, which states that $a^{2}+b^{2}=c^{2}$ .

We know that this particular right triangle has a base of , which can be substituted for , and a height of , which can be substituted for . If we rewrite the theorom using these numbers, we get:

$3^{2}+9^{2}=c^{2}$

Next, we evaluate the expoenents:

$3^{2}=9$

$9^{2}=81$

$9+81=c^{2}$

9+81=90

Then, $90=c^{2}$ .

To solve for , we must find the square root of . Since this is not a perfect square, our answer is simply $c=\sqrt{90}$ .

Report an Error

View Tutors

Margaret
Certified Tutor

University of Central Florida, Bachelor of Science, Health Services Administration. Nova Southeastern University, Master of S...

View Tutors

Reuben
Certified Tutor

University of Alberta, Bachelor of Science, Mathematics.

View Tutors

Aaron
Certified Tutor

Portland State University, Bachelors, Economics. Portland State University, Masters, Environmental and Resource Management.

All ISEE Upper Level Math Resources

6 Diagnostic Tests 244 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

French Tutors in Miami, SSAT Tutors in Phoenix, Algebra Tutors in Denver, Spanish Tutors in San Diego, MCAT Tutors in New York City, English Tutors in Miami, Biology Tutors in Houston, Biology Tutors in Philadelphia, Computer Science Tutors in Miami, Reading Tutors in Los Angeles

Popular Courses & Classes

SSAT Courses & Classes in Boston, ISEE Courses & Classes in Philadelphia, ISEE Courses & Classes in Los Angeles, MCAT Courses & Classes in Philadelphia, SSAT Courses & Classes in San Francisco-Bay Area, GRE Courses & Classes in Houston, SAT Courses & Classes in Dallas Fort Worth, ACT Courses & Classes in Houston, Spanish Courses & Classes in Phoenix, SSAT Courses & Classes in Seattle

Popular Test Prep

ACT Test Prep in Houston, GRE Test Prep in Houston, MCAT Test Prep in New York City, SSAT Test Prep in Miami, SSAT Test Prep in Dallas Fort Worth, ISEE Test Prep in Philadelphia, SSAT Test Prep in Atlanta, GRE Test Prep in Seattle, SAT Test Prep in Chicago, GMAT Test Prep in Seattle

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

ISEE Upper Level Math : Right Triangles

Study concepts, example questions & explanations for ISEE Upper Level Math

All ISEE Upper Level Math Resources

Example Questions

Example Question #4 : Triangles

Example Question #44 : Geometry

Example Question #1 : How To Find The Length Of The Side Of A Right Triangle

Example Question #1 : Right Triangles

Example Question #51 : Geometry

Example Question #4 : Triangles

Example Question #52 : Geometry

Example Question #1 : How To Find If Right Triangles Are Similar

Example Question #1 : How To Find The Length Of The Hypotenuse Of A Right Triangle : Pythagorean Theorem

Example Question #51 : Geometry

All ISEE Upper Level Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: