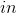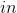ISEE Upper Level Quantitative : How to find the diagonal of a cube
Study concepts, example questions & explanations for ISEE Upper Level Quantitative
All ISEE Upper Level Quantitative Resources
Example Questions
Example Question #1 : How To Find The Diagonal Of A Cube
What is the length of the diagonal of a cube with a side length of 
It is easiest to think about diagonals like this by considering two points on a cube (if it were drawn in three dimensions). We could draw it like this:
(Note that not all points are drawn in on the cube.)
The two points we are looking at are:
Solve this by using the distance formula. This is very easy since one point is all 
This is approximately 
Example Question #2 : How To Find The Diagonal Of A Cube
What is the length of the diagonal of a cube with a side length of 
It is easiest to think about diagonals like this by considering two points on a cube (if it were drawn in three dimensions). We could draw it like this:
(Note that not all points are drawn in on the cube.)
The two points we are looking at are:
Solve this by using the distance formula. This is very easy since one point is all 
This is approximately 
Example Question #3 : How To Find The Diagonal Of A Cube
What is the length of the diagonal of a cube with a volume of 
First, you need to find the side length of this cube. We know that the volume is:
Therefore, based on our data, we can say:
Solving for 
Now, it is easiest to think about diagonals like this by considering two points on a cube (if it were drawn in three dimensions). We could draw it like this:
(Note that not all points are drawn in on the cube.)
The two points we are looking at are:
Solve this by using the distance formula. This is very easy since one point is all 
This is approximately 
Example Question #1 : How To Find The Diagonal Of A Cube
What is the length of the diagonal of a cube with a surface area of 
First, you need to find the side length of this cube. We know that the surface area is defined by:
Therefore, based on our data, we can say:
Take the square root of both sides and get:
Now, it is easiest to think about diagonals like this by considering two points on a cube (if it were drawn in three dimensions). We could draw it like this:
(Note that not all points are drawn in on the cube.)
The two points we are looking at are:
Solve this by using the distance formula. This is very easy since one point is all 
This is approximately 
Certified Tutor
Certified Tutor
All ISEE Upper Level Quantitative Resources
