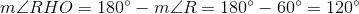Call Now to Set Up Tutoring:

(888) 888-0446

PSAT Math : How to find the length of the diagonal of a rhombus

Study concepts, example questions & explanations for PSAT Math

Create An Account

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #601 : Geometry

In Rhombus 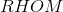RHOM $\displaystyle RHOM$ , $m \angle R = 60^{\circ }$ $\displaystyle m \angle R = 60^{\circ }$ . If $\overline{HM}$ $\displaystyle \overline{HM}$ is constructed, which of the following is true about $\Delta RHM$ $\displaystyle \Delta RHM$ ?

Possible Answers:

$\Delta RHM$ $\displaystyle \Delta RHM$ is obtuse and isosceles, but not equilateral

$\Delta RHM$ $\displaystyle \Delta RHM$ obtuse and scalene

$\Delta RHM$ $\displaystyle \Delta RHM$ is acute and isosceles, but not equilateral

$\Delta RHM$ $\displaystyle \Delta RHM$ is acute and scalene

$\Delta RHM$ $\displaystyle \Delta RHM$ is acute and equilateral

Correct answer:

$\Delta RHM$ $\displaystyle \Delta RHM$ is acute and equilateral

Explanation:

The figure referenced is below.

Rhombus

Consecutive angles of a rhombus are supplementary - as they are with all parallelograms - so

$m \angle RHO= 180^{\circ} - m \angle R = 180^{\circ} - 60^{\circ} = 120^{\circ}$ $\displaystyle m \angle RHO= 180^{\circ} - m \angle R = 180^{\circ} - 60^{\circ} = 120^{\circ}$

A diagonal of a rhombus bisects its angles, so

$m \angle RHM = \frac{1}{2} m \angle RHO= \frac{1}{2} \cdot 120^{\circ} = 60^{\circ}$ $\displaystyle m \angle RHM = \frac{1}{2} m \angle RHO= \frac{1}{2} \cdot 120^{\circ} = 60^{\circ}$

A similar argument proves that $m \angle RMH = 60^{\circ}$ $\displaystyle m \angle RMH = 60^{\circ}$ .

Since all three angles of $\Delta RHM$ $\displaystyle \Delta RHM$ measure $60^{\circ}$ $\displaystyle 60^{\circ}$ , the triangle is acute. It is also equiangular, and, subsequently, equilateral.

Report an Error

View PSAT Mathematics Tutors

Wardell
Certified Tutor

SIU Edwardsville, Bachelor in Arts, English.

View PSAT Mathematics Tutors

Westley
Certified Tutor

University of Nebraska-Lincoln, Bachelor in Arts, Mathematics.

View PSAT Mathematics Tutors

Sarah
Certified Tutor

Broward College, Bachelor of Science, Business Administration and Management.

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Statistics Tutors in San Francisco-Bay Area, Algebra Tutors in Boston, Biology Tutors in Chicago, MCAT Tutors in Miami, Math Tutors in Miami, GRE Tutors in Phoenix, Reading Tutors in San Diego, LSAT Tutors in Houston, Statistics Tutors in Seattle, SAT Tutors in Denver

Popular Courses & Classes

GMAT Courses & Classes in Chicago, ISEE Courses & Classes in Philadelphia, LSAT Courses & Classes in Chicago, Spanish Courses & Classes in Philadelphia, SSAT Courses & Classes in Phoenix, ACT Courses & Classes in Chicago, SAT Courses & Classes in Houston, ISEE Courses & Classes in Denver, GRE Courses & Classes in New York City, SSAT Courses & Classes in Boston

Popular Test Prep

SSAT Test Prep in Boston, GMAT Test Prep in Chicago, ACT Test Prep in Chicago, SAT Test Prep in Houston, GRE Test Prep in San Francisco-Bay Area, GRE Test Prep in Dallas Fort Worth, ISEE Test Prep in Philadelphia, MCAT Test Prep in San Francisco-Bay Area, ISEE Test Prep in Dallas Fort Worth, ACT Test Prep in San Diego

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

PSAT Math : How to find the length of the diagonal of a rhombus

Study concepts, example questions & explanations for PSAT Math

All PSAT Math Resources

Example Questions

Example Question #601 : Geometry

All PSAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: