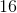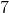Follow the correct order of operations: parenthenses, exponents, multiplication, division, addition, subtraction.

$\displaystyle \small (3+4)^2+(\frac{3+5}{2})+6\div 2$

First, evaluate any terms in parenthesis.

$\displaystyle (7)^2+(\frac{8}{2})+6\div 2$

$\displaystyle 7^2+4+6\div 2$

Next, evaluate the exponent.

$\displaystyle \small 49+4+6\div2$

Divide.

$\displaystyle \small 49+4+3$

Finally, add.

$\displaystyle \small 49+4+3=56$

Follow the correct order of operations: parentheses, exponents, multiplication, division, addition, subtraction. (This is typically abbreviated as PEMDAS. Note that both multiplication and division, and addition and subtraction, are equal to each other in terms of rank, so when both are present, solving the equation proceeds from left to right).

First, simplify anything in parentheses.

$\displaystyle \left(\frac{6}{6}\right)+8^2-4*6+5$

$\displaystyle \small 1+8^2-4*6+5$

Next, simplify any terms with exponents.

$\displaystyle \small 1+64-4*6+5$

Now, perform multiplication.

$\displaystyle \small 1+64-24+5$

Since all we are left with is addition and subtraction, we perform simplification from left to right.

$\displaystyle \small \small 65-24+5 = 41+5=46$

Thus, our answer is:

$\displaystyle \small \small 46$

In modulo 7 arithmetic, a number is congruent to the remainder of its division by 7. 

Therefore, since $\displaystyle 5 + 4 + 6 + 2 = 17$ and $\displaystyle 17 \div 7 = 2 \textrm{ R }3$,

$\displaystyle 5 + 4 + 6 + 2 \equiv 3 \mod 7$,

and the correct response is 3.

To solve $\displaystyle 100+1.01+0.01+0.00001$, make sure the digits are aligned with the correct placeholder.  It is also possible to add term by term.

$\displaystyle 100+1.01= 101.01$

$\displaystyle 101.01+0.01= 101.02$

$\displaystyle 101.02+ 0.00001=101.02001$

The correct answer is: $\displaystyle 101.02001$

Step 1: Recall PEMDAS...

Parentheses, Exponents, Multiplication, Division, Addition, Subtraction.

Step 2: Perform the evaluation in separate pieces...

$\displaystyle (2+1)^3=3^3=27$

$\displaystyle (3*4)=12$

$\displaystyle -7+3=-4$

$\displaystyle (24\div 6)=4$

Step 3: Replace the values and keep the signs..

$\displaystyle 27-12+4-4$

Step 4: Evaluate:

$\displaystyle (27-12)+(4-4)=15+0=15$

Add all the ones digits.

$\displaystyle 3+4+8+2+4 = 21$

Add the tens digits with the two as the carryover.

$\displaystyle 1+1+8+1+5+(2) = 18$

Combine this value with the ones digit of the first number.

The answer is:  $\displaystyle 181$

Add the ones digits.

$\displaystyle 4+9+9 = 22$

Add the tens digits with the tens digit of the previous number as carryover.

$\displaystyle 3+8+7+(2) = 20$

Repeat the process with the hundreds digits.

$\displaystyle 1+1+8+(2) = 12$

Combine this number with the ones digits of the previous calculations.

The answer is:  $\displaystyle 1202$

A set with $\displaystyle N$ elements has $\displaystyle 2 ^{N}$ subsets.

Solve:

$\displaystyle 2 ^{N} = 128$

$\displaystyle \ln 2 ^{N} = \ln 128$

$\displaystyle N \ln 2 = \ln 128$

$\displaystyle N = \frac{\ln 128}{\ln 2} = \frac{4.8520 }{0.6931} = 7$

Using the properties of logarithms

$\displaystyle n \ln a = \ln a^{n}$ and $\displaystyle \ln a - \ln b = \ln \frac{a}{b}$,

we simplify as follows:

$\displaystyle \ln x - 2 \ln (x + 2)$

$\displaystyle = \ln x - \ln (x + 2)^{2}$

$\displaystyle = \ln x - \ln (x^{2} +4x + 4)$

$\displaystyle = \ln \frac{x}{x^{2} +4x + 4}$

In order to solve this problem, covert 27 to the correct base and power.

$\displaystyle log_{3}27 = log_{3}\: 3^3$

Since $\displaystyle log_xx^n = n$, the correct answer is $\displaystyle 3$.