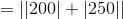Place values in the base two system are powers of two rather than powers of ten.

\(\displaystyle 1011101_{\textrm{two}}\) is equal to:

\(\displaystyle 1\times 2^{6}+0\times 2^{5}+ 1\times 2^{4}+1 \times 2^{3}+ 1 \times 2^{2}+ 0 \times 2^{1} +1\)

\(\displaystyle = 1\times 64+0\times32+ 1\times 16+1 \times 8+ 1 \times 4+ 0 \times2+1\)

\(\displaystyle = 64+0+ 16+ 8+ 4+ 0 +1 = 93\)

68 can be expressed as the sum of distinct powers of two as follows:

\(\displaystyle 68 = 64 + 4 = 2^{6}+ 2^{2}\)

Therefore, there are 1's in the seventh and third place from the right, and 0's everywhere else, so

\(\displaystyle 68 = 1000100_{\textrm{two}}\)

73 can be uniquely expressed as the sum of distinct powers of two as follows:

\(\displaystyle 73 = 64 + 8 + 1 = 2^{6} + 2^{3}+ 2^{0}\)

Therefore, there is a "1" in the seventh, fourth, and first positions from the right, and a "0" everywhere else. So,

\(\displaystyle 73 = 1001001_{\textrm{two}}\)

By definition, an imaginary number is a number with the term "i" in it. For this problem 7i is the only answer with the imaginary part.

Substitute \(\displaystyle a= 100, b = -50\) in the expression:

\(\displaystyle a \vee b =\left | \left | a - 2b \right | + \left | 2a - b\right | \right |\)

\(\displaystyle 100 \vee (-50) =\left | \left | 100 - 2(-50)\right | + \left | 2 (100 ) - (-50)\right | \right |\)

\(\displaystyle =\left | \left | 100 - (-100)\right | + \left | 200 +50\right | \right |\)

\(\displaystyle =\left | \left | 200\right | + \left | 2 50\right | \right |\)

\(\displaystyle =\left | 200 + 2 50 \right |\)

\(\displaystyle =\left | 4 50 \right |\)

\(\displaystyle = 4 50\)

From the last digit, it can be immediately determined that 873 is not a multple of 2 or 5; since \(\displaystyle 873 \div 3 = 291\), 873 is a multiple of 3. Therefore, 

\(\displaystyle 873 \in A' \cap B \cap C'\)

We are looking for an integer that is also in this set - that is, one that is also a multiple of 3 but not 2 or 5. From the last digits, we can immediately eliminate 366 and 368 as multiples of 2 and 365 as a multiple of 5. We test 367 and 369 to see which one is a multiple of 3:

\(\displaystyle 367 \div 3 = 122 \textrm{ R }1\)

\(\displaystyle 369 \div 3 = 123\)

369 is the correct choice.

A set is closed under multiplication if and only if the product of any two (not necessarily distinct) elements of that set is itself an element of that set. 

This can easily be disproved in the case of three of these sets:

\(\displaystyle \left \{ 2, 12, 22, 32, 42...\right \}\)

\(\displaystyle 2 \times 12 = 24 \notin \left \{ 2, 12, 22, 32, 42...\right \}\)

\(\displaystyle \left \{ 3, 13, 23, 33, 43...\right \}\)

\(\displaystyle 3 \times 13 = 39 \notin \left \{ 3, 13, 23, 33, 43...\right \}\)

\(\displaystyle \left \{ 4, 14, 24, 34, 44...\right \}\)

\(\displaystyle 4 \times 14 = 96 \notin \left \{ 4, 14, 24, 34, 44...\right \}\)

But closure can be proved to hold in the case of \(\displaystyle \left \{ 1, 11, 21, 31, 41,...\right \}\). Each number takes the form of \(\displaystyle 10N+1\) for some nonnegative integer \(\displaystyle N\). If we multiply two numbers in this form, we get

\(\displaystyle \left (10N+1 \right )\left (10M+1 \right )\)

\(\displaystyle = 100MN + 10N + 10M + 1\)

\(\displaystyle = 10 (10MN+M+N)+1\)

which is an element of \(\displaystyle \left \{ 1, 11, 21, 31, 41,...\right \}\), being of this form.

The power set is the set of all subsets that can be created from the original set.

For the set  \(\displaystyle X=\left \{a,b,c}{ \right \}\), you can create the subsets:

\(\displaystyle \left \{ a\right \}\left \{ b\right \}\left \{ c\right \}\left \{a,b \right \}\left \{ a,c\right \}\left \{b,c \right \}\left \{ a,b,c\right \}\left \{ \varnothing \right \}\)

This means that the power set is the set of all sets, so the power set is:

\(\displaystyle P=\left \{ \left \{ a\right \}\left \{ b\right \}\left \{ c\right \}\left \{a,b \right \}\left \{ a,c\right \}\left \{b,c \right \}\left \{ a,b,c\right \}\left \{ \varnothing \right \} \right \}\)

This is the product of a complex number and its complex conjugate. They can be multiplied using the pattern

\(\displaystyle \left (A + Bi \right )\left (A - Bi \right ) = A^{2} + B^{2}\)

with \(\displaystyle A = 4, B = 7\)

\(\displaystyle \left (4 + 7i \right )\left (4 - 7i \right ) = 4^{2} + 7^{2} = 16 + 49 = 65\)

This is not among the given responses.

\(\displaystyle -3 i \cdot 8i \cdot 5i \cdot 6i\)

\(\displaystyle = \left (-3 \cdot 8 \cdot 5 \cdot 6 \right ) \cdot\left ( i \cdot i \cdot i \cdot i \right )\)

\(\displaystyle = \left (-720 \right ) \cdot\left ( i ^{4}\right )\)

\(\displaystyle -720 \cdot 1 = -720\)