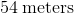We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

SAT Math : How to find the perimeter of a rectangle

Study concepts, example questions & explanations for SAT Math

Create An Account

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #201 : Geometry

A rectangle has a width of 2x. If the length is five more than 150% of the width, what is the perimeter of the rectangle?

Possible Answers:

6x² + 10x

5x + 10

5x + 5

10(x + 1)

6x² + 5

Correct answer:

10(x + 1)

Explanation:

Given that w = 2x and l = 1.5w + 5, a substitution will show that l = 1.5(2x) + 5 = 3x + 5.

P = 2w + 2l = 2(2x) + 2(3x + 5) = 4x + 6x + 10 = 10x + 10 = 10(x + 1)

Report an Error

Example Question #1 : How To Find The Perimeter Of A Rectangle

Find the perimeter of a rectangle with width 7 and length 9.

Possible Answers:

$\frac{63}{2}$

Correct answer:

Explanation:

To solve, simply use the formula for the perimeter of a rectangle.

Substitute in the width of seven and the length of nine.

Thus,

P=2(w+l)=2*(7+9)=2*16=32

Report an Error

Example Question #31 : Quadrilaterals

Find the perimeter of a rectangle whose side lengths are 1 and 2.

Possible Answers:

Correct answer:

Explanation:

To solve, simply use the formula for the perimeter of a rectangle. Thus,

P=2(w+l)=2*(1+2)=2*3=6

Report an Error

Example Question #2 : How To Find The Perimeter Of A Rectangle

Find the perimeter of a rectangle with width 6 and length 9.

Possible Answers:

Correct answer:

Explanation:

To solve, simply use the formula for the perimeter.

P=2(w+l)

P=2*(6+9)=2*15=30

Another way to solve this problem is to add up all of the sides. Remember that even though only two values are given, a rectangle has 4 sides. Thus,

P=6+6+9+9=30

Report an Error

Example Question #31 : Rectangles

You have a poster of one of your favorite bands that you are planning on putting up in your dorm room. If the poster is 3 feet tall by 1.5 feet wide, what is the perimeter of the poster?

Possible Answers:

9ft

12ft

9.5ft

4.5ft^2

Correct answer:

9ft

Explanation:

You have a poster of one of your favorite bands that you are planning on putting up in your dorm room. If the poster is 3 feet tall by 1.5 feet wide, what is the perimeter of the poster?

Perimeter of a rectangle is found via:

P=2l+2w=2(3ft)+2(1.5ft)=6ft+3ft=9ft

Report an Error

Example Question #3 : How To Find The Perimeter Of A Rectangle

Three of the vertices of a rectangle on the coordinate plane are located at the origin, (10, 0) , and (0, 7) . Give the perimeter of the rectangle.

Possible Answers:

Correct answer:

Explanation:

The rectangle in question is below:

Rectangle 3

The lengths of the rectangle is 10, the distance from the origin to (10,0) ; its width is 7, the distance from the origin to (0, 7) . The perimeter of a rectangle is equal to twice the sum of its length and width, so calculate:

$P= 2 (10+ 7 ) = 2 \times 17 = 34$ .

Report an Error

Example Question #3 : How To Find The Perimeter Of A Figure

A rectangular garden has an area of $80\: \mbox{m^2}$ . Its length is meters longer than its width. How much fencing is needed to enclose the garden?

Possible Answers:

$36\: \mbox{meters}$

$18\: \mbox{meters}$

$54\: \mbox{meters}$

$24\: \mbox{meters}$

$40\: \mbox{meters}$

Correct answer:

$36\: \mbox{meters}$

Explanation:

We define the variables as $w = \mbox{width}$ and $l = \mbox{length} = w + 2$ .

We substitute these values into the equation for the area of a rectangle and get $A_{\mbox{rectangle} }= wl = w(w + 2) = 80$ .

w^2 +2w-80=0

(w - 8)(w + 10) = 0

w = 8 or w = -10

Lengths cannot be negative, so the only correct answer is w = 8 . If w = 8 , then l = 10 .

Therefore, $\mbox{perimeter }= 2w + 2l = 16 + 20 = 36\:\mbox{m}$ .

Report an Error

View SAT Mathematics Tutors

Nicholas
Certified Tutor

Fordham University, Bachelors, Business Administration and Management. Boston University, Masters, Media Ventures.

View SAT Mathematics Tutors

Scott
Certified Tutor

Rutgers College, Bachelors, Political Science and Government. Florida Coastal School of Law, PHD, Law.

View SAT Mathematics Tutors

Noah
Certified Tutor

Bard College, Bachelors, Philosophy and Creative Writing (Dual Major). Sarah Lawrence College, Master of Fine Arts, Creative ...

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

SAT Math Tutors in Top Cities:

Atlanta SAT Math Tutors, Austin SAT Math Tutors, Boston SAT Math Tutors, Chicago SAT Math Tutors, Dallas Fort Worth SAT Math Tutors, Denver SAT Math Tutors, Houston SAT Math Tutors, Kansas City SAT Math Tutors, Los Angeles SAT Math Tutors, Miami SAT Math Tutors, New York City SAT Math Tutors, Philadelphia SAT Math Tutors, Phoenix SAT Math Tutors, San Diego SAT Math Tutors, San Francisco-Bay Area SAT Math Tutors, Seattle SAT Math Tutors, St. Louis SAT Math Tutors, Tucson SAT Math Tutors, Washington DC SAT Math Tutors

Popular Courses & Classes

GMAT Courses & Classes in Phoenix, ISEE Courses & Classes in Miami, GRE Courses & Classes in Boston, MCAT Courses & Classes in Denver, ACT Courses & Classes in San Diego, SSAT Courses & Classes in San Diego, Spanish Courses & Classes in San Francisco-Bay Area, SSAT Courses & Classes in Philadelphia, MCAT Courses & Classes in Atlanta, SSAT Courses & Classes in Seattle

Popular Test Prep

SAT Test Prep in Dallas Fort Worth, MCAT Test Prep in Chicago, GRE Test Prep in Denver, SSAT Test Prep in Dallas Fort Worth, ACT Test Prep in New York City, SSAT Test Prep in Washington DC, ACT Test Prep in Houston, ISEE Test Prep in Denver, SAT Test Prep in Washington DC, SAT Test Prep in Los Angeles

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SAT Math : How to find the perimeter of a rectangle

Study concepts, example questions & explanations for SAT Math

All SAT Math Resources

Example Questions

Example Question #201 : Geometry

Example Question #1 : How To Find The Perimeter Of A Rectangle

Example Question #31 : Quadrilaterals

Example Question #2 : How To Find The Perimeter Of A Rectangle

Example Question #31 : Rectangles

Example Question #3 : How To Find The Perimeter Of A Rectangle

Example Question #3 : How To Find The Perimeter Of A Figure

All SAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: